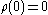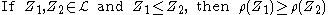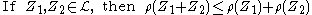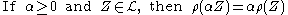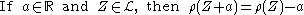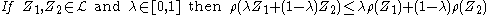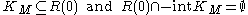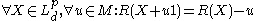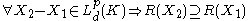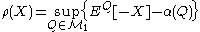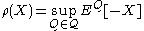Coherent risk measure
Encyclopedia
In the field of financial economics
there are a number of ways that risk can be defined; to clarify the concept theoreticians have described a number of properties that a risk measure
might or might not have. A coherent risk measure is a function ρ that satisfies properties of monotonicity, sub-additivity, homogeneity
, and translational invariance.
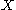 viewed as an element of a linear space
viewed as an element of a linear space 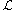 of measurable functions, defined on an appropriate probability space. A functional
of measurable functions, defined on an appropriate probability space. A functional
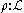 →
→ 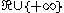 is said to be coherent risk measure for
is said to be coherent risk measure for  if it satisfies the following properties:
if it satisfies the following properties:
Normalized
That is, the risk of holding no assets is zero.
Monotonicity
That is, if portfolio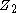 always has better values than portfolio
always has better values than portfolio 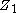 under all scenarios then the risk of
under all scenarios then the risk of  should be less than the risk of
should be less than the risk of 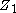 .
.
Sub-additivity
Indeed, the risk of two portfolios together cannot get any worse than adding the two risks separately: this is the diversification
principle.
Positive homogeneity
Loosely speaking, if you double your portfolio then you double your risk.
Translation invariance
The value is just adding cash to your portfolio
is just adding cash to your portfolio  , which acts like an insurance: the risk of
, which acts like an insurance: the risk of  is less than the risk of
is less than the risk of 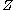 , and the difference is exactly the added cash
, and the difference is exactly the added cash  . In particular, if
. In particular, if 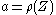 then
then 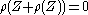 .
.
Convexity
is not, in general, a coherent risk measure as it does not respect the sub-additivity property. An immediate consequence is that value at risk
might discourage diversification.
Value at risk
is, however, coherent, under the assumption of elliptically distributed
losses (e.g. normally distributed) when the portfolio value is a linear function of the asset prices. However, in this case the value at risk becomes equivalent to a mean-variance approach where the risk of a portfolio is measured by the variance of the portfolio's return.
Assume the following:
Under these conditions the 95% VaR for holding either of the bonds is 0% since the probability of default is less than 5%. However if we held a portfolio that consisted of 50% of each bond by value then the 95% VaR is 35% since the probability of at least one of the bonds defaulting is 7.84% which exceeds 5%. This violates the sub-additivity property showing that VaR is not a coherent risk measure.
or conditional value-at-risk) is a coherent risk measure, even though it is derived from Value at Risk which is not.
is a convex risk measure which is not coherent. It is related to the exponential utility
.
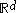 -valued portfolios such that risk can be measured in
-valued portfolios such that risk can be measured in  of the assets, then a set of portfolios is the proper way to depict risk. Set-valued risk measures are useful for markets with transaction cost
of the assets, then a set of portfolios is the proper way to depict risk. Set-valued risk measures are useful for markets with transaction cost
s.
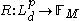 , where
, where 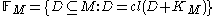 and
and 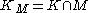 where
where  is a constant solvency cone
is a constant solvency cone
and is the set of portfolios of the
is the set of portfolios of the 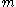 reference assets.
reference assets. 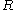 must have the following properties:
must have the following properties:
Normalized
Translative in M
Monotone
Sublinear
is convex (coherent) if and only if the corresponding risk measure is convex (coherent). As defined below it can be shown that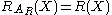 and
and 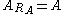 .
.
 can be represented as
can be represented as
such that is a penalty function.
is a penalty function.
A risk measure is coherent if and only if it can be represented as
such that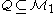 .
.
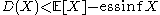 for every X (where
for every X (where 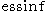 is the essential infimum) is a deviation risk measure
is the essential infimum) is a deviation risk measure
, then there is a one-to-one relationship between D and an expectation-bounded coherent risk measure where for any
where for any 
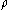 is expectation bounded if
is expectation bounded if 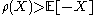 for any nonconstant X and
for any nonconstant X and 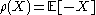 for any constant X.
for any constant X.
Financial economics
Financial Economics is the branch of economics concerned with "the allocation and deployment of economic resources, both spatially and across time, in an uncertain environment"....
there are a number of ways that risk can be defined; to clarify the concept theoreticians have described a number of properties that a risk measure
Risk measure
A Risk measure is used to determine the amount of an asset or set of assets to be kept in reserve. The purpose of this reserve is to make the risks taken by financial institutions, such as banks and insurance companies, acceptable to the regulator...
might or might not have. A coherent risk measure is a function ρ that satisfies properties of monotonicity, sub-additivity, homogeneity
Homogeneity (statistics)
In statistics, homogeneity and its opposite, heterogeneity, arise in describing the properties of a dataset, or several datasets. They relate to the validity of the often convenient assumption that the statistical properties of any one part of an overall dataset are the same as any other part...
, and translational invariance.
Properties
Consider a random outcome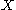 viewed as an element of a linear space
viewed as an element of a linear space 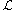 of measurable functions, defined on an appropriate probability space. A functional
of measurable functions, defined on an appropriate probability space. A functionalFunctional (mathematics)
In mathematics, and particularly in functional analysis, a functional is a map from a vector space into its underlying scalar field. In other words, it is a function that takes a vector as its input argument, and returns a scalar...
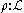 →
→ 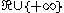 is said to be coherent risk measure for
is said to be coherent risk measure for  if it satisfies the following properties:
if it satisfies the following properties:Normalized
That is, the risk of holding no assets is zero.
Monotonicity
That is, if portfolio
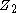 always has better values than portfolio
always has better values than portfolio 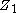 under all scenarios then the risk of
under all scenarios then the risk of  should be less than the risk of
should be less than the risk of 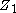 .
.Sub-additivity
Indeed, the risk of two portfolios together cannot get any worse than adding the two risks separately: this is the diversification
Diversification
Diversification may refer to:* Diversification involves spreading investments* Diversification is a corporate strategy to increase market penetration...
principle.
Positive homogeneity
Loosely speaking, if you double your portfolio then you double your risk.
Translation invariance
The value
 is just adding cash to your portfolio
is just adding cash to your portfolio  , which acts like an insurance: the risk of
, which acts like an insurance: the risk of  is less than the risk of
is less than the risk of 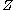 , and the difference is exactly the added cash
, and the difference is exactly the added cash  . In particular, if
. In particular, if 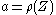 then
then 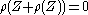 .
.Convex risk measures
The notion of coherence has been subsequently relaxed. Indeed, the notions of Sub-additivity and Positive Homogeneity can be replaced by the notion of convexity:Convexity
Value at risk
It is well known that value at riskValue at risk
In financial mathematics and financial risk management, Value at Risk is a widely used risk measure of the risk of loss on a specific portfolio of financial assets...
is not, in general, a coherent risk measure as it does not respect the sub-additivity property. An immediate consequence is that value at risk
Value at risk
In financial mathematics and financial risk management, Value at Risk is a widely used risk measure of the risk of loss on a specific portfolio of financial assets...
might discourage diversification.
Value at risk
Value at risk
In financial mathematics and financial risk management, Value at Risk is a widely used risk measure of the risk of loss on a specific portfolio of financial assets...
is, however, coherent, under the assumption of elliptically distributed
Elliptical distribution
In probability and statistics, an elliptical distribution is any member of a broad family of probability distributions that generalize the multivariate normal distribution and inherit some of its properties.-Definition:...
losses (e.g. normally distributed) when the portfolio value is a linear function of the asset prices. However, in this case the value at risk becomes equivalent to a mean-variance approach where the risk of a portfolio is measured by the variance of the portfolio's return.
Illustration
As a simple example to demonstrate the non-coherence of value-at-risk consider looking at the VaR of a portfolio at 95% confidence over the next year of two default-able zero coupon bonds that mature in 1 years time denominated in our numeraire currency.Assume the following:
- The current yield on the two bonds is 0%
- The two bonds are from different issuers
- Each bonds has a 4% probability of defaulting over the next year
- The event of default in either bond is independent of the other
- Upon default the bonds have a recovery rate of 30%
Under these conditions the 95% VaR for holding either of the bonds is 0% since the probability of default is less than 5%. However if we held a portfolio that consisted of 50% of each bond by value then the 95% VaR is 35% since the probability of at least one of the bonds defaulting is 7.84% which exceeds 5%. This violates the sub-additivity property showing that VaR is not a coherent risk measure.
Average value at risk
The average value at risk (sometimes called expected shortfallExpected shortfall
Expected shortfall is a risk measure, a concept used in finance to evaluate the market risk or credit risk of a portfolio. It is an alternative to value at risk that is more sensitive to the shape of the loss distribution in the tail of the distribution...
or conditional value-at-risk) is a coherent risk measure, even though it is derived from Value at Risk which is not.
Tail value at risk
The tail value at risk (or tail conditional expectation) is a coherent risk measure only when the underlying distribution is continuous.Entropic risk measure
The entropic risk measureEntropic risk measure
In financial mathematics, the entropic risk measure is a risk measure which depends on the risk aversion of the user through the exponential utility function. This makes it a theoretically interesting measure because it would provide different risk values for different individuals...
is a convex risk measure which is not coherent. It is related to the exponential utility
Exponential utility
In economics exponential discounting is a specific form of the discount function, used in the analysis of choice over time...
.
Set-valued
In a situation with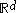 -valued portfolios such that risk can be measured in
-valued portfolios such that risk can be measured in  of the assets, then a set of portfolios is the proper way to depict risk. Set-valued risk measures are useful for markets with transaction cost
of the assets, then a set of portfolios is the proper way to depict risk. Set-valued risk measures are useful for markets with transaction costTransaction cost
In economics and related disciplines, a transaction cost is a cost incurred in making an economic exchange . For example, most people, when buying or selling a stock, must pay a commission to their broker; that commission is a transaction cost of doing the stock deal...
s.
Properties
A set-valued coherent risk measure is a function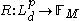 , where
, where 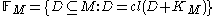 and
and 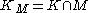 where
where  is a constant solvency cone
is a constant solvency coneSolvency cone
The solvency cone is a concept used in financial mathematics which models the possible trades in the financial market. This is of particular interest to markets with transaction costs...
and
 is the set of portfolios of the
is the set of portfolios of the 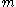 reference assets.
reference assets. 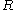 must have the following properties:
must have the following properties:Normalized
Translative in M
Monotone
Sublinear
Set-valued convex risk measure
If instead of the sublinear property,R is convex, then R is a set-valued convex risk measure.Relation to Acceptance Sets
An acceptance setAcceptance set
In financial mathematics, acceptance sets are a mathematical concept related to risk measures. As the name suggests an acceptance set is a set of acceptable future net worth, which are acceptable to the regulator.-Mathematical Definition:...
is convex (coherent) if and only if the corresponding risk measure is convex (coherent). As defined below it can be shown that
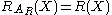 and
and 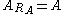 .
.Risk Measure to Acceptance Set
- If
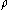 is a (scalar) risk measure then
is a (scalar) risk measure then 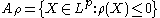 is an acceptance set.
is an acceptance set. - If
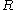 is a set-valued risk measure then
is a set-valued risk measure then 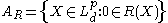 is an acceptance set.
is an acceptance set.
Acceptance Set to Risk Measure
- If
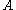 is an acceptance set (in 1-d) then
is an acceptance set (in 1-d) then 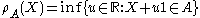 defines a (scalar) risk measure.
defines a (scalar) risk measure. - If
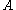 is an acceptance set then
is an acceptance set then 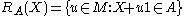 is a set-valued risk measure.
is a set-valued risk measure.
Dual representation
A convex risk measure can be represented as
can be represented as
such that
 is a penalty function.
is a penalty function.A risk measure is coherent if and only if it can be represented as
such that
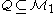 .
.Relation to deviation risk measure
If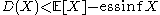 for every X (where
for every X (where 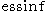 is the essential infimum) is a deviation risk measure
is the essential infimum) is a deviation risk measureDeviation risk measure
In financial mathematics, a deviation risk measure is a function to quantify financial risk in a different method than a general risk measure...
, then there is a one-to-one relationship between D and an expectation-bounded coherent risk measure
 where for any
where for any 
-
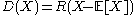
-
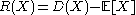 .
.
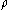 is expectation bounded if
is expectation bounded if 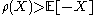 for any nonconstant X and
for any nonconstant X and 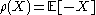 for any constant X.
for any constant X.External links
- A list of important papers on coherent and convex risk measures
- Glyn Holton: The Case for Incoherence Controversial article that argues coherence is not a desirable property. "There are two types of risk metrics – coherent and incoherent. In the vast majority of cases, you want one that is incoherent."