
100-year flood
Encyclopedia
A one-hundred-year flood is calculated to be the level of flood
water expected to be equaled or exceeded every 100 years on average. The 100-year flood is more accurately referred to as the 1% annual exceedance probability flood, since it is a flood that has a 1% chance of being equaled or exceeded in any single year. Similarly, a flood level expected to be equaled or exceeded every 10 years on average is known as a ten-year flood. Based on the expected flood water
level, a predicted area of inundation can be mapped out. This floodplain
map figures very importantly in building permits, environmental regulations, and flood insurance
.
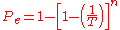
where T is the return period of a given storm threshold (e.g. 100-yr, 50-yr, 25-yr, and so forth), and n is the number of years. The exceedance probability Pe is also described as the natural, inherent, or hydrologic risk of failure. However, the expected value
of the number of 100-year floods occurring in any 100-year period is 1.
Ten-year floods have a 10% chance of occurring in any given year (Pe =0.10); 500-year have a 0.2% chance of occurring in any given year (Pe =0.002); etc. The percent chance of an X-year flood occurring in a single year can be calculated by dividing 100 by X.
The field of extreme value theory
was created to model rare events such as 100-year floods for the purposes of civil engineering. This theory is most commonly applied to the maximum or minimum observed stream flows of a given river. In desert areas where there are only ephemeral washes, this method is applied to the maximum observed rainfall over a given period of time (24-hours, 6-hours, or 3-hours). The extreme value analysis only considers the most extreme event observed in a given year. So, between the large spring runoff and a heavy summer rain storm, whichever resulted in more runoff would be considered the extreme event, while the smaller event would be ignored in the analysis (even though both may have been capable of causing terrible flooding in their own right).
from year-to-year. In other words the maximum river flow rate from 1984, can not be found to be significantly
correlated with the observed flow rate in 1985. 1985 can not be correlated with 1986, and so forth. The second assumption is that the observed extreme events must come from the same probability distribution function
. The third assumption is that the probability distribution relates to the largest storm (rainfall or river flow rate measurement) that occurs in any one year. The fourth assumption is that the probability distribution function is stationary, meaning that the mean
(average), standard deviation
and max/min values are not increasing or decreasing over time. This concept is referred to as stationarity
.
The first assumption has a very low chance of being valid in all places. Studies have shown that extreme events in certain watersheds in the U.S. are not significantly correlated, but this must be determined on a case by case basis. The second assumption is often valid if the extreme events are observed under similar climate conditions. For example, if the extreme events on record all come from late summer thunder storms (as is the case in the southwest U.S.), or from snow pack melting (as is the case in north-central U.S.), then this assumption should be valid. If, however, there are some extreme events taken from thunder storms, others from snow pack melting, and others from hurricanes, then this assumption is most likely not valid. The third assumption is only a problem if you are trying to forecast a low, but maximum flow event (say, you are tying to find the max event for the 1-year storm event). Since this is not typically a goal in extreme analysis, or in civil engineering design, then the situation rarely presents itself. The final assumption about stationarity has come into question in light of the research being done on climate change
. In short, the argument being made is that if temperatures are changing and precipitation cycles are being altered, then there is compelling evidence that the probability distribution is also changing. The simplest implication of this is that not all of the historical data are, or can be, considered valid as input into the extreme event analysis.
Most precipitation records are based on a measured depth of water received within a fixed time interval. Frequency of a precipitation threshold of interest may be determined from the number of measurements exceeding that threshold value within the total time period for which observations are available. Individual data points are converted to intensity by dividing each measured depth by the period of time between observations. This intensity will be less than the actual peak intensity if the duration of the rainfall event was less than the fixed time interval for which measurements are reported. Convective precipitation events (thunderstorms) tend to produce shorter duration storm events than orographic precipitation. Duration, intensity, and frequency of rainfall events are important to flood prediction. Short duration precipitation is more significant to flooding within small drainage basins.
The most important upslope factor in determining flood magnitude is the land area of the watershed upstream of the area of interest. Rainfall intensity is the second most important factor for watersheds of less than approximately 30 square miles or 77.7 km². The main channel slope is the second most important factor for larger watersheds. Channel slope and rainfall intensity become the third most important factors for small and large watersheds, respectively.
or a natural or artificial lake
. Elevation changes such as tidal fluctuations are significant determinants of coastal and estuarine flooding. Less predictable events like tsunami
s and storm surge
s may also cause elevation changes in large bodies of water. Elevation of flowing water is controlled by the geometry of the flow channel. Flow channel restrictions like bridges and canyons tend to control water elevation above the restriction. The actual control point for any given reach of the drainage may change with changing water elevation, so a closer point may control for lower water levels until a more distant point controls at higher water levels.
Effective flood channel geometry may be changed by growth of vegetation, accumulation of ice or debris, or construction of bridges, buildings, or levees within the flood channel.
is the time required for runoff from the most distant point of the upstream drainage area to reach the point of the drainage channel controlling flooding of the area of interest. The time of concentration defines the critical duration of peak rainfall for the area of interest. The critical duration of intense rainfall might be only a few minutes for roof and parking lot drainage structures, while cumulative rainfall over several days would be critical for river basins.
Extreme flood events often result from coincidence such as unusually intense, warm rainfall melting heavy snow pack, producing channel obstructions from floating ice, and releasing small impoundments like beaver
dams. Coincident events may cause flooding outside the statistical distribution anticipated by simplistic prediction models. Debris modification of channel geometry is common when heavy flows move uprooted woody vegetation and flood-damaged structures and vehicles, including boats and railway equipment.
Flood
A flood is an overflow of an expanse of water that submerges land. The EU Floods directive defines a flood as a temporary covering by water of land not normally covered by water...
water expected to be equaled or exceeded every 100 years on average. The 100-year flood is more accurately referred to as the 1% annual exceedance probability flood, since it is a flood that has a 1% chance of being equaled or exceeded in any single year. Similarly, a flood level expected to be equaled or exceeded every 10 years on average is known as a ten-year flood. Based on the expected flood water
Water
Water is a chemical substance with the chemical formula H2O. A water molecule contains one oxygen and two hydrogen atoms connected by covalent bonds. Water is a liquid at ambient conditions, but it often co-exists on Earth with its solid state, ice, and gaseous state . Water also exists in a...
level, a predicted area of inundation can be mapped out. This floodplain
Floodplain
A floodplain, or flood plain, is a flat or nearly flat land adjacent a stream or river that stretches from the banks of its channel to the base of the enclosing valley walls and experiences flooding during periods of high discharge...
map figures very importantly in building permits, environmental regulations, and flood insurance
Flood insurance
Flood insurance denotes the specific insurance coverage against property loss from flooding. To determine risk factors for specific properties, insurers will often refer to topographical maps that denote lowlands, floodplains and floodways that are susceptible to flooding.-Hidden floods:Nationwide,...
.
Probability
There is approximately a 63.4% chance of one or more 100-year floods occurring in any 100-year period, not a 100 percent chance. The probability Pe that one or more of a certain-size flood occurring during any period will exceed the 100-yr flood threshold can be expressed as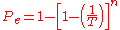
where T is the return period of a given storm threshold (e.g. 100-yr, 50-yr, 25-yr, and so forth), and n is the number of years. The exceedance probability Pe is also described as the natural, inherent, or hydrologic risk of failure. However, the expected value
Expected value
In probability theory, the expected value of a random variable is the weighted average of all possible values that this random variable can take on...
of the number of 100-year floods occurring in any 100-year period is 1.
Ten-year floods have a 10% chance of occurring in any given year (Pe =0.10); 500-year have a 0.2% chance of occurring in any given year (Pe =0.002); etc. The percent chance of an X-year flood occurring in a single year can be calculated by dividing 100 by X.
The field of extreme value theory
Extreme value theory
Extreme value theory is a branch of statistics dealing with the extreme deviations from the median of probability distributions. The general theory sets out to assess the type of probability distributions generated by processes...
was created to model rare events such as 100-year floods for the purposes of civil engineering. This theory is most commonly applied to the maximum or minimum observed stream flows of a given river. In desert areas where there are only ephemeral washes, this method is applied to the maximum observed rainfall over a given period of time (24-hours, 6-hours, or 3-hours). The extreme value analysis only considers the most extreme event observed in a given year. So, between the large spring runoff and a heavy summer rain storm, whichever resulted in more runoff would be considered the extreme event, while the smaller event would be ignored in the analysis (even though both may have been capable of causing terrible flooding in their own right).
Statistical assumptions
There are a number of assumptions which are made to complete the analysis which determines the 100-year flood. First, the extreme events observed in each year, must be independentStatistical independence
In probability theory, to say that two events are independent intuitively means that the occurrence of one event makes it neither more nor less probable that the other occurs...
from year-to-year. In other words the maximum river flow rate from 1984, can not be found to be significantly
Statistical significance
In statistics, a result is called statistically significant if it is unlikely to have occurred by chance. The phrase test of significance was coined by Ronald Fisher....
correlated with the observed flow rate in 1985. 1985 can not be correlated with 1986, and so forth. The second assumption is that the observed extreme events must come from the same probability distribution function
Probability distribution function
Depending upon which text is consulted, a probability distribution function is any of:* a probability distribution function,* a cumulative distribution function,* a probability mass function, or* a probability density function....
. The third assumption is that the probability distribution relates to the largest storm (rainfall or river flow rate measurement) that occurs in any one year. The fourth assumption is that the probability distribution function is stationary, meaning that the mean
Mean
In statistics, mean has two related meanings:* the arithmetic mean .* the expected value of a random variable, which is also called the population mean....
(average), standard deviation
Standard deviation
Standard deviation is a widely used measure of variability or diversity used in statistics and probability theory. It shows how much variation or "dispersion" there is from the average...
and max/min values are not increasing or decreasing over time. This concept is referred to as stationarity
Stationary process
In the mathematical sciences, a stationary process is a stochastic process whose joint probability distribution does not change when shifted in time or space...
.
The first assumption has a very low chance of being valid in all places. Studies have shown that extreme events in certain watersheds in the U.S. are not significantly correlated, but this must be determined on a case by case basis. The second assumption is often valid if the extreme events are observed under similar climate conditions. For example, if the extreme events on record all come from late summer thunder storms (as is the case in the southwest U.S.), or from snow pack melting (as is the case in north-central U.S.), then this assumption should be valid. If, however, there are some extreme events taken from thunder storms, others from snow pack melting, and others from hurricanes, then this assumption is most likely not valid. The third assumption is only a problem if you are trying to forecast a low, but maximum flow event (say, you are tying to find the max event for the 1-year storm event). Since this is not typically a goal in extreme analysis, or in civil engineering design, then the situation rarely presents itself. The final assumption about stationarity has come into question in light of the research being done on climate change
Climate change
Climate change is a significant and lasting change in the statistical distribution of weather patterns over periods ranging from decades to millions of years. It may be a change in average weather conditions or the distribution of events around that average...
. In short, the argument being made is that if temperatures are changing and precipitation cycles are being altered, then there is compelling evidence that the probability distribution is also changing. The simplest implication of this is that not all of the historical data are, or can be, considered valid as input into the extreme event analysis.
Probability uncertainty
When these assumptions are violated there is an unknown amount of uncertainty introduced into the reported value of what the 100-year flood means in terms of rainfall intensity, or river flood depth. When all of the inputs are known the uncertainty can be measured in the form of a confidence interval. For example, one might say there is a 95% chance that the 100-year flood is greater than X, but less than Y. Without analyzing the statistical uncertainty of a given 100-year flood, scientists and engineers can decrease the uncertainty by using two practical rules. First, forecast an extreme event which is no more than double your observation years (e.g. you have 27 observed river measurements, so you can determine a 50-year event since 27×2=54, but not a 100-yr event). The second way to decrease the uncertainty of the extreme event is to forecast a value which is less than the maximum observed value (e.g. the maximum rainfall event on record is 5.25 inches/hour, so the 100-year storm event should be less than this).Upslope factors
The amount, location, and timing of water reaching a drainage channel from natural precipitation and controlled or uncontrolled reservoir releases determines the flow at downstream locations. Some precipitation evaporates, some slowly percolates through soil, some may be temporarily sequestered as snow or ice, and some may produce rapid runoff from surfaces including rock, pavement, roofs, and saturated or frozen ground. The fraction of incident precipitation promptly reaching a drainage channel has been observed from nil for light rain on dry, level ground to as high as 170 percent for warm rain on accumulated snow.Most precipitation records are based on a measured depth of water received within a fixed time interval. Frequency of a precipitation threshold of interest may be determined from the number of measurements exceeding that threshold value within the total time period for which observations are available. Individual data points are converted to intensity by dividing each measured depth by the period of time between observations. This intensity will be less than the actual peak intensity if the duration of the rainfall event was less than the fixed time interval for which measurements are reported. Convective precipitation events (thunderstorms) tend to produce shorter duration storm events than orographic precipitation. Duration, intensity, and frequency of rainfall events are important to flood prediction. Short duration precipitation is more significant to flooding within small drainage basins.
The most important upslope factor in determining flood magnitude is the land area of the watershed upstream of the area of interest. Rainfall intensity is the second most important factor for watersheds of less than approximately 30 square miles or 77.7 km². The main channel slope is the second most important factor for larger watersheds. Channel slope and rainfall intensity become the third most important factors for small and large watersheds, respectively.
Downslope factors
Water flowing downhill ultimately encounters downstream conditions slowing movement. The final limitation is often the oceanOcean
An ocean is a major body of saline water, and a principal component of the hydrosphere. Approximately 71% of the Earth's surface is covered by ocean, a continuous body of water that is customarily divided into several principal oceans and smaller seas.More than half of this area is over 3,000...
or a natural or artificial lake
Lake
A lake is a body of relatively still fresh or salt water of considerable size, localized in a basin, that is surrounded by land. Lakes are inland and not part of the ocean and therefore are distinct from lagoons, and are larger and deeper than ponds. Lakes can be contrasted with rivers or streams,...
. Elevation changes such as tidal fluctuations are significant determinants of coastal and estuarine flooding. Less predictable events like tsunami
Tsunami
A tsunami is a series of water waves caused by the displacement of a large volume of a body of water, typically an ocean or a large lake...
s and storm surge
Storm surge
A storm surge is an offshore rise of water associated with a low pressure weather system, typically tropical cyclones and strong extratropical cyclones. Storm surges are caused primarily by high winds pushing on the ocean's surface. The wind causes the water to pile up higher than the ordinary sea...
s may also cause elevation changes in large bodies of water. Elevation of flowing water is controlled by the geometry of the flow channel. Flow channel restrictions like bridges and canyons tend to control water elevation above the restriction. The actual control point for any given reach of the drainage may change with changing water elevation, so a closer point may control for lower water levels until a more distant point controls at higher water levels.
Effective flood channel geometry may be changed by growth of vegetation, accumulation of ice or debris, or construction of bridges, buildings, or levees within the flood channel.
Prediction
Statistical analysis requires all data in a series be gathered under similar conditions. A simple prediction model might be based upon observed flows within a fixed channel geometry. Alternatively, prediction may rely upon assumed channel geometry and runoff patterns using historical precipitation records. The rational method has been used for drainage basins small enough that observed rainfall intensities may be assumed to occur uniformly over the entire basin. Time of ConcentrationTime of Concentration
Time of concentration is a concept used in hydrology to measure the response of a watershed to a rain event. It is defined as the time needed for water to flow from the most remote point in a watershed to the watershed outlet...
is the time required for runoff from the most distant point of the upstream drainage area to reach the point of the drainage channel controlling flooding of the area of interest. The time of concentration defines the critical duration of peak rainfall for the area of interest. The critical duration of intense rainfall might be only a few minutes for roof and parking lot drainage structures, while cumulative rainfall over several days would be critical for river basins.
Extreme flood events often result from coincidence such as unusually intense, warm rainfall melting heavy snow pack, producing channel obstructions from floating ice, and releasing small impoundments like beaver
Beaver
The beaver is a primarily nocturnal, large, semi-aquatic rodent. Castor includes two extant species, North American Beaver and Eurasian Beaver . Beavers are known for building dams, canals, and lodges . They are the second-largest rodent in the world...
dams. Coincident events may cause flooding outside the statistical distribution anticipated by simplistic prediction models. Debris modification of channel geometry is common when heavy flows move uprooted woody vegetation and flood-damaged structures and vehicles, including boats and railway equipment.
External links
- "What is a 100 year flood?". Boulder Area Sustainability Information Network (BASIN). URL accessed 2006-06-16.

