
Van der Pol oscillator
Encyclopedia
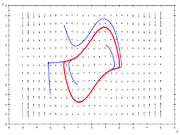
Dynamical system
A dynamical system is a concept in mathematics where a fixed rule describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a...
, the Van der Pol oscillator is a non-conservative
Conservative force
A conservative force is a force with the property that the work done in moving a particle between two points is independent of the path taken. Equivalently, if a particle travels in a closed loop, the net work done by a conservative force is zero.It is possible to define a numerical value of...
oscillator with non-linear
Nonlinearity
In mathematics, a nonlinear system is one that does not satisfy the superposition principle, or one whose output is not directly proportional to its input; a linear system fulfills these conditions. In other words, a nonlinear system is any problem where the variable to be solved for cannot be...
damping
Damping
In physics, damping is any effect that tends to reduce the amplitude of oscillations in an oscillatory system, particularly the harmonic oscillator.In mechanics, friction is one such damping effect...
. It evolves in time according to the second order differential equation
Differential equation
A differential equation is a mathematical equation for an unknown function of one or several variables that relates the values of the function itself and its derivatives of various orders...
:

where x is the position coordinate
Coordinate system
In geometry, a coordinate system is a system which uses one or more numbers, or coordinates, to uniquely determine the position of a point or other geometric element. The order of the coordinates is significant and they are sometimes identified by their position in an ordered tuple and sometimes by...
— which is a function
Function (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
of the time t, and μ is a scalar
Scalar (mathematics)
In linear algebra, real numbers are called scalars and relate to vectors in a vector space through the operation of scalar multiplication, in which a vector can be multiplied by a number to produce another vector....
parameter indicating the nonlinearity and the strength of the damping.
History
The Van der Pol oscillator was originally proposed by the Dutch electrical engineerElectrical engineering
Electrical engineering is a field of engineering that generally deals with the study and application of electricity, electronics and electromagnetism. The field first became an identifiable occupation in the late nineteenth century after commercialization of the electric telegraph and electrical...
and physicist Balthasar van der Pol
Balthasar van der Pol
Balthasar van der Pol was a Dutch physicist.Van der Pol studied physics in Utrecht, and in 1920 he was awarded his doctorate . He studied experimental physics with John Ambrose Fleming and Sir J. J. Thomson in England...
whilst he was working at Philips
Philips
Koninklijke Philips Electronics N.V. , more commonly known as Philips, is a multinational Dutch electronics company....
. Van der Pol found stable oscillations, which he called relaxation-oscillations
Relaxation oscillator
A relaxation oscillator is an oscillator based upon the behavior of a physical system's return to equilibrium after being disturbed. That is, a dynamical system within the oscillator continuously dissipates its internal energy...
and are now known as limit cycles, in electrical circuits employing vacuum tube
Vacuum tube
In electronics, a vacuum tube, electron tube , or thermionic valve , reduced to simply "tube" or "valve" in everyday parlance, is a device that relies on the flow of electric current through a vacuum...
s. When these circuits were driven near the limit cycle they become entrained
Entrainment (physics)
Entrainment has been used to refer to the process of mode locking of coupled driven oscillators, which is the process whereby two interacting oscillating systems, which have different periods when they function independently, assume a common period...
, i.e. the driving signal
Signal (electrical engineering)
In the fields of communications, signal processing, and in electrical engineering more generally, a signal is any time-varying or spatial-varying quantity....
pulls the current along with it. Van der Pol and his colleague, van der Mark, reported in the September 1927 issue of Nature
Nature (journal)
Nature, first published on 4 November 1869, is ranked the world's most cited interdisciplinary scientific journal by the Science Edition of the 2010 Journal Citation Reports...
that at certain drive frequencies
Frequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
an irregular noise was heard. This irregular noise was always heard near the natural entrainment frequencies. This was one of the first discovered instances of deterministic chaos
Chaos theory
Chaos theory is a field of study in mathematics, with applications in several disciplines including physics, economics, biology, and philosophy. Chaos theory studies the behavior of dynamical systems that are highly sensitive to initial conditions, an effect which is popularly referred to as the...
.
The Van der Pol equation has a long history of being used in both the physical
Physical science
Physical science is an encompassing term for the branches of natural science and science that study non-living systems, in contrast to the life sciences...
and biological
Biology
Biology is a natural science concerned with the study of life and living organisms, including their structure, function, growth, origin, evolution, distribution, and taxonomy. Biology is a vast subject containing many subdivisions, topics, and disciplines...
science
Science
Science is a systematic enterprise that builds and organizes knowledge in the form of testable explanations and predictions about the universe...
s. For instance, in biology, Fitzhugh and Nagumo extended the equation in a planar field
Plane (mathematics)
In mathematics, a plane is a flat, two-dimensional surface. A plane is the two dimensional analogue of a point , a line and a space...
as a model
FitzHugh–Nagumo model
The FitzHugh–Nagumo model, named after Richard FitzHugh who suggested the system in 1961 and J. Nagumo et al. who created the equivalent circuit the following year, describes a prototype of an excitable system ....
for action potential
Action potential
In physiology, an action potential is a short-lasting event in which the electrical membrane potential of a cell rapidly rises and falls, following a consistent trajectory. Action potentials occur in several types of animal cells, called excitable cells, which include neurons, muscle cells, and...
s of neurons. The equation has also been utilised in seismology
Seismology
Seismology is the scientific study of earthquakes and the propagation of elastic waves through the Earth or through other planet-like bodies. The field also includes studies of earthquake effects, such as tsunamis as well as diverse seismic sources such as volcanic, tectonic, oceanic,...
to model the two plates in a geological fault.
Two dimensional form
Liénard's TheoremLiénard's theorem
In mathematics, more specifically in the study of dynamical systems and differential equations, a Liénard equation is a second order differential equation, named after the French physicist Alfred-Marie Liénard....
can be used to prove that the system has a limit cycle. Applying the Liénard transformation
 , where the dot indicates the time derivative, the Van der Pol oscillator can be written in its two dimensional form:
, where the dot indicates the time derivative, the Van der Pol oscillator can be written in its two dimensional form:

Results for the unforced oscillator
Two interesting regimes for the characteristics of the unforced oscillator are:- When μ = 0, i.e. there is no damping function, the equation becomes:
- This is a form of the simple harmonic oscillatorHarmonic oscillatorIn classical mechanics, a harmonic oscillator is a system that, when displaced from its equilibrium position, experiences a restoring force, F, proportional to the displacement, x: \vec F = -k \vec x \, where k is a positive constant....
and there is always conservation of energyConservation of energyThe nineteenth century law of conservation of energy is a law of physics. It states that the total amount of energy in an isolated system remains constant over time. The total energy is said to be conserved over time...
.
- When μ > 0, the system will enter a limit cycle, where energy continues to be conserved. Near the origin x = dx/dt = 0 the system is unstable, and far from the origin the system is damped. Energy can be lost or gained, and work is done, if the system does not enter a limit cycle immediately.
The forced Van der Pol oscillator
The forced, or driven, Van der Pol oscillator takes the 'original' function and adds a driving function Asin(ωt) to give a differential equation of the form:
where A is the amplitude
Amplitude
Amplitude is the magnitude of change in the oscillating variable with each oscillation within an oscillating system. For example, sound waves in air are oscillations in atmospheric pressure and their amplitudes are proportional to the change in pressure during one oscillation...
, or displacement
Displacement (vector)
A displacement is the shortest distance from the initial to the final position of a point P. Thus, it is the length of an imaginary straight path, typically distinct from the path actually travelled by P...
, of the wave function
Wave equation
The wave equation is an important second-order linear partial differential equation for the description of waves – as they occur in physics – such as sound waves, light waves and water waves. It arises in fields like acoustics, electromagnetics, and fluid dynamics...
and ω is its angular velocity
Angular velocity
In physics, the angular velocity is a vector quantity which specifies the angular speed of an object and the axis about which the object is rotating. The SI unit of angular velocity is radians per second, although it may be measured in other units such as degrees per second, revolutions per...
.
Popular Culture
Author James GleickJames Gleick
James Gleick is an American author, journalist, and biographer, whose books explore the cultural ramifications of science and technology...
described a vacuum-tube Van der Pol oscillator in his book Chaos: Making a New Science
Chaos: Making a New Science
Chaos: Making A New Science is the best-selling book by James Gleick that first introduced the principles and early development of chaos theory to the public...
. According to a New York Times article, Gleick received a modern electronic Van der Pol oscillator from a reader in 1988.


