The universal parabolic constant is a mathematical constant

Mathematical constant

A mathematical constant is a special number, usually a real number, that is "significantly interesting in some way". Constants arise in many different areas of mathematics, with constants such as and occurring in such diverse contexts as geometry, number theory and calculus.What it means for a...

.

It is defined as the ratio, for any parabola

Parabola

In mathematics, the parabola is a conic section, the intersection of a right circular conical surface and a plane parallel to a generating straight line of that surface...

, of the arc length

Arc length

Determining the length of an irregular arc segment is also called rectification of a curve. Historically, many methods were used for specific curves...

 of the parabolic segment formed by the latus rectum to the focal parameter. It is denoted P₂.

In the diagram, the latus rectum is pictured in blue, the parabolic segment that it forms in red and the focal parameter in green.

The value of P₂ is

The circle

Circle

A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....

 and parabola are unique among conic sections in that they have a universal constant. The analogous ratios for ellipse

Ellipse

In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...

s and hyperbola

Hyperbola

In mathematics a hyperbola is a curve, specifically a smooth curve that lies in a plane, which can be defined either by its geometric properties or by the kinds of equations for which it is the solution set. A hyperbola has two pieces, called connected components or branches, which are mirror...

s depend on their eccentricities

Eccentricity (mathematics)

In mathematics, the eccentricity, denoted e or \varepsilon, is a parameter associated with every conic section. It can be thought of as a measure of how much the conic section deviates from being circular.In particular,...

. This means that all circles are similar

Similarity (geometry)

Two geometrical objects are called similar if they both have the same shape. More precisely, either one is congruent to the result of a uniform scaling of the other...

 and all parabolas are similar, whereas ellipses and hyperbolas are not.

Derivation

Take as the equation of the parabola. The focal parameter is 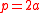and the semilatus rectum is .

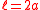Properties

P₂ is a transcendental number

Transcendental number

In mathematics, a transcendental number is a number that is not algebraic—that is, it is not a root of a non-constant polynomial equation with rational coefficients. The most prominent examples of transcendental numbers are π and e...

.

Proof. Suppose that P₂ is algebraic

Algebraic number

In mathematics, an algebraic number is a number that is a root of a non-zero polynomial in one variable with rational coefficients. Numbers such as π that are not algebraic are said to be transcendental; almost all real numbers are transcendental...

. Then must also be algebraic. However, by the Lindemann–Weierstrass theorem

Lindemann–Weierstrass theorem

In mathematics, the Lindemann–Weierstrass theorem is a result that is very useful in establishing the transcendence of numbers. It states that if 1, ...,  are algebraic numbers which are linearly independent over the rational numbers ', then 1, ...,  are algebraically...

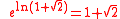, would be transcendental, which is not the case. Hence P₂ is transcendental.

Since P₂ is transcendental, it is also irrational

Irrational number

In mathematics, an irrational number is any real number that cannot be expressed as a ratio a/b, where a and b are integers, with b non-zero, and is therefore not a rational number....

.

Applications

The average distance from a point randomly selected in the unit square to its center is

The source of this article is wikipedia, the free encyclopedia.  The text of this article is licensed under the GFDL.