
Stochastic calculus
Encyclopedia
Stochastic calculus is a branch of mathematics
that operates on stochastic process
es. It allows a consistent theory of integration to be defined for integrals of stochastic processes with respect to stochastic processes. It is used to model systems that behave randomly.
The best-known stochastic process to which stochastic calculus is applied is the Wiener process
(named in honor of Norbert Wiener
), which is used for modeling Brownian motion
as described by Albert Einstein
and other physical diffusion
processes in space of particles subject to random forces. Since the 1970s, the Wiener process has been widely applied in financial mathematics and economics
to model the evolution in time of stock prices and bond interest rates.
The main flavours of stochastic calculus are the Itō calculus
and its variational relative the Malliavin calculus
. For technical reasons the Itō integral is the most useful for general classes of processes but the related Stratonovich integral
is frequently useful in problem formulation (particularly in engineering disciplines.) The Stratonovich integral can readily be expressed in terms of the Itō integral. The main benefit of the Stratonovich integral is that it obeys the usual chain rule
and does therefore not require Itō's lemma
. This enables problems to be expressed in a co-ordinate system invariant form, which is invaluable when developing stochastic calculus on manifolds other than Rn.
The dominated convergence theorem
does not hold for the Stratonovich integral, consequently it is very difficult to prove results without re-expressing the integrals in Itō form.
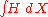 is defined for a semimartingale
is defined for a semimartingale
X and locally bounded predictable process H.
 against another semimartingale
against another semimartingale
Y can be defined in terms of the Itō integral as

where [X, Y]tc denotes the quadratic covariation
of the continuous parts of X
and Y. The alternative notation
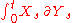
is also used to denote the Stratonovich integral.
.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
that operates on stochastic process
Stochastic process
In probability theory, a stochastic process , or sometimes random process, is the counterpart to a deterministic process...
es. It allows a consistent theory of integration to be defined for integrals of stochastic processes with respect to stochastic processes. It is used to model systems that behave randomly.
The best-known stochastic process to which stochastic calculus is applied is the Wiener process
Wiener process
In mathematics, the Wiener process is a continuous-time stochastic process named in honor of Norbert Wiener. It is often called standard Brownian motion, after Robert Brown...
(named in honor of Norbert Wiener
Norbert Wiener
Norbert Wiener was an American mathematician.A famous child prodigy, Wiener later became an early researcher in stochastic and noise processes, contributing work relevant to electronic engineering, electronic communication, and control systems.Wiener is regarded as the originator of cybernetics, a...
), which is used for modeling Brownian motion
Brownian motion
Brownian motion or pedesis is the presumably random drifting of particles suspended in a fluid or the mathematical model used to describe such random movements, which is often called a particle theory.The mathematical model of Brownian motion has several real-world applications...
as described by Albert Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
and other physical diffusion
Diffusion
Molecular diffusion, often called simply diffusion, is the thermal motion of all particles at temperatures above absolute zero. The rate of this movement is a function of temperature, viscosity of the fluid and the size of the particles...
processes in space of particles subject to random forces. Since the 1970s, the Wiener process has been widely applied in financial mathematics and economics
Economics
Economics is the social science that analyzes the production, distribution, and consumption of goods and services. The term economics comes from the Ancient Greek from + , hence "rules of the house"...
to model the evolution in time of stock prices and bond interest rates.
The main flavours of stochastic calculus are the Itō calculus
Ito calculus
Itō calculus, named after Kiyoshi Itō, extends the methods of calculus to stochastic processes such as Brownian motion . It has important applications in mathematical finance and stochastic differential equations....
and its variational relative the Malliavin calculus
Malliavin calculus
The Malliavin calculus, named after Paul Malliavin, is a theory of variational stochastic calculus. In other words it provides the mechanics to compute derivatives of random variables....
. For technical reasons the Itō integral is the most useful for general classes of processes but the related Stratonovich integral
Stratonovich integral
In stochastic processes, the Stratonovich integral is a stochastic integral, the most common alternative to the Itō integral...
is frequently useful in problem formulation (particularly in engineering disciplines.) The Stratonovich integral can readily be expressed in terms of the Itō integral. The main benefit of the Stratonovich integral is that it obeys the usual chain rule
Chain rule
In calculus, the chain rule is a formula for computing the derivative of the composition of two or more functions. That is, if f is a function and g is a function, then the chain rule expresses the derivative of the composite function in terms of the derivatives of f and g.In integration, the...
and does therefore not require Itō's lemma
Ito's lemma
In mathematics, Itō's lemma is used in Itō stochastic calculus to find the differential of a function of a particular type of stochastic process. It is named after its discoverer, Kiyoshi Itō...
. This enables problems to be expressed in a co-ordinate system invariant form, which is invaluable when developing stochastic calculus on manifolds other than Rn.
The dominated convergence theorem
Dominated convergence theorem
In measure theory, Lebesgue's dominated convergence theorem provides sufficient conditions under which two limit processes commute, namely Lebesgue integration and almost everywhere convergence of a sequence of functions...
does not hold for the Stratonovich integral, consequently it is very difficult to prove results without re-expressing the integrals in Itō form.
Itō integral
The Itō integral is central to the study of stochastic calculus. The integral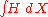 is defined for a semimartingale
is defined for a semimartingaleSemimartingale
In probability theory, a real valued process X is called a semimartingale if it can be decomposed as the sum of a local martingale and an adapted finite-variation process....
X and locally bounded predictable process H.
Stratonovich integral
The Stratonovich integral of a semimartingaleSemimartingale
In probability theory, a real valued process X is called a semimartingale if it can be decomposed as the sum of a local martingale and an adapted finite-variation process....
 against another semimartingale
against another semimartingaleSemimartingale
In probability theory, a real valued process X is called a semimartingale if it can be decomposed as the sum of a local martingale and an adapted finite-variation process....
Y can be defined in terms of the Itō integral as

where [X, Y]tc denotes the quadratic covariation
Quadratic variation
In mathematics, quadratic variation is used in the analysis of stochastic processes such as Brownian motion and martingales. Quadratic variation is just one kind of variation of a process.- Definition :...
of the continuous parts of X
and Y. The alternative notation
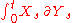
is also used to denote the Stratonovich integral.
Applications
A very important application of stochastic calculus is in quantitative finance, in which asset prices are often assumed to follow stochastic differential equations. In the Black-Scholes model, prices are assumed to follow the geometric Brownian motionGeometric Brownian motion
A geometric Brownian motion is a continuous-time stochastic process in which the logarithm of the randomly varying quantity follows a Brownian motion, also called a Wiener process...
.

