
Skyrmion
Encyclopedia
In theoretical physics
, a skyrmion is a mathematical model used to model baryon
s (a subatomic particle
). It was conceived by Tony Skyrme
.
non-trivial classical solution of a nonlinear sigma model with a non-trivial target manifold topology
—a particular case of a topological soliton. It arises, for example, in chiral model
s of mesons where the target manifold is a homogeneous space
of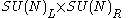
(the structure group)
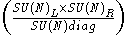
where SU(N)L and SU(N)R are the left and right copies respectively, and SU(N)diag is the diagonal subgroup.
If spacetime
has the topology S3×R (for space
and time
respectively), then classical configurations are classified by an integral winding number
because the third homotopy group
,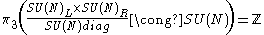
(The congruence sign here refers to homeomorphism
, not isomorphism.)
It is possible to add a topological term to the chiral lagrangian whose integral only depends upon the homotopy class. This results in superselection sector
s in the quantized model. A toy model for Skyrmion is soliton
of Sine-Gordon equation
. It can be quantized semi-classically or solved exactly by Bethe Ansatz
. After quantization soliton of Sine-Gordon turns into a fermion
interacting by means of massive Thirring model
.
s, it is predicted that Skyrmions could arise in Bose-Einstein Condensates, and in superconductors. Skyrmions have also been experimentally observed to describe certain chiral magnetic vortices in thin layers of magnetic materials.
Theoretical physics
Theoretical physics is a branch of physics which employs mathematical models and abstractions of physics to rationalize, explain and predict natural phenomena...
, a skyrmion is a mathematical model used to model baryon
Baryon
A baryon is a composite particle made up of three quarks . Baryons and mesons belong to the hadron family, which are the quark-based particles...
s (a subatomic particle
Subatomic particle
In physics or chemistry, subatomic particles are the smaller particles composing nucleons and atoms. There are two types of subatomic particles: elementary particles, which are not made of other particles, and composite particles...
). It was conceived by Tony Skyrme
Tony Skyrme
Tony Hilton Royle Skyrme, was a British physicist. He first proposed modeling the effective interaction between nucleons in nuclei by a zero-range potential, an idea still widely used today in nuclear structure and in equation of state for neutron stars. However, he is best known for formulating...
.
Overview
A skyrmion is a homotopicallyHomotopy
In topology, two continuous functions from one topological space to another are called homotopic if one can be "continuously deformed" into the other, such a deformation being called a homotopy between the two functions...
non-trivial classical solution of a nonlinear sigma model with a non-trivial target manifold topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
—a particular case of a topological soliton. It arises, for example, in chiral model
Chiral model
In nuclear physics, the chiral model is a phenomenological model describing mesons in the chiral limit where the masses of the quarks go to zero . It's a nonlinear sigma model with the principal homogeneous space of the Lie group SU as its target manifold where N is the number of quark flavors...
s of mesons where the target manifold is a homogeneous space
Homogeneous space
In mathematics, particularly in the theories of Lie groups, algebraic groups and topological groups, a homogeneous space for a group G is a non-empty manifold or topological space X on which G acts continuously by symmetry in a transitive way. A special case of this is when the topological group,...
of
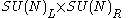
(the structure group)
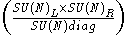
where SU(N)L and SU(N)R are the left and right copies respectively, and SU(N)diag is the diagonal subgroup.
If spacetime
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
has the topology S3×R (for space
Space
Space is the boundless, three-dimensional extent in which objects and events occur and have relative position and direction. Physical space is often conceived in three linear dimensions, although modern physicists usually consider it, with time, to be part of a boundless four-dimensional continuum...
and time
Time
Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects....
respectively), then classical configurations are classified by an integral winding number
Winding number
In mathematics, the winding number of a closed curve in the plane around a given point is an integer representing the total number of times that curve travels counterclockwise around the point...
because the third homotopy group
Homotopy group
In mathematics, homotopy groups are used in algebraic topology to classify topological spaces. The first and simplest homotopy group is the fundamental group, which records information about loops in a space...
,
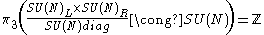
(The congruence sign here refers to homeomorphism
Homeomorphism
In the mathematical field of topology, a homeomorphism or topological isomorphism or bicontinuous function is a continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphisms in the category of topological spaces—that is, they are...
, not isomorphism.)
It is possible to add a topological term to the chiral lagrangian whose integral only depends upon the homotopy class. This results in superselection sector
Superselection sector
In Quantum mechanics, superselection extends the concept of selection rules.Superselection rules are postulated rules forbidding the preparation of quantum states that exhibit coherence between eigenstates of certain observables....
s in the quantized model. A toy model for Skyrmion is soliton
Soliton
In mathematics and physics, a soliton is a self-reinforcing solitary wave that maintains its shape while it travels at constant speed. Solitons are caused by a cancellation of nonlinear and dispersive effects in the medium...
of Sine-Gordon equation
Sine-Gordon equation
The sine–Gordon equation is a nonlinear hyperbolic partial differential equation in 1 + 1 dimensions involving the d'Alembert operator and the sine of the unknown function. It was originally considered in the nineteenth century in the course of study of surfaces of constant negative...
. It can be quantized semi-classically or solved exactly by Bethe Ansatz
Bethe ansatz
In physics, the Bethe ansatz is a method for finding the exact solutions of certain one-dimensional quantum many-body models. It was invented by Hans Bethe in 1931 to find the exact eigenvalues and eigenvectors of the one-dimensional antiferromagnetic Heisenberg model Hamiltonian...
. After quantization soliton of Sine-Gordon turns into a fermion
Fermion
In particle physics, a fermion is any particle which obeys the Fermi–Dirac statistics . Fermions contrast with bosons which obey Bose–Einstein statistics....
interacting by means of massive Thirring model
Thirring model
The Thirring model is an exactly solvable quantum field theory which describes the self-interactions of a Dirac field in two dimension.-Definition:The Thirring model is given by the Lagrangian density...
.
Other Applications
Besides baryonBaryon
A baryon is a composite particle made up of three quarks . Baryons and mesons belong to the hadron family, which are the quark-based particles...
s, it is predicted that Skyrmions could arise in Bose-Einstein Condensates, and in superconductors. Skyrmions have also been experimentally observed to describe certain chiral magnetic vortices in thin layers of magnetic materials.

