
Shape of the distribution
Encyclopedia
In statistics
, the concept of the shape of the distribution refers to the shape
of a probability distribution
and it most often arises in questions of finding an appropriate distribution to use to model the statistical properties of a population, given a sample from that population. The shape of a distribution may be considered either descriptively, using terms such as "J-shaped", or numerically, using quantitative measures such as skewness
and kurtosis
.
Considerations of the shape of a distribution arise in statistical data analysis
, where simple quantitative descriptive statistics and plotting techniques such as histograms can lead on to the selection of a particular family of distributions for modelling purposes.
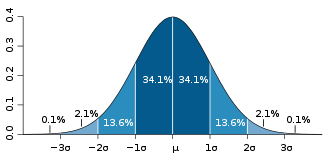
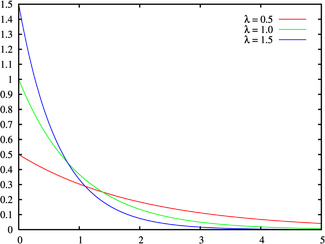
would have two high points rather than one. The shape of a distribution is sometimes characterised by the behaviours of the tails (as in a long or short tail). For example a flat distribution can be said either to have no tails, or to have short tails. A normal distribution is usually regarded as having short tails, while an exponential distribution
has exponential tails and a Pareto distribution has long tails.
Even in the relatively simple case of a mounded distribution, the distribution may be skewed to the left or skewed to the right, with symmetric corresponding to no skew.
Statistics
Statistics is the study of the collection, organization, analysis, and interpretation of data. It deals with all aspects of this, including the planning of data collection in terms of the design of surveys and experiments....
, the concept of the shape of the distribution refers to the shape
Shape
The shape of an object located in some space is a geometrical description of the part of that space occupied by the object, as determined by its external boundary – abstracting from location and orientation in space, size, and other properties such as colour, content, and material...
of a probability distribution
Probability distribution
In probability theory, a probability mass, probability density, or probability distribution is a function that describes the probability of a random variable taking certain values....
and it most often arises in questions of finding an appropriate distribution to use to model the statistical properties of a population, given a sample from that population. The shape of a distribution may be considered either descriptively, using terms such as "J-shaped", or numerically, using quantitative measures such as skewness
Skewness
In probability theory and statistics, skewness is a measure of the asymmetry of the probability distribution of a real-valued random variable. The skewness value can be positive or negative, or even undefined...
and kurtosis
Kurtosis
In probability theory and statistics, kurtosis is any measure of the "peakedness" of the probability distribution of a real-valued random variable...
.
Considerations of the shape of a distribution arise in statistical data analysis
Data analysis
Analysis of data is a process of inspecting, cleaning, transforming, and modeling data with the goal of highlighting useful information, suggesting conclusions, and supporting decision making...
, where simple quantitative descriptive statistics and plotting techniques such as histograms can lead on to the selection of a particular family of distributions for modelling purposes.


Descriptions of shape
The shape of a distribution will fall somewhere in a continuum where a flat distribution might be considered central and where types of departure from this include: mounded (or unimodal), U-shaped, J-shaped, reverse-J shaped and multi-modal. A bimodal distributionBimodal distribution
In statistics, a bimodal distribution is a continuous probability distribution with two different modes. These appear as distinct peaks in the probability density function, as shown in Figure 1....
would have two high points rather than one. The shape of a distribution is sometimes characterised by the behaviours of the tails (as in a long or short tail). For example a flat distribution can be said either to have no tails, or to have short tails. A normal distribution is usually regarded as having short tails, while an exponential distribution
Exponential distribution
In probability theory and statistics, the exponential distribution is a family of continuous probability distributions. It describes the time between events in a Poisson process, i.e...
has exponential tails and a Pareto distribution has long tails.
Even in the relatively simple case of a mounded distribution, the distribution may be skewed to the left or skewed to the right, with symmetric corresponding to no skew.

