Rotation system
Encyclopedia
In combinatorial
mathematics
, rotation systems encode embeddings of graphs
onto orientable
surface
s, by describing the circular ordering of a graph's edges around each vertex.
A more formal definition of a rotation system involves pairs of permutations; such a pair is sufficient to determine a multigraph, a surface, and a 2-cell embedding
of the multigraph onto the surface.
Every rotation scheme defines a unique 2-cell embedding of a connected multigraph on a closed oriented surface (up to orientation preserving topological equivalence). Conversely, any embedding of a connected multigraph G on an oriented closed surface defines a unique rotation system having G as its underlying multigraph. This fundamental equivalence between rotation systems and 2-cell-embeddings was first settled in a dual form by Heffter and extensively used by Ringel
during the 1950s. Independently, Edmonds
gave the primal form of the theorem and the details of his study have been popularized by Youngs. The generalization to the whole set of multigraphs was developed by Gross and Alpert.
Rotation systems are related to, but not the same as, the rotation map
s used by Reingold et al. (2002) to define the zig-zag product
of graphs. A rotation system specifies a circular ordering of the edges around each vertex, while a rotation map specifies a (non-circular) permutation of the edges at each vertex. In addition, rotation systems can be defined for any graph, while as Reingold et al. define them rotation maps are restricted to regular graph
s.
<σ,θ> generated
by σ and θ acts transitively
on B.
To derive a rotation system from a 2-cell embedding of a connected multigraph G on an oriented surface, let B consist of the darts (or flags, or half-edges) of G; that is, for each edge of G we form two elements of B, one for each endpoint of the edge. Even when an edge has the same vertex as both of its endpoints, we create two darts for that edge. We let θ(b) be the other dart formed from the same edge as b; this is clearly an involution with no fixed points. We let σ(b) be the dart in the clockwise position from b in the cyclic order of edges incident to the same vertex, where "clockwise" is defined by the orientation of the surface.
If a multigraph is embedded on an orientable but not oriented surface, it generally corresponds to two rotation systems, one for each of the two orientations of the surface. These two rotation systems have the same involution θ, but the permutation σ for one rotation system is the inverse of the corresponding permutation for the other rotation system.
To embed the graph derived from a rotation system onto a surface, form a disk for each orbit of σθ, and glue two disks together along an edge e whenever the two darts corresponding to e belong to the two orbits corresponding to these disks. The result is a 2-cell embedding of the derived multigraph, the two-cells of which are the disks corresponding to the orbits of σθ. The surface of this embedding can be oriented in such a way that the clockwise ordering of the edges around each vertex is the same as the clockwise ordering given by σ.
g of the closed orientable surface defined by the rotation system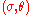
(that is, the surface on which the underlying multigraph is 2-cell embedded):
where
denotes the set of the orbits of permutation 
.
Combinatorics
Combinatorics is a branch of mathematics concerning the study of finite or countable discrete structures. Aspects of combinatorics include counting the structures of a given kind and size , deciding when certain criteria can be met, and constructing and analyzing objects meeting the criteria ,...
mathematics
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, rotation systems encode embeddings of graphs
Graph theory
In mathematics and computer science, graph theory is the study of graphs, mathematical structures used to model pairwise relations between objects from a certain collection. A "graph" in this context refers to a collection of vertices or 'nodes' and a collection of edges that connect pairs of...
onto orientable
Orientability
In mathematics, orientability is a property of surfaces in Euclidean space measuring whether or not it is possible to make a consistent choice of surface normal vector at every point. A choice of surface normal allows one to use the right-hand rule to define a "clockwise" direction of loops in the...
surface
Surface
In mathematics, specifically in topology, a surface is a two-dimensional topological manifold. The most familiar examples are those that arise as the boundaries of solid objects in ordinary three-dimensional Euclidean space R3 — for example, the surface of a ball...
s, by describing the circular ordering of a graph's edges around each vertex.
A more formal definition of a rotation system involves pairs of permutations; such a pair is sufficient to determine a multigraph, a surface, and a 2-cell embedding
Graph embedding
In topological graph theory, an embedding of a graph G on a surface Σ is a representation of G on Σ in which points of Σ are associated to vertices and simple arcs are associated to edges in such a way that:...
of the multigraph onto the surface.
Every rotation scheme defines a unique 2-cell embedding of a connected multigraph on a closed oriented surface (up to orientation preserving topological equivalence). Conversely, any embedding of a connected multigraph G on an oriented closed surface defines a unique rotation system having G as its underlying multigraph. This fundamental equivalence between rotation systems and 2-cell-embeddings was first settled in a dual form by Heffter and extensively used by Ringel
Gerhard Ringel
Gerhard Ringel was a German mathematician who earned his Ph.D. from the University of Bonn in 1951...
during the 1950s. Independently, Edmonds
Jack Edmonds
Jack R. Edmonds is a mathematician, regarded as one of the most important contributors to the field of combinatorial optimization...
gave the primal form of the theorem and the details of his study have been popularized by Youngs. The generalization to the whole set of multigraphs was developed by Gross and Alpert.
Rotation systems are related to, but not the same as, the rotation map
Rotation map
In mathematics, a rotation map is a function that represents an undirected edge-labeled graph, where each vertex enumerates its outgoing neighbors...
s used by Reingold et al. (2002) to define the zig-zag product
Zig-zag product
In graph theory, the zig-zag product of regular graphs G,H, denoted by G \circ H, takes a large graph and a small graph , and produces a graph that approximately inherits the size of the large one but the degree of the small one...
of graphs. A rotation system specifies a circular ordering of the edges around each vertex, while a rotation map specifies a (non-circular) permutation of the edges at each vertex. In addition, rotation systems can be defined for any graph, while as Reingold et al. define them rotation maps are restricted to regular graph
Regular graph
In graph theory, a regular graph is a graph where each vertex has the same number of neighbors; i.e. every vertex has the same degree or valency. A regular directed graph must also satisfy the stronger condition that the indegree and outdegree of each vertex are equal to each other...
s.
Formal definition
Formally, a rotation system is defined as a pair (σ,θ) where σ and θ are permutations acting on the same ground set B, θ is a fixed-point-free involution, and the groupGroup (mathematics)
In mathematics, a group is an algebraic structure consisting of a set together with an operation that combines any two of its elements to form a third element. To qualify as a group, the set and the operation must satisfy a few conditions called group axioms, namely closure, associativity, identity...
<σ,θ> generated
Generating set of a group
In abstract algebra, a generating set of a group is a subset that is not contained in any proper subgroup of the group. Equivalently, a generating set of a group is a subset such that every element of the group can be expressed as the combination of finitely many elements of the subset and their...
by σ and θ acts transitively
Group action
In algebra and geometry, a group action is a way of describing symmetries of objects using groups. The essential elements of the object are described by a set, and the symmetries of the object are described by the symmetry group of this set, which consists of bijective transformations of the set...
on B.
To derive a rotation system from a 2-cell embedding of a connected multigraph G on an oriented surface, let B consist of the darts (or flags, or half-edges) of G; that is, for each edge of G we form two elements of B, one for each endpoint of the edge. Even when an edge has the same vertex as both of its endpoints, we create two darts for that edge. We let θ(b) be the other dart formed from the same edge as b; this is clearly an involution with no fixed points. We let σ(b) be the dart in the clockwise position from b in the cyclic order of edges incident to the same vertex, where "clockwise" is defined by the orientation of the surface.
If a multigraph is embedded on an orientable but not oriented surface, it generally corresponds to two rotation systems, one for each of the two orientations of the surface. These two rotation systems have the same involution θ, but the permutation σ for one rotation system is the inverse of the corresponding permutation for the other rotation system.
Recovering the embedding from the rotation system
To recover a multigraph from a rotation system, we form a vertex for each orbit of σ, and an edge for each orbit of θ. A vertex is incident with an edge if these two orbits have a nonempty intersection. Thus, the number of incidences per vertex is the size of the orbit, and the number of incidences per edge is exactly two. If a rotation system is derived from a 2-cell embedding of a connected multigraph G, the graph derived from the rotation system is isomorphic to G.To embed the graph derived from a rotation system onto a surface, form a disk for each orbit of σθ, and glue two disks together along an edge e whenever the two darts corresponding to e belong to the two orbits corresponding to these disks. The result is a 2-cell embedding of the derived multigraph, the two-cells of which are the disks corresponding to the orbits of σθ. The surface of this embedding can be oriented in such a way that the clockwise ordering of the edges around each vertex is the same as the clockwise ordering given by σ.
Characterizing the surface of the embedding
According to the Euler formula we can deduce the genusGenus (mathematics)
In mathematics, genus has a few different, but closely related, meanings:-Orientable surface:The genus of a connected, orientable surface is an integer representing the maximum number of cuttings along non-intersecting closed simple curves without rendering the resultant manifold disconnected. It...
g of the closed orientable surface defined by the rotation system
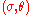(that is, the surface on which the underlying multigraph is 2-cell embedded):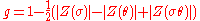where
denotes the set of the orbits of permutation .