
Poisson supermanifold
Encyclopedia
In differential geometry a Poisson supermanifold is a differential supermanifold
M such that the supercommutative algebra
of smooth function
s over it (to clarify this: M is not a point set space and so, doesn't "really" exist, and really, this algebra is all we have),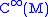 is equipped with a bilinear map called the Poisson superbracket turning it into a Poisson superalgebra
is equipped with a bilinear map called the Poisson superbracket turning it into a Poisson superalgebra
.
Every symplectic supermanifold is a Poisson supermanifold but not vice versa.
Supermanifold
In physics and mathematics, supermanifolds are generalizations of the manifold concept based on ideas coming from supersymmetry. Several definitions are in use, some of which are described below.- Physics :...
M such that the supercommutative algebra
Supercommutative algebra
In mathematics, a supercommutative algebra is a superalgebra such that for any two homogeneous elements x, y we haveyx = ^In mathematics, a supercommutative algebra is a superalgebra In mathematics, a supercommutative algebra is a superalgebra (i.e. a Z2-graded algebra) such that for any two...
of smooth function
Smooth function
In mathematical analysis, a differentiability class is a classification of functions according to the properties of their derivatives. Higher order differentiability classes correspond to the existence of more derivatives. Functions that have derivatives of all orders are called smooth.Most of...
s over it (to clarify this: M is not a point set space and so, doesn't "really" exist, and really, this algebra is all we have),
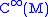 is equipped with a bilinear map called the Poisson superbracket turning it into a Poisson superalgebra
is equipped with a bilinear map called the Poisson superbracket turning it into a Poisson superalgebraPoisson superalgebra
In mathematics, a Poisson superalgebra is a Z2-graded generalization of a Poisson algebra. Specifically, a Poisson superalgebra is an superalgebra A with a Lie superbracket[\cdot,\cdot] : A\otimes A\to A...
.
Every symplectic supermanifold is a Poisson supermanifold but not vice versa.
See also
- Poisson manifoldPoisson manifoldIn mathematics, a Poisson manifold is a differentiable manifold M such that the algebra C^\infty\, of smooth functions over M is equipped with a bilinear map called the Poisson bracket, turning it into a Poisson algebra...
- Poisson algebraPoisson algebraIn mathematics, a Poisson algebra is an associative algebra together with a Lie bracket that also satisfies Leibniz' law; that is, the bracket is also a derivation. Poisson algebras appear naturally in Hamiltonian mechanics, and are also central in the study of quantum groups...
- Poisson superalgebraPoisson superalgebraIn mathematics, a Poisson superalgebra is a Z2-graded generalization of a Poisson algebra. Specifically, a Poisson superalgebra is an superalgebra A with a Lie superbracket[\cdot,\cdot] : A\otimes A\to A...
- noncommutative geometryNoncommutative geometryNoncommutative geometry is a branch of mathematics concerned with geometric approach to noncommutative algebras, and with construction of spaces which are locally presented by noncommutative algebras of functions...

