In mathematics

Mathematics

Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...

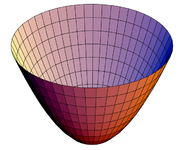
, a paraboloid is a quadric surface of special kind. There are two kinds of paraboloids: elliptic and hyperbolic. The elliptic paraboloid is shaped like an oval cup and can have a maximum or minimum point.
In a suitable coordinate system with three axes , , and , it can be represented by the equation


where and are constants that dictate the level of curvature in the - and - planes respectively.

This is an elliptic paraboloid which opens upward.

The hyperbolic paraboloid (not to be confused with a hyperboloid) is a doubly ruled surface shaped like a saddle

Saddle

A saddle is a supportive structure for a rider or other load, fastened to an animal's back by a girth. The most common type is the equestrian saddle designed for a horse, but specialized saddles have been created for camels and other creatures...

. In a suitable coordinate system, a hyperbolic paraboloid can be represented by the equation


For c>0, this is a hyperbolic paraboloid that opens up along the x-axis and down along the y-axis (i.e., the parabola in the plane x=0 opens upward and the parabola in the plane y=0 opens downward).

Properties

With a = b an elliptic paraboloid is a paraboloid of revolution: a surface obtained by revolving a parabola

Parabola

In mathematics, the parabola is a conic section, the intersection of a right circular conical surface and a plane parallel to a generating straight line of that surface...

 around its axis. It is the shape of the parabolic reflector

Parabolic reflector

A parabolic reflector is a reflective device used to collect or project energy such as light, sound, or radio waves. Its shape is that of a circular paraboloid, that is, the surface generated by a parabola revolving around its axis...

s used in mirror

Mirror

A mirror is an object that reflects light or sound in a way that preserves much of its original quality prior to its contact with the mirror. Some mirrors also filter out some wavelengths, while preserving other wavelengths in the reflection...

s, antenna dishes, and the like; and is also the shape of the surface of a rotating liquid, a principle used in liquid mirror telescopes. It is also called a circular paraboloid.

A point light source at the focal point produces a parallel light beam. This also works the other way around: a parallel beam of light incident on the paraboloid is concentrated at the focal point. This applies also for other waves, hence parabolic antenna

Parabolic antenna

A parabolic antenna is an antenna that uses a parabolic reflector, a curved surface with the cross-sectional shape of a parabola, to direct the radio waves. The most common form is shaped like a dish and is popularly called a dish antenna or parabolic dish...

s.

The hyperbolic paraboloid is a doubly ruled surface: it contains two families of mutually skew lines

Skew lines

In solid geometry, skew lines are two lines that do not intersect and are not parallel. Equivalently, they are lines that are not coplanar. A simple example of a pair of skew lines is the pair of lines through opposite edges of a regular tetrahedron...

. The lines in each family are parallel to a common plane, but not to each other.

Curvature

The elliptic paraboloid, parametrized simply as
has Gaussian curvature

Gaussian curvature

In differential geometry, the Gaussian curvature or Gauss curvature of a point on a surface is the product of the principal curvatures, κ1 and κ2, of the given point. It is an intrinsic measure of curvature, i.e., its value depends only on how distances are measured on the surface, not on the way...

and mean curvature

Mean curvature

In mathematics, the mean curvature H of a surface S is an extrinsic measure of curvature that comes from differential geometry and that locally describes the curvature of an embedded surface in some ambient space such as Euclidean space....

which are both always positive, have their maximum at the origin, become smaller as a point on the surface moves further away from the origin, and tend asymptotically to zero as the said point moves infinitely away from the origin.

The hyperbolic paraboloid, when parametrized as
has Gaussian curvature
and mean curvature

Multiplication table

If the hyperbolic paraboloid
is rotated by an angle of π/4 in the +z direction (according to the right hand rule), the result is the surface
and if then this simplifies to.
Finally, letting , we see that the hyperbolic paraboloid
is congruent to the surface
which can be thought of as the geometric representation (a three-dimensional nomograph

Nomogram

A nomogram, nomograph, or abac is a graphical calculating device developed by P.E. Elyasberg, a two-dimensional diagram designed to allow the approximate graphical computation of a function: it uses a coordinate system other than Cartesian coordinates...

, as it were) of a multiplication table

Multiplication table

In mathematics, a multiplication table is a mathematical table used to define a multiplication operation for an algebraic system....

.

The two paraboloidal functions
and
are harmonic conjugate

Harmonic conjugate

In mathematics, a function u defined on some open domain \Omega\subset\R^2 is said to have as a conjugate a function v if and only if they are respectively real and imaginary part of a holomorphic function f of the complex variable z:=x+iy\in\Omega. That is, v is conjugated to u if f:=u+iv is...

s, and together form the analytic function

Analytic function

In mathematics, an analytic function is a function that is locally given by a convergent power series. There exist both real analytic functions and complex analytic functions, categories that are similar in some ways, but different in others...

which is the analytic continuation

Analytic continuation

In complex analysis, a branch of mathematics, analytic continuation is a technique to extend the domain of a given analytic function. Analytic continuation often succeeds in defining further values of a function, for example in a new region where an infinite series representation in terms of which...

 of the parabolic function

Applications

The fluid in a spinning cylinder will form a paraboloid. This property can be used to make a liquid mirror telescope with a rotating pool of a reflective liquid, such as mercury, for the primary mirror.

The manufacturers of a widely-sold fried snack food, Pringles

Pringles

Pringles is a brand of potato and wheat based snacks originally developed by Procter & Gamble. Pringles are sold in more than 140 countries and have yearly sales of more than...

 ersatz potato chips, utilize a hyperbolic paraboloid shape in order to produce a stackable snack.

See also

• Quadratic form
  Quadratic form
  In mathematics, a quadratic form is a homogeneous polynomial of degree two in a number of variables. For example,4x^2 + 2xy - 3y^2\,\!is a quadratic form in the variables x and y....
  
  
• Ellipsoid
• Hyperboloid
• Hyperboloid structure
  Hyperboloid structure
  Hyperboloid structures are architectural structures designed with hyperboloid geometry. Often these are tall structures such as towers where the hyperboloid geometry's structural strength is used to support an object high off the ground, but hyperboloid geometry is also often used for decorative...
  
  
• Saddle roof
  Saddle roof
  A saddle roof is one which follows a convex curve about one axis and a concave curve about the other. The hyperbolic paraboloid form has been used for roofs at various times since it is easily constructed from straight sections of lumber, steel, or other conventional materials...
  
  
• Holophones