In geometric topology

Geometric topology

In mathematics, geometric topology is the study of manifolds and maps between them, particularly embeddings of one manifold into another.- Topics :...

, PDIFF, for piecewise differentiable, is the category

Category (mathematics)

In mathematics, a category is an algebraic structure that comprises "objects" that are linked by "arrows". A category has two basic properties: the ability to compose the arrows associatively and the existence of an identity arrow for each object. A simple example is the category of sets, whose...

 of piecewise

Piecewise

On mathematics, a piecewise-defined function is a function whose definition changes depending on the value of the independent variable...

-smooth

Smooth function

In mathematical analysis, a differentiability class is a classification of functions according to the properties of their derivatives. Higher order differentiability classes correspond to the existence of more derivatives. Functions that have derivatives of all orders are called smooth.Most of...

 manifolds and piecewise-smooth maps

Function (mathematics)

In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...

 between them. It properly contains Diff – the category of smooth manifolds and smooth function

Smooth function

In mathematical analysis, a differentiability class is a classification of functions according to the properties of their derivatives. Higher order differentiability classes correspond to the existence of more derivatives. Functions that have derivatives of all orders are called smooth.Most of...

s between them – and PL – the category of piecewise linear manifolds and piecewise linear maps between them – and the reason it is defined is to allow one to relate these two categories. Further, piecewise functions such as splines

Spline (mathematics)

In mathematics, a spline is a sufficiently smooth piecewise-polynomial function. In interpolating problems, spline interpolation is often preferred to polynomial interpolation because it yields similar results, even when using low-degree polynomials, while avoiding Runge's phenomenon for higher...

 and polygonal chain

Polygonal chain

A polygonal chain, polygonal curve, polygonal path, or piecewise linear curve, is a connected series of line segments. More formally, a polygonal chain P is a curve specified by a sequence of points \scriptstyle called its vertices so that the curve consists of the line segments connecting the...

s are common in mathematics, and PDIFF provides a category for discussing them.

Motivation

PDIFF is mostly a technical point: smooth maps are not piecewise linear (unless linear), and piecewise linear maps are not smooth (unless globally linear), so they cannot directly be related.

However, while a smooth manifold is not a PL manifold, it carries a canonical PL structure – it is uniquely triangularizable; conversely, not every PL manifold is smoothable. For a particular smooth manifold or smooth map between smooth manifolds, this can be shown by breaking up the manifold into small enough pieces, and then linearizing the manifold or map on each piece: for example, a circle in the plane can be approximated by a triangle, but not by a 2-gon, since this latter cannot be linearly embedded.

This relation between Diff and PL requires choices, however, and is more naturally shown and understood by including both categories in a larger category, and then showing that the inclusion of PL is an equivalence: every smooth manifold and every PL manifold is a PDiff manifold. Thus, going from Diff to PDiff and PL to PDiff are natural – they are just inclusion. The map PL to PDiff, while not an equality – not every piecewise smooth function is piecewise linear – is an equivalence: one can linearize pieces. Thus it can for some purposes be inverted, or considered an isomorphism, which gives a map 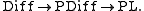These categories all sit inside TOP, the category of topological manifold and continuous maps between them.

In summary, PDiff is more general than Diff because it allows pieces (corners), and one cannot in general smooth corners, while PL is no less general that PDiff because one can linearize pieces (more precisely, one may need to break them up into smaller pieces and then linearize, which is allowed in PDiff).