
Order-7 triangular tiling
Encyclopedia
In geometry
, the order-7 triangular tiling is a regular tiling of the hyperbolic plane
with a Schläfli symbol of {3,7}.
The dual tiling is the order-3 heptagonal tiling.
Wythoff construction
s yields further uniform tiling
s, yielding eight uniform tilings, including the two regular ones given here.

The symmetry group of the tiling is the (2,3,7) triangle group
, and a fundamental domain for this action is the (2,3,7) Schwarz triangle
. This is the smallest hyperbolic Schwarz triangle, and thus, by the proof of Hurwitz's automorphisms theorem, the tiling is the universal tiling that covers all Hurwitz surface
s (the Riemann surfaces with maximal symmetry group), giving them a triangulation whose symmetry group equals their automorphism group as Riemann surfaces.
 The smallest of these is the Klein quartic
The smallest of these is the Klein quartic
, the most symmetric genus 3 surface, together with a tiling by 56 triangles, meeting at 24 vertices, with symmetry group the simple group of order 168, known as PSL(2,7)
. The resulting surface can in turn be polyhedrally immersed into Euclidean 3-space, yielding the small cubicuboctahedron
.
The dual order-3 heptagonal tiling has the same symmetry group, and thus yields heptagonal tilings of Hurwitz surfaces.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, the order-7 triangular tiling is a regular tiling of the hyperbolic plane
Hyperbolic geometry
In mathematics, hyperbolic geometry is a non-Euclidean geometry, meaning that the parallel postulate of Euclidean geometry is replaced...
with a Schläfli symbol of {3,7}.
Related polyhedra and tiling
This tiling is topologically related as a part of sequence of regular polyhedra with Schläfli symbol {3,p}. {3,3} Tetrahedron In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids... |
 {3,4} Octahedron In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex.... |
 {3,5} Icosahedron In geometry, an icosahedron is a regular polyhedron with 20 identical equilateral triangular faces, 30 edges and 12 vertices. It is one of the five Platonic solids.... |
|
 {3,6} |
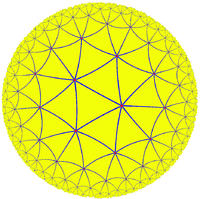 {3,7} |
 {3,8} |
 {3,9} |
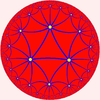
The dual tiling is the order-3 heptagonal tiling.
 order-3 heptagonal tiling |
 order-7 triangular tiling |
Wythoff construction
Wythoff construction
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.- Construction process :...
s yields further uniform tiling
Uniform tiling
In geometry, a uniform tiling is a tessellation of the plane by regular polygon faces with the restriction of being vertex-uniform.Uniform tilings can exist in both the Euclidean plane and hyperbolic plane...
s, yielding eight uniform tilings, including the two regular ones given here.
Hurwitz surfaces

The symmetry group of the tiling is the (2,3,7) triangle group
(2,3,7) triangle group
In the theory of Riemann surfaces and hyperbolic geometry, the triangle group is particularly important. This importance stems from its connection to Hurwitz surfaces, namely Riemann surfaces of genus g with the largest possible order, 84, of its automorphism group.A note on terminology – the "...
, and a fundamental domain for this action is the (2,3,7) Schwarz triangle
Schwarz triangle
In geometry, a Schwarz triangle, named after Hermann Schwarz is a spherical triangle that can be used to tile a sphere, possibly overlapping, through reflections in its edges. They were classified in ....
. This is the smallest hyperbolic Schwarz triangle, and thus, by the proof of Hurwitz's automorphisms theorem, the tiling is the universal tiling that covers all Hurwitz surface
Hurwitz surface
In Riemann surface theory and hyperbolic geometry, a Hurwitz surface, named after Adolf Hurwitz, is a compact Riemann surface with preciselyautomorphisms, where g is the genus of the surface. This number is maximal by virtue of Hurwitz's theorem on automorphisms...
s (the Riemann surfaces with maximal symmetry group), giving them a triangulation whose symmetry group equals their automorphism group as Riemann surfaces.

Klein quartic
In hyperbolic geometry, the Klein quartic, named after Felix Klein, is a compact Riemann surface of genus 3 with the highest possible order automorphism group for this genus, namely order 168 orientation-preserving automorphisms, and 336 automorphisms if orientation may be reversed...
, the most symmetric genus 3 surface, together with a tiling by 56 triangles, meeting at 24 vertices, with symmetry group the simple group of order 168, known as PSL(2,7)
PSL(2,7)
In mathematics, the projective special linear group PSL is a finite simple group that has important applications in algebra, geometry, and number theory. It is the automorphism group of the Klein quartic as well as the symmetry group of the Fano plane...
. The resulting surface can in turn be polyhedrally immersed into Euclidean 3-space, yielding the small cubicuboctahedron
Small cubicuboctahedron
In geometry, the small cubicuboctahedron is a uniform star polyhedron, indexed as U13. It has 20 faces , 48 edges, and 24 vertices. Its vertex figure is a crossed quadrilateral.- Related polyhedra :...
.
The dual order-3 heptagonal tiling has the same symmetry group, and thus yields heptagonal tilings of Hurwitz surfaces.
See also
- List of regular polytopes
- List of uniform planar tilings
- Tilings of regular polygons
- Triangular tiling
- Uniform tilings in hyperbolic planeUniform tilings in hyperbolic planeThere are an infinite number of uniform tilings on the hyperbolic plane based on the where 1/p + 1/q + 1/r ...

