Octeract - AbsoluteAstronomy.com

8-cube Octeract
Orthogonal projection inside Petrie polygon Petrie polygon In geometry, a Petrie polygon for a regular polytope of n dimensions is a skew polygon such that every consecutive sides belong to one of the facets...
Type	Regular 8-polytope 8-polytope In eight-dimensional geometry, a polyzetton is a polytope contained by 7-polytope facets. Each 6-polytope ridge being shared by exactly two 7-polytope facets....
Family	hypercube Hypercube In geometry, a hypercube is an n-dimensional analogue of a square and a cube . It is a closed, compact, convex figure whose 1-skeleton consists of groups of opposite parallel line segments aligned in each of the space's dimensions, perpendicular to each other and of the same length.An...
Schläfli symbol	{4,3⁶}
Coxeter-Dynkin diagram Coxeter-Dynkin diagram In geometry, a Coxeter–Dynkin diagram is a graph with numerically labeled edges representing the spatial relations between a collection of mirrors...
7-faces	16 {4,3⁵} Hepteract In geometry, a 7-cube is a seven-dimensional hypercube with 128 vertices, 448 edges, 672 square faces, 560 cubic cells, 280 tesseract 4-faces, 84 penteract 5-faces, and 14 hexeract 6-faces....
6-faces	112 {4,3⁴} Hexeract In geometry, a 6-cube is a six-dimensional hypercube with 64 vertices, 192 edges, 240 square faces, 160 cubic cells, 60 tesseract 4-faces, and 12 5-cube 5-faces....
5-faces	448 {4,3³} Penteract In five dimensional geometry, a 5-cube is a name for a five dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract hypercells....
4-faces	1120 {4,3²} Tesseract In geometry, the tesseract, also called an 8-cell or regular octachoron or cubic prism, is the four-dimensional analog of the cube. The tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of 6 square faces, the hypersurface of the tesseract consists of 8...
Cells	1792 {4,3} Cube In geometry, a cube is a three-dimensional solid object bounded by six square faces, facets or sides, with three meeting at each vertex. The cube can also be called a regular hexahedron and is one of the five Platonic solids. It is a special kind of square prism, of rectangular parallelepiped and...
Faces	1792 {4} Square (geometry) In geometry, a square is a regular quadrilateral. This means that it has four equal sides and four equal angles...
Edges	1024
Vertices	256
Vertex figure Vertex figure In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...	7-simplex
Petrie polygon Petrie polygon In geometry, a Petrie polygon for a regular polytope of n dimensions is a skew polygon such that every consecutive sides belong to one of the facets...	hexadecagon Hexadecagon In mathematics, a hexadecagon is a polygon with 16 sides and 16 vertices.- Regular hexadecagon :A regular hexadecagon is constructible with a compass and straightedge....
Coxeter group Coxeter group In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example...	C₈, [3⁶,4]
Dual	8-orthoplex
Properties	convex Convex polytope A convex polytope is a special case of a polytope, having the additional property that it is also a convex set of points in the n-dimensional space Rn...

In geometry

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

, an 8-cube is an eight-dimension

Dimension

In physics and mathematics, the dimension of a space or object is informally defined as the minimum number of coordinates needed to specify any point within it. Thus a line has a dimension of one because only one coordinate is needed to specify a point on it...

al hypercube

Hypercube

In geometry, a hypercube is an n-dimensional analogue of a square and a cube . It is a closed, compact, convex figure whose 1-skeleton consists of groups of opposite parallel line segments aligned in each of the space's dimensions, perpendicular to each other and of the same length.An...

(8-cube). It has 256 vertices

Vertex (geometry)

In geometry, a vertex is a special kind of point that describes the corners or intersections of geometric shapes.-Of an angle:...

, 1024 edge

Edge (geometry)

In geometry, an edge is a one-dimensional line segment joining two adjacent zero-dimensional vertices in a polygon. Thus applied, an edge is a connector for a one-dimensional line segment and two zero-dimensional objects....

s, 1792 square faces

Face (geometry)

In geometry, a face of a polyhedron is any of the polygons that make up its boundaries. For example, any of the squares that bound a cube is a face of the cube...

, 1792 cubic cells, 1120 tesseract

Tesseract

In geometry, the tesseract, also called an 8-cell or regular octachoron or cubic prism, is the four-dimensional analog of the cube. The tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of 6 square faces, the hypersurface of the tesseract consists of 8...

4-faces

Hypercell

In geometry, a hypercell is a descriptive term for an element of a polytope or tessellation, usually representing an element one dimension higher than a cell. The most generally accepted term is 4-face because it contains a 4-dimensional interior...

, 448 5-cube 5-faces, 112 6-cube 6-faces, and 16 7-cube 7-faces.

It is represented by Schläfli symbol {4,3⁶}, being composed of 3 7-cubes around each 6-face. It is called an octeract, derived from combining the name tesseract

Tesseract

(the 4-cube) with oct for eight (dimensions) in Greek

Greek language

Greek is an independent branch of the Indo-European family of languages. Native to the southern Balkans, it has the longest documented history of any Indo-European language, spanning 34 centuries of written records. Its writing system has been the Greek alphabet for the majority of its history;...

. It can also be called a regular hexdeca-8-tope or hexadecazetton, being a 8 dimensional polytope

8-polytope

In eight-dimensional geometry, a polyzetton is a polytope contained by 7-polytope facets. Each 6-polytope ridge being shared by exactly two 7-polytope facets....

constructed from 16 regular facets.

Related polytopes

It is a part of an infinite family of polytopes, called hypercube

Hypercube

s. The dual of an 8-cube can be called a 8-orthoplex, and is a part of the infinite family of cross-polytope

Cross-polytope

In geometry, a cross-polytope, orthoplex, hyperoctahedron, or cocube is a regular, convex polytope that exists in any number of dimensions. The vertices of a cross-polytope are all the permutations of . The cross-polytope is the convex hull of its vertices...

Cartesian coordinates

Cartesian coordinates for the vertices of an 8-cube centered at the origin and edge length 2 are

(±1,±1,±1,±1,±1,±1,±1,±1)

while the interior of the same consists of all points (x₀, x₁, x₂, x₃, x₄, x₅, x₆, x₇) with -1 < x_i < 1.

Projections

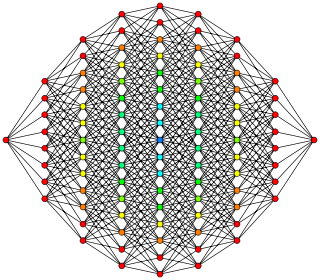This 8-cube graph is an orthogonal projection. This oriention shows columns of vertices positioned a vertex-edge-vertex distance from one vertex on the left to one vertex on the right, and edges attaching adjacent columns of vertices. The number of vertices in each column represents rows in Pascal's triangle Pascal's triangle In mathematics, Pascal's triangle is a triangular array of the binomial coefficients in a triangle. It is named after the French mathematician, Blaise Pascal... , being 1:8:28:56:70:56:28:8:1.

Derived polytopes

Applying an alternation operation, deleting alternating vertices of the hepteract, creates another uniform polytope

Uniform polytope

A uniform polytope is a vertex-transitive polytope made from uniform polytope facets of a lower dimension. Uniform polytopes of 2 dimensions are the regular polygons....

, called a 8-demicube, (part of an infinite family called demihypercubes), which has 16 demihepteract

Demihepteract

In geometry, a demihepteract or 7-demicube is a uniform 7-polytope, constructed from the 7-hypercube with alternated vertices deleted...

ic and 128 8-simplex facets.

External links

Multi-dimensional Glossary: hypercube Garrett Jones

The source of this article is wikipedia, the free encyclopedia. The text of this article is licensed under the GFDL.