Nash equilibrium
Encyclopedia
In game theory
, Nash equilibrium (named after John Forbes Nash, who proposed it) is a solution concept
of a game involving two or more players, in which each player is assumed to know the equilibrium strategies of the other players, and no player has anything to gain by changing only his own strategy unilaterally. If each player has chosen a strategy and no player can benefit by changing his or her strategy while the other players keep theirs unchanged, then the current set of strategy choices and the corresponding payoffs constitute a Nash equilibrium.
Stated simply, Amy and Phil are in Nash equilibrium if Amy is making the best decision she can, taking into account Phil's decision, and Phil is making the best decision he can, taking into account Amy's decision. Likewise, a group of players is in Nash equilibrium if each one is making the best decision that he or she can, taking into account the decisions of the others. However, Nash equilibrium does not necessarily mean the best payoff for all the players involved; in many cases, all the players might improve their payoffs if they could somehow agree on strategies different from the Nash equilibrium: e.g., competing businesses forming a cartel
in order to increase their profits.
of several decision makers
. In other words, it provides a way of predicting what will happen if several people or several institutions are making decisions at the same time, and if the outcome depends on the decisions of the others. The simple insight underlying John Nash's idea is that we cannot predict the result of the choices of multiple decision makers if we analyze those decisions in isolation. Instead, we must ask what each player would do, taking into account the decision-making of the others.
Nash equilibrium has been used to analyze hostile situations like war
and arms race
s (see Prisoner's dilemma
), and also how conflict may be mitigated by repeated interaction (see Tit-for-tat). It has also been used to study to what extent people with different preferences can cooperate (see Battle of the sexes
), and whether they will take risks to achieve a cooperative outcome (see Stag hunt
). It has been used to study the adoption of technical standards, and also the occurrence of bank run
s and currency crises (see Coordination game
). Other applications include traffic flow (see Wardrop's principle), how to organize auctions (see auction theory
), the outcome of efforts exerted by multiple parties in the education process, and even penalty kicks in soccer (see Matching pennies
).
in his theory of oligopoly (1838). In Cournot's theory, firms choose how much output to produce to maximize their own profit. However, the best output for one firm depends on the outputs of others. A Cournot equilibrium occurs when each firm's output maximizes its profits given the output of the other firms, which is a pure strategy Nash Equilibrium.
The modern game-theoretic concept of Nash Equilibrium is instead defined in terms of mixed strategies, where players choose a probability distribution over possible actions. The concept of the mixed strategy Nash Equilibrium was introduced by John von Neumann
and Oskar Morgenstern
in their 1944 book The Theory of Games and Economic Behavior. However, their analysis was restricted to the special case of zero-sum
games. They showed that a mixed-strategy Nash Equilibrium will exist for any zero-sum game with a finite set of actions. The contribution of John Forbes Nash
in his 1951 article Non-Cooperative Games was to define a mixed strategy Nash Equilibrium for any game with a finite set of actions and prove that at least one (mixed strategy) Nash Equilibrium must exist in such a game.
Since the development of the Nash equilibrium concept, game theorists have discovered that it makes misleading predictions (or fails to make a unique prediction) in certain circumstances. Therefore they have proposed many related solution concept
s (also called 'refinements' of Nash equilibrium) designed to overcome perceived flaws in the Nash concept. One particularly important issue is that some Nash equilibria may be based on threats that are not 'credible
'. Therefore, in 1965 Reinhard Selten
proposed subgame perfect equilibrium
as a refinement that eliminates equilibria which depend on non-credible threats. Other extensions of the Nash equilibrium concept have addressed what happens if a game is repeated
, or what happens if a game is played in the absence of perfect information
. However, subsequent refinements and extensions of the Nash equilibrium concept share the main insight on which Nash's concept rests: all equilibrium concepts analyze what choices will be made when each player takes into account the decision-making of others.
If any player would answer "Yes", then that set of strategies is not a Nash equilibrium. But if every player prefers not to switch (or is indifferent between switching and not) then the set of strategies is a Nash equilibrium. Thus, each strategy in a Nash equilibrium is a best response
to all other strategies in that equilibrium.
The Nash equilibrium may sometimes appear non-rational in a third-person perspective. This is because it may happen that a Nash equilibrium is not Pareto optimal
.
The Nash equilibrium may also have non-rational consequences in sequential games because players may "threaten" each other with non-rational moves. For such games the subgame perfect Nash equilibrium may be more meaningful as a tool of analysis.
s and f=(f1(x), ..., fn(x)) is the payoff function for x S. Let xi be a strategy profile of player i and x-i be a strategy profile of all players except for player i. When each player i
S. Let xi be a strategy profile of player i and x-i be a strategy profile of all players except for player i. When each player i 
{1, ..., n} chooses strategy xi resulting in strategy profile x = (x1, ..., xn) then player i obtains payoff fi(x). Note that the payoff depends on the strategy profile chosen, i.e., on the strategy chosen by player i as well as the strategies chosen by all the other players. A strategy profile x*  S is a Nash equilibrium (NE) if no unilateral deviation in strategy by any single player is profitable for that player, that is
S is a Nash equilibrium (NE) if no unilateral deviation in strategy by any single player is profitable for that player, that is
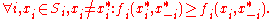
A game can have either a pure-strategy or a mixed Nash Equilibrium, (in the latter a pure strategy is chosen stochastic
ally with a fixed frequency). Nash proved that if we allow mixed strategies, then every game with a finite number of players in which each player can choose from finitely many pure strategies has at least one Nash equilibrium.
When the inequality above holds strictly (with instead of
instead of  ) for all players and all feasible alternative strategies, then the equilibrium is classified as a strict Nash equilibrium. If instead, for some player, there is exact equality between
) for all players and all feasible alternative strategies, then the equilibrium is classified as a strict Nash equilibrium. If instead, for some player, there is exact equality between 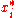 and some other strategy in the set
and some other strategy in the set 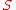 , then the equilibrium is classified as a weak Nash equilibrium.
, then the equilibrium is classified as a weak Nash equilibrium.
The coordination game is a classic (symmetric
) two player, two strategy
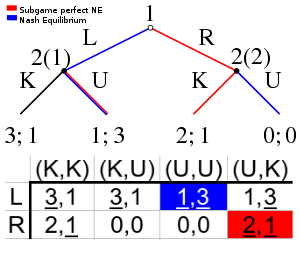
game, with an example payoff matrix shown to the right. The players should thus coordinate, both adopting strategy A, to receive the highest payoff; i.e., 4. If both players chose strategy B though, there is still a Nash equilibrium. Although each player is awarded less than optimal payoff, neither player has incentive to change strategy due to a reduction in the immediate payoff (from 3 to 1).
A famous example of this type of game was called the Stag Hunt
; in the game two players may choose to hunt a stag or a rabbit, the former providing more meat (4 utility units) than the latter (1 utility unit). The caveat is that the stag must be cooperatively hunted, so if one player attempts to hunt the stag, while the other hunts the rabbit, he will fail in hunting (0 utility units), whereas if they both hunt it they will split the payload (2, 2). The game hence exhibits two equilibrium at (stag, stag) and (rabbit, rabbit) and hence the players' optimal strategy depend on their expectation on what the other player may do. If one hunter trusts that the other will hunt the stag, he should hunt the stag; however if he suspects that the other will hunt the rabbit, he should hunt the rabbit. This game was used as an analogy for social cooperation, since much of the benefit that people gain in society depends upon people cooperating and implicitly trusting one another to act in a manner corresponding with cooperation.
Another example of a coordination game is the setting where two technologies are available to two firms with compatible products, and they have to elect a strategy to become the market standard. If both firms agree on the chosen technology, high sales are expected for both firms. If the firms do not agree on the standard technology, few sales result. Both strategies are Nash equilibria of the game.
Driving on a road, and having to choose either to drive on the left or to drive on the right of the road, is also a coordination game. For example, with payoffs 100 meaning no crash and 0 meaning a crash, the coordination game can be defined with the following payoff matrix:
In this case there are two pure strategy Nash equilibria, when both choose to either drive on the left or on the right. If we admit mixed strategies (where a pure strategy is chosen at random, subject to some fixed probability), then there are three Nash equilibria for the same case: two we have seen from the pure-strategy form, where the probabilities are (0%,100%) for player one, (0%, 100%) for player two; and (100%, 0%) for player one, (100%, 0%) for player two respectively. We add another where the probabilities for each player is (50%, 50%).
The Prisoner's Dilemma has the same payoff matrix as depicted for the Coordination Game, but now C > A > D > B. Because C > A and D > B, each player improves his situation by switching from strategy #1 to strategy #2, no matter what the other player decides. The Prisoner's Dilemma thus has a single Nash Equilibrium: both players choosing strategy #2 ("defect"). What has long made this an interesting case to study is the fact that D < A (i.e., "both defect" is globally inferior to "both remain loyal"). The globally optimal strategy is unstable; it is not an equilibrium.
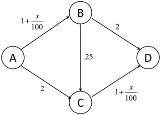
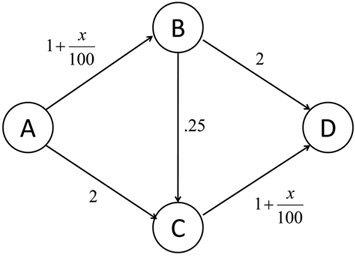 An application of Nash equilibria is in determining the expected flow of traffic in a network. Consider the graph on the right. If we assume that there are
An application of Nash equilibria is in determining the expected flow of traffic in a network. Consider the graph on the right. If we assume that there are  "cars" traveling from A to D, what is the expected distribution of traffic in the network?
"cars" traveling from A to D, what is the expected distribution of traffic in the network?
This situation can be modeled as a "game" where every traveler has a choice of 3 strategies, where each strategy is a route from A to D (either ,
,  , or
, or  ). The "payoff" of each strategy is the travel time of each route. In the graph on the right, a car travelling via
). The "payoff" of each strategy is the travel time of each route. In the graph on the right, a car travelling via  experiences travel time of
experiences travel time of 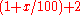 , where
, where  is the number of cars traveling on edge
is the number of cars traveling on edge  . Thus, payoffs for any given strategy depend on the choices of the other players, as is usual. However, the goal in this case is to minimize travel time, not maximize it. Equilibrium will occur when the time on all paths is exactly the same. When that happens, no single driver has any incentive to switch routes, since it can only add to his/her travel time. For the graph on the right, if, for example, 100 cars are travelling from A to D, then equilibrium will occur when 25 drivers travel via
. Thus, payoffs for any given strategy depend on the choices of the other players, as is usual. However, the goal in this case is to minimize travel time, not maximize it. Equilibrium will occur when the time on all paths is exactly the same. When that happens, no single driver has any incentive to switch routes, since it can only add to his/her travel time. For the graph on the right, if, for example, 100 cars are travelling from A to D, then equilibrium will occur when 25 drivers travel via  , 50 via
, 50 via  , and 25 via
, and 25 via  . Every driver now has a total travel time of 3.75.
. Every driver now has a total travel time of 3.75.
Notice that this distribution is not, actually, socially optimal. If the 100 cars agreed that 50 travel via and the other 50 through
and the other 50 through  , then travel time for any single car would actually be 3.5 which is less than 3.75. This is also the Nash equilibrium if the path between B and C is removed, which means that adding an additional possible route can decrease the efficiency of the system, a phenomenon known as Braess's Paradox.
, then travel time for any single car would actually be 3.5 which is less than 3.75. This is also the Nash equilibrium if the path between B and C is removed, which means that adding an additional possible route can decrease the efficiency of the system, a phenomenon known as Braess's Paradox.
This can be illustrated by a two-player game in which both players simultaneously choose an integer from 0 to 3 and they both win the smaller of the two numbers in points. In addition, if one player chooses a larger number than the other, then he/she has to give up two points to the other.
This game has a unique pure-strategy Nash equilibrium: both players choosing 0 (highlighted in light red). Any other choice of strategies can be improved if one of the players lowers his number to one less than the other player's number. In the table to the right, for example, when starting at the green square it is in player 1's interest to move to the purple square by choosing a smaller number, and it is in player 2's interest to move to the blue square by choosing a smaller number. If the game is modified so that the two players win the named amount if they both choose the same number, and otherwise win nothing, then there are 4 Nash equilibria (0,0...1,1...2,2...and 3,3).
We can apply this rule to a 3×3 matrix:
Using the rule, we can very quickly (much faster than with formal analysis) see that the Nash Equlibria cells are (B,A), (A,B), and (C,C). Indeed, for cell (B,A) 40 is the maximum of the first column and 25 is the maximum of the second row. For (A,B) 25 is the maximum of the second column and 40 is the maximum of the first row. Same for cell (C,C). For other cells, either one or both of the duplet members are not the maximum of the corresponding rows and columns.
This said, the actual mechanics of finding equilibrium cells is obvious: find the maximum of a column and check if the second member of the pair is the maximum of the row. If these conditions are met, the cell represents a Nash Equilibrium. Check all columns this way to find all NE cells. An N×N matrix may have between 0 and N×N pure strategy Nash equilibria.
, useful in the analysis of many kinds of equilibria, can also be applied to Nash equilibria.
A Nash equilibrium for a mixed strategy game is stable if a small change (specifically, an infinitesimal change) in probabilities for one player leads to a situation where two conditions hold:
If these cases are both met, then a player with the small change in his mixed-strategy will return immediately to the Nash equilibrium. The equilibrium is said to be stable. If condition one does not hold then the equilibrium is unstable. If only condition one holds then there are likely to be an infinite number of optimal strategies for the player who changed. John Nash
showed that the latter situation could not arise in a range of well-defined games.
In the "driving game" example above there are both stable and unstable equilibria. The equilibria involving mixed-strategies with 100% probabilities are stable. If either player changes his probabilities slightly, they will be both at a disadvantage, and his opponent will have no reason to change his strategy in turn. The (50%,50%) equilibrium is unstable. If either player changes his probabilities, then the other player immediately has a better strategy at either (0%, 100%) or (100%, 0%).
Stability is crucial in practical applications of Nash equilibria, since the mixed-strategy of each player is not perfectly known, but has to be inferred from statistical distribution of his actions in the game. In this case unstable equilibria are very unlikely to arise in practice, since any minute change in the proportions of each strategy seen will lead to a change in strategy and the breakdown of the equilibrium.
The Nash equilibrium defines stability only in terms of unilateral deviations. In cooperative games such a concept is not convincing enough. Strong Nash equilibrium
allows for deviations by every conceivable coalition. Formally, a Strong Nash equilibrium
is a Nash equilibrium in which no coalition, taking the actions of its complements as given, can cooperatively deviate in a way that benefits all of its members. However, the Strong Nash concept is sometimes perceived as too "strong" in that the environment allows for unlimited private communication. In fact, Strong Nash equilibrium has to be Pareto efficient. As a result of these requirements, Strong Nash almost never exists.
A refined Nash equilibrium known as coalition-proof Nash equilibrium
(CPNE) occurs when players cannot do better even if they are allowed to communicate and make "self-enforcing" agreement to deviate. Every correlated strategy supported by iterated strict dominance
and on the Pareto frontier is a CPNE. Further, it is possible for a game to have a Nash equilibrium that is resilient against coalitions less than a specified size, k. CPNE is related to the theory of the core
.
problems in which these conditions are not met:
and evolutionary biology, the NE has explanatory power. The payoff in economics is utility (or sometimes money), and in evolutionary biology gene transmission, both are the fundamental bottom line of survival. Researchers who apply games theory in these fields claim that strategies failing to maximize these for whatever reason will be competed out of the market or environment, which are ascribed the ability to test all strategies. This conclusion is drawn from the "stability" theory above. In these situations the assumption that the strategy observed is actually a NE has often been borne out by research.
 The Nash equilibrium is a superset of the subgame perfect Nash equilibrium. The subgame perfect equilibrium in addition to the Nash Equilibrium requires that the strategy also is a Nash equilibrium in every subgame of that game. This eliminates all non-credible threats, that is, strategies that contain non-rational moves in order to make the counter-player change his strategy.
The Nash equilibrium is a superset of the subgame perfect Nash equilibrium. The subgame perfect equilibrium in addition to the Nash Equilibrium requires that the strategy also is a Nash equilibrium in every subgame of that game. This eliminates all non-credible threats, that is, strategies that contain non-rational moves in order to make the counter-player change his strategy.
The image to the right shows a simple sequential game that illustrates the issue with subgame imperfect Nash equilibria. In this game player one chooses left(L) or right(R), which is followed by player two being called upon to be kind (K) or unkind (U) to player one, However, player two only stands to gain from being unkind if player one goes left. If player one goes right the rational player two would de facto be kind to him in that subgame. However, The non-credible threat of being unkind at 2(2) is still part of the blue (L, (U,U)) Nash equilibrium. Therefore, if rational behavior can be expected by both parties the subgame perfect Nash equilibrium may be a more meaningful solution concept when such dynamic inconsistencies
arise.
Proof using the Kakutani fixed point theorem
Nash's original proof (in his thesis) used Brouwer's fixed point theorem (e.g., see below for a variant). We give a simpler proof via the Kakutani fixed point theorem
, following Nash's 1950 paper (he credits David Gale
with the observation that such a simplification is possible).
To prove the existence of a Nash Equilibrium, let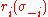 be the best response of player i to the strategies of all other players.
be the best response of player i to the strategies of all other players.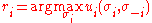
Here,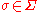 is a mixed strategy profile in the set of all mixed strategies and
is a mixed strategy profile in the set of all mixed strategies and 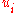 it the payoff function for player i. Define a function
it the payoff function for player i. Define a function 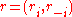 such that
such that  . The existence of a Nash Equilibrium is equivalent to
. The existence of a Nash Equilibrium is equivalent to  having a fixed point.
having a fixed point.
Kakutani's fixed point theorem guarantees the existence of a fixed point if the following four conditions are satisfied.
Condition 1. is satisfied from the fact that is a simplex and thus compact. Convexity follows from players' ability to mix strategies.
is a simplex and thus compact. Convexity follows from players' ability to mix strategies.  is nonempty as long as players have strategies.
is nonempty as long as players have strategies.
Condition 2. is satisfied because players maximize expected payoffs which is continuous function over a compact set. The Weierstrauss Extreme Value Theorem
guarantees that there is always a maximum value.
Condition 3. is satisfied as a result of mixed strategies. Suppose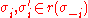 , then
, then 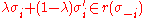 . i.e. if two strategies maximize payoffs, then a mix between the two strategies will yield the same payoff.
. i.e. if two strategies maximize payoffs, then a mix between the two strategies will yield the same payoff.
Condition 4. is satisfied by way of Berge's Maximum Theorem. Because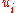 is continuos and compact,
is continuos and compact,  is upper hemicontinuous.
is upper hemicontinuous.
Therefore, there exists a fixed point in and a Nash Equilibrium.
and a Nash Equilibrium.
When Nash made this point to John von Neumann
in 1949, von Neumann famously dismissed it with the words, "That's trivial, you know. That's just a fixed point
theorem." (See Nasar, 1998, p. 94.)
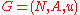 where
where  is the number of players and
is the number of players and
 is the
is the
action set for the players. All of the action sets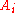 are finite. Let
are finite. Let
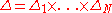 denote the set of mixed strategies
denote the set of mixed strategies
for the players. The finiteness of the s ensures the compactness of
s ensures the compactness of  .
.
We can now define the gain functions. For a mixed strategy , we let
, we let
the gain for player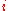 on action
on action  be
be
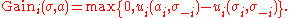
The gain function represents the benefit a player gets by unilaterally changing his strategy.
We now define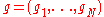 where
where
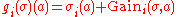
for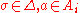 . We see that
. We see that
We now use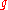 to define
to define 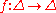 as follows.
as follows.
Let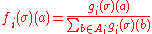
for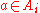 . It is easy to see that each
. It is easy to see that each 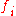 is a valid mixed strategy in
is a valid mixed strategy in 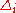 . It is also easy to check that each
. It is also easy to check that each 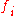 is a continuous function of
is a continuous function of  , and hence
, and hence  is a continuous function. Now
is a continuous function. Now  is the cross product of a finite number of compact convex sets, and so we get that
is the cross product of a finite number of compact convex sets, and so we get that  is also compact and convex. Therefore we may apply the Brouwer fixed point theorem to
is also compact and convex. Therefore we may apply the Brouwer fixed point theorem to  . So
. So  has a fixed point in
has a fixed point in  , call it
, call it  .
.
I claim that is a Nash Equilibrium in
is a Nash Equilibrium in  . For this purpose, it suffices to show that
. For this purpose, it suffices to show that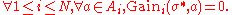
This simply states the each player gains no benefit by unilaterally changing his strategy which is exactly the
necessary condition for being a Nash Equilibrium.
Now assume that the gains are not all zero. Therefore, ,
, 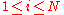 , and
, and
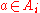 such that
such that 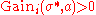 . Note then that
. Note then that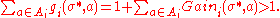
So let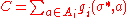 . Also we shall denote
. Also we shall denote  as the gain vector indexed by
as the gain vector indexed by
actions in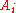 . Since
. Since  we clearly have that
we clearly have that 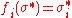 . Therefore we see that
. Therefore we see that

Since we have that
we have that 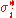 is some positive scaling of the vector
is some positive scaling of the vector 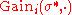 .
.
Now I claim that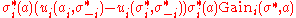
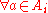 . To see this, we first note that if
. To see this, we first note that if 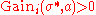 then this is true by definition of the
then this is true by definition of the
gain function. Now assume that . By our previous statements we have that
. By our previous statements we have that
and so the left term is zero, giving us that the entire expression is as needed.
as needed.
So we finally have that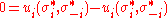
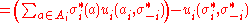
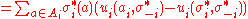
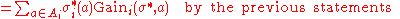
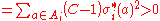
where the last inequality follows since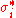 is a non-zero vector. But this is a clear contradiction,
is a non-zero vector. But this is a clear contradiction,
so all the gains must indeed be zero. Therefore is a Nash Equilibrium for
is a Nash Equilibrium for  as needed.
as needed.
 then there exists a Nash equilibrium in which A plays
then there exists a Nash equilibrium in which A plays  . In the case of two players A and B, there exists a Nash equilibrium in which A plays
. In the case of two players A and B, there exists a Nash equilibrium in which A plays  and B plays a best response
and B plays a best response
to . If
. If  is a strictly dominant strategy, A plays
is a strictly dominant strategy, A plays  in all Nash equilibria. If both A and B have strictly dominant strategies, there exists a unique Nash equilibrium in which each plays his strictly dominant strategy.
in all Nash equilibria. If both A and B have strictly dominant strategies, there exists a unique Nash equilibrium in which each plays his strictly dominant strategy.
In games with mixed strategy Nash equilibria, the probability of a player choosing any particular strategy can be computed by assigning a variable to each strategy that represents a fixed probability for choosing that strategy. In order for a player to be willing to randomize, his expected payoff for each strategy should be the same. In addition, the sum of the probabilities for each strategy of a particular player should be 1. This creates a system of equations from which the probabilities of choosing each strategy can be derived.
In the matching pennies game, player A loses a point to B if A and B play the same strategy and wins a point from B if they play different strategies. To compute the mixed strategy Nash equilibrium, assign A the probability p of playing H and (1−p) of playing T, and assign B the probability q of playing H and (1−q) of playing T.
Thus a mixed strategy Nash equilibrium in this game is for each player to randomly choose H or T with equal probability.
Game theory
Game theory is a mathematical method for analyzing calculated circumstances, such as in games, where a person’s success is based upon the choices of others...
, Nash equilibrium (named after John Forbes Nash, who proposed it) is a solution concept
Solution concept
In game theory, a solution concept is a formal rule for predicting how the game will be played. These predictions are called "solutions", and describe which strategies will be adopted by players, therefore predicting the result of the game...
of a game involving two or more players, in which each player is assumed to know the equilibrium strategies of the other players, and no player has anything to gain by changing only his own strategy unilaterally. If each player has chosen a strategy and no player can benefit by changing his or her strategy while the other players keep theirs unchanged, then the current set of strategy choices and the corresponding payoffs constitute a Nash equilibrium.
Stated simply, Amy and Phil are in Nash equilibrium if Amy is making the best decision she can, taking into account Phil's decision, and Phil is making the best decision he can, taking into account Amy's decision. Likewise, a group of players is in Nash equilibrium if each one is making the best decision that he or she can, taking into account the decisions of the others. However, Nash equilibrium does not necessarily mean the best payoff for all the players involved; in many cases, all the players might improve their payoffs if they could somehow agree on strategies different from the Nash equilibrium: e.g., competing businesses forming a cartel
Cartel
A cartel is a formal agreement among competing firms. It is a formal organization of producers and manufacturers that agree to fix prices, marketing, and production. Cartels usually occur in an oligopolistic industry, where there is a small number of sellers and usually involve homogeneous products...
in order to increase their profits.
Applications
Game theorists use the Nash equilibrium concept to analyze the outcome of the strategic interactionStrategy
Strategy, a word of military origin, refers to a plan of action designed to achieve a particular goal. In military usage strategy is distinct from tactics, which are concerned with the conduct of an engagement, while strategy is concerned with how different engagements are linked...
of several decision makers
Decision making
Decision making can be regarded as the mental processes resulting in the selection of a course of action among several alternative scenarios. Every decision making process produces a final choice. The output can be an action or an opinion of choice.- Overview :Human performance in decision terms...
. In other words, it provides a way of predicting what will happen if several people or several institutions are making decisions at the same time, and if the outcome depends on the decisions of the others. The simple insight underlying John Nash's idea is that we cannot predict the result of the choices of multiple decision makers if we analyze those decisions in isolation. Instead, we must ask what each player would do, taking into account the decision-making of the others.
Nash equilibrium has been used to analyze hostile situations like war
War
War is a state of organized, armed, and often prolonged conflict carried on between states, nations, or other parties typified by extreme aggression, social disruption, and usually high mortality. War should be understood as an actual, intentional and widespread armed conflict between political...
and arms race
Arms race
The term arms race, in its original usage, describes a competition between two or more parties for the best armed forces. Each party competes to produce larger numbers of weapons, greater armies, or superior military technology in a technological escalation...
s (see Prisoner's dilemma
Prisoner's dilemma
The prisoner’s dilemma is a canonical example of a game, analyzed in game theory that shows why two individuals might not cooperate, even if it appears that it is in their best interest to do so. It was originally framed by Merrill Flood and Melvin Dresher working at RAND in 1950. Albert W...
), and also how conflict may be mitigated by repeated interaction (see Tit-for-tat). It has also been used to study to what extent people with different preferences can cooperate (see Battle of the sexes
Battle of the sexes (game theory)
In game theory, battle of the sexes , also called Bach or Stravinsky, is a two-player coordination game. Imagine a couple that agreed to meet this evening, but cannot recall if they will be attending the opera or a football match. The husband would most of all like to go to the football game. The...
), and whether they will take risks to achieve a cooperative outcome (see Stag hunt
Stag hunt
In game theory, the stag hunt is a game which describes a conflict between safety and social cooperation. Other names for it or its variants include "assurance game", "coordination game", and "trust dilemma". Jean-Jacques Rousseau described a situation in which two individuals go out on a hunt. ...
). It has been used to study the adoption of technical standards, and also the occurrence of bank run
Bank run
A bank run occurs when a large number of bank customers withdraw their deposits because they believe the bank is, or might become, insolvent...
s and currency crises (see Coordination game
Coordination game
In game theory, coordination games are a class of games with multiple pure strategy Nash equilibria in which players choose the same or corresponding strategies...
). Other applications include traffic flow (see Wardrop's principle), how to organize auctions (see auction theory
Auction theory
Auction theory is an applied branch of economics which deals with how people act in auction markets and researches the properties of auction markets. There are many possible designs for an auction and typical issues studied by auction theorists include the efficiency of a given auction design,...
), the outcome of efforts exerted by multiple parties in the education process, and even penalty kicks in soccer (see Matching pennies
Matching pennies
Matching pennies is the name for a simple example game used in game theory. It is the two strategy equivalent of Rock, Paper, Scissors. Matching pennies is used primarily to illustrate the concept of mixed strategies and a mixed strategy Nash equilibrium....
).
History
A version of the Nash equilibrium concept was first used by Antoine Augustin CournotAntoine Augustin Cournot
Antoine Augustin Cournot was a French philosopher and mathematician.Antoine Augustin Cournot was born at Gray, Haute-Saone. In 1821 he entered one of the most prestigious Grande École, the École Normale Supérieure, and in 1829 he had earned a doctoral degree in mathematics, with mechanics as his...
in his theory of oligopoly (1838). In Cournot's theory, firms choose how much output to produce to maximize their own profit. However, the best output for one firm depends on the outputs of others. A Cournot equilibrium occurs when each firm's output maximizes its profits given the output of the other firms, which is a pure strategy Nash Equilibrium.
The modern game-theoretic concept of Nash Equilibrium is instead defined in terms of mixed strategies, where players choose a probability distribution over possible actions. The concept of the mixed strategy Nash Equilibrium was introduced by John von Neumann
John von Neumann
John von Neumann was a Hungarian-American mathematician and polymath who made major contributions to a vast number of fields, including set theory, functional analysis, quantum mechanics, ergodic theory, geometry, fluid dynamics, economics and game theory, computer science, numerical analysis,...
and Oskar Morgenstern
Oskar Morgenstern
Oskar Morgenstern was a German-born Austrian-School economist. He, along with John von Neumann, helped found the mathematical field of game theory ....
in their 1944 book The Theory of Games and Economic Behavior. However, their analysis was restricted to the special case of zero-sum
Zero-sum
In game theory and economic theory, a zero-sum game is a mathematical representation of a situation in which a participant's gain of utility is exactly balanced by the losses of the utility of other participant. If the total gains of the participants are added up, and the total losses are...
games. They showed that a mixed-strategy Nash Equilibrium will exist for any zero-sum game with a finite set of actions. The contribution of John Forbes Nash
John Forbes Nash
John Forbes Nash, Jr. is an American mathematician whose works in game theory, differential geometry, and partial differential equations have provided insight into the forces that govern chance and events inside complex systems in daily life...
in his 1951 article Non-Cooperative Games was to define a mixed strategy Nash Equilibrium for any game with a finite set of actions and prove that at least one (mixed strategy) Nash Equilibrium must exist in such a game.
Since the development of the Nash equilibrium concept, game theorists have discovered that it makes misleading predictions (or fails to make a unique prediction) in certain circumstances. Therefore they have proposed many related solution concept
Solution concept
In game theory, a solution concept is a formal rule for predicting how the game will be played. These predictions are called "solutions", and describe which strategies will be adopted by players, therefore predicting the result of the game...
s (also called 'refinements' of Nash equilibrium) designed to overcome perceived flaws in the Nash concept. One particularly important issue is that some Nash equilibria may be based on threats that are not 'credible
Credibility
Credibility refers to the objective and subjective components of the believability of a source or message.Traditionally, modern, credibility has two key components: trustworthiness and expertise, which both have objective and subjective components. Trustworthiness is based more on subjective...
'. Therefore, in 1965 Reinhard Selten
Reinhard Selten
-Life and career:Selten was born in Breslau in Lower Silesia, now in Poland, to a Jewish father, Adolf Selten, and Protestant mother, Käthe Luther. For his work in game theory, Selten won the 1994 Nobel Memorial Prize in Economic Sciences...
proposed subgame perfect equilibrium
Subgame perfect equilibrium
In game theory, a subgame perfect equilibrium is a refinement of a Nash equilibrium used in dynamic games. A strategy profile is a subgame perfect equilibrium if it represents a Nash equilibrium of every subgame of the original game...
as a refinement that eliminates equilibria which depend on non-credible threats. Other extensions of the Nash equilibrium concept have addressed what happens if a game is repeated
Repeated game
In game theory, a repeated game is an extensive form game which consists in some number of repetitions of some base game . The stage game is usually one of the well-studied 2-person games...
, or what happens if a game is played in the absence of perfect information
Global game
In economics and game theory, global games are games of incomplete information where players receive possibly-correlated signals of the underlying state of the world. Global games were originally defined by Carlsson and van Damme...
. However, subsequent refinements and extensions of the Nash equilibrium concept share the main insight on which Nash's concept rests: all equilibrium concepts analyze what choices will be made when each player takes into account the decision-making of others.
Informal definition
Informally, a set of strategies is a Nash equilibrium if no player can do better by unilaterally changing his or her strategy. To see what this means, imagine that each player is told the strategies of the others. Suppose then that each player asks himself or herself: "Knowing the strategies of the other players, and treating the strategies of the other players as set in stone, can I benefit by changing my strategy?"If any player would answer "Yes", then that set of strategies is not a Nash equilibrium. But if every player prefers not to switch (or is indifferent between switching and not) then the set of strategies is a Nash equilibrium. Thus, each strategy in a Nash equilibrium is a best response
Best response
In game theory, the best response is the strategy which produces the most favorable outcome for a player, taking other players' strategies as given...
to all other strategies in that equilibrium.
The Nash equilibrium may sometimes appear non-rational in a third-person perspective. This is because it may happen that a Nash equilibrium is not Pareto optimal
Pareto efficiency
Pareto efficiency, or Pareto optimality, is a concept in economics with applications in engineering and social sciences. The term is named after Vilfredo Pareto, an Italian economist who used the concept in his studies of economic efficiency and income distribution.Given an initial allocation of...
.
The Nash equilibrium may also have non-rational consequences in sequential games because players may "threaten" each other with non-rational moves. For such games the subgame perfect Nash equilibrium may be more meaningful as a tool of analysis.
Formal definition
Let (S, f) be a game with n players, where Si is the strategy set for player i, S=S1 X S2 ... X Sn is the set of strategy profileStrategy (game theory)
In game theory, a player's strategy in a game is a complete plan of action for whatever situation might arise; this fully determines the player's behaviour...
s and f=(f1(x), ..., fn(x)) is the payoff function for x
 S. Let xi be a strategy profile of player i and x-i be a strategy profile of all players except for player i. When each player i
S. Let xi be a strategy profile of player i and x-i be a strategy profile of all players except for player i. When each player i 
 S is a Nash equilibrium (NE) if no unilateral deviation in strategy by any single player is profitable for that player, that is
S is a Nash equilibrium (NE) if no unilateral deviation in strategy by any single player is profitable for that player, that is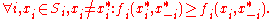
A game can have either a pure-strategy or a mixed Nash Equilibrium, (in the latter a pure strategy is chosen stochastic
Stochastic
Stochastic refers to systems whose behaviour is intrinsically non-deterministic. A stochastic process is one whose behavior is non-deterministic, in that a system's subsequent state is determined both by the process's predictable actions and by a random element. However, according to M. Kac and E...
ally with a fixed frequency). Nash proved that if we allow mixed strategies, then every game with a finite number of players in which each player can choose from finitely many pure strategies has at least one Nash equilibrium.
When the inequality above holds strictly (with
 instead of
instead of  ) for all players and all feasible alternative strategies, then the equilibrium is classified as a strict Nash equilibrium. If instead, for some player, there is exact equality between
) for all players and all feasible alternative strategies, then the equilibrium is classified as a strict Nash equilibrium. If instead, for some player, there is exact equality between 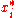 and some other strategy in the set
and some other strategy in the set 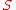 , then the equilibrium is classified as a weak Nash equilibrium.
, then the equilibrium is classified as a weak Nash equilibrium.Coordination game
| Player 2 adopts strategy A | Player 2 adopts strategy B | |
|---|---|---|
| Player 1 adopts strategy A | 4, 4 | 1, 3 |
| Player 1 adopts strategy B | 3, 1 | 3, 3 |
The coordination game is a classic (symmetric
Symmetric game
In game theory, a symmetric game is a game where the payoffs for playing a particular strategy depend only on the other strategies employed, not on who is playing them. If one can change the identities of the players without changing the payoff to the strategies, then a game is symmetric. ...
) two player, two strategy
Strategy (game theory)
In game theory, a player's strategy in a game is a complete plan of action for whatever situation might arise; this fully determines the player's behaviour...
game, with an example payoff matrix shown to the right. The players should thus coordinate, both adopting strategy A, to receive the highest payoff; i.e., 4. If both players chose strategy B though, there is still a Nash equilibrium. Although each player is awarded less than optimal payoff, neither player has incentive to change strategy due to a reduction in the immediate payoff (from 3 to 1).
A famous example of this type of game was called the Stag Hunt
Stag hunt
In game theory, the stag hunt is a game which describes a conflict between safety and social cooperation. Other names for it or its variants include "assurance game", "coordination game", and "trust dilemma". Jean-Jacques Rousseau described a situation in which two individuals go out on a hunt. ...
; in the game two players may choose to hunt a stag or a rabbit, the former providing more meat (4 utility units) than the latter (1 utility unit). The caveat is that the stag must be cooperatively hunted, so if one player attempts to hunt the stag, while the other hunts the rabbit, he will fail in hunting (0 utility units), whereas if they both hunt it they will split the payload (2, 2). The game hence exhibits two equilibrium at (stag, stag) and (rabbit, rabbit) and hence the players' optimal strategy depend on their expectation on what the other player may do. If one hunter trusts that the other will hunt the stag, he should hunt the stag; however if he suspects that the other will hunt the rabbit, he should hunt the rabbit. This game was used as an analogy for social cooperation, since much of the benefit that people gain in society depends upon people cooperating and implicitly trusting one another to act in a manner corresponding with cooperation.
Another example of a coordination game is the setting where two technologies are available to two firms with compatible products, and they have to elect a strategy to become the market standard. If both firms agree on the chosen technology, high sales are expected for both firms. If the firms do not agree on the standard technology, few sales result. Both strategies are Nash equilibria of the game.
Driving on a road, and having to choose either to drive on the left or to drive on the right of the road, is also a coordination game. For example, with payoffs 100 meaning no crash and 0 meaning a crash, the coordination game can be defined with the following payoff matrix:
| Drive on the Left | Drive on the Right | |
|---|---|---|
| Drive on the Left | 100, 100 | 0, 0 |
| Drive on the Right | 0, 0 | 100, 100 |
In this case there are two pure strategy Nash equilibria, when both choose to either drive on the left or on the right. If we admit mixed strategies (where a pure strategy is chosen at random, subject to some fixed probability), then there are three Nash equilibria for the same case: two we have seen from the pure-strategy form, where the probabilities are (0%,100%) for player one, (0%, 100%) for player two; and (100%, 0%) for player one, (100%, 0%) for player two respectively. We add another where the probabilities for each player is (50%, 50%).
Prisoner's dilemma
(note differences in the orientation of the payoff matrix)| Cooperate | Defect | |
|---|---|---|
| Cooperate | 3, 3 | 0, 5 |
| Defect | 5, 0 | 1, 1 |
The Prisoner's Dilemma has the same payoff matrix as depicted for the Coordination Game, but now C > A > D > B. Because C > A and D > B, each player improves his situation by switching from strategy #1 to strategy #2, no matter what the other player decides. The Prisoner's Dilemma thus has a single Nash Equilibrium: both players choosing strategy #2 ("defect"). What has long made this an interesting case to study is the fact that D < A (i.e., "both defect" is globally inferior to "both remain loyal"). The globally optimal strategy is unstable; it is not an equilibrium.
Network traffic

 "cars" traveling from A to D, what is the expected distribution of traffic in the network?
"cars" traveling from A to D, what is the expected distribution of traffic in the network?This situation can be modeled as a "game" where every traveler has a choice of 3 strategies, where each strategy is a route from A to D (either
 ,
,  , or
, or  ). The "payoff" of each strategy is the travel time of each route. In the graph on the right, a car travelling via
). The "payoff" of each strategy is the travel time of each route. In the graph on the right, a car travelling via  experiences travel time of
experiences travel time of 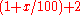 , where
, where  is the number of cars traveling on edge
is the number of cars traveling on edge  . Thus, payoffs for any given strategy depend on the choices of the other players, as is usual. However, the goal in this case is to minimize travel time, not maximize it. Equilibrium will occur when the time on all paths is exactly the same. When that happens, no single driver has any incentive to switch routes, since it can only add to his/her travel time. For the graph on the right, if, for example, 100 cars are travelling from A to D, then equilibrium will occur when 25 drivers travel via
. Thus, payoffs for any given strategy depend on the choices of the other players, as is usual. However, the goal in this case is to minimize travel time, not maximize it. Equilibrium will occur when the time on all paths is exactly the same. When that happens, no single driver has any incentive to switch routes, since it can only add to his/her travel time. For the graph on the right, if, for example, 100 cars are travelling from A to D, then equilibrium will occur when 25 drivers travel via  , 50 via
, 50 via  , and 25 via
, and 25 via  . Every driver now has a total travel time of 3.75.
. Every driver now has a total travel time of 3.75.Notice that this distribution is not, actually, socially optimal. If the 100 cars agreed that 50 travel via
 and the other 50 through
and the other 50 through  , then travel time for any single car would actually be 3.5 which is less than 3.75. This is also the Nash equilibrium if the path between B and C is removed, which means that adding an additional possible route can decrease the efficiency of the system, a phenomenon known as Braess's Paradox.
, then travel time for any single car would actually be 3.5 which is less than 3.75. This is also the Nash equilibrium if the path between B and C is removed, which means that adding an additional possible route can decrease the efficiency of the system, a phenomenon known as Braess's Paradox.Competition game
| Player 2 chooses '0' | Player 2 chooses '1' | Player 2 chooses '2' | Player 2 chooses '3' | |
|---|---|---|---|---|
| Player 1 chooses '0' | 0, 0 | 2, -2 | 2, -2 | 2, -2 |
| Player 1 chooses '1' | -2, 2 | 1, 1 | 3, -1 | 3, -1 |
| Player 1 chooses '2' | -2, 2 | -1, 3 | 2, 2 | 4, 0 |
| Player 1 chooses '3' | -2, 2 | -1, 3 | 0, 4 | 3, 3 |
This can be illustrated by a two-player game in which both players simultaneously choose an integer from 0 to 3 and they both win the smaller of the two numbers in points. In addition, if one player chooses a larger number than the other, then he/she has to give up two points to the other.
This game has a unique pure-strategy Nash equilibrium: both players choosing 0 (highlighted in light red). Any other choice of strategies can be improved if one of the players lowers his number to one less than the other player's number. In the table to the right, for example, when starting at the green square it is in player 1's interest to move to the purple square by choosing a smaller number, and it is in player 2's interest to move to the blue square by choosing a smaller number. If the game is modified so that the two players win the named amount if they both choose the same number, and otherwise win nothing, then there are 4 Nash equilibria (0,0...1,1...2,2...and 3,3).
Nash equilibria in a payoff matrix
There is an easy numerical way to identify Nash equilibria on a payoff matrix. It is especially helpful in two-person games where players have more than two strategies. In this case formal analysis may become too long. This rule does not apply to the case where mixed (stochastic) strategies are of interest. The rule goes as follows: if the first payoff number, in the duplet of the cell, is the maximum of the column of the cell and if the second number is the maximum of the row of the cell - then the cell represents a Nash equilibrium.| Option A | Option B | Option C | |
|---|---|---|---|
| Option A | 0, 0 | 25, 40 | 5, 10 |
| Option B | 40, 25 | 0, 0 | 5, 15 |
| Option C | 10, 5 | 15, 5 | 10, 10 |
We can apply this rule to a 3×3 matrix:
Using the rule, we can very quickly (much faster than with formal analysis) see that the Nash Equlibria cells are (B,A), (A,B), and (C,C). Indeed, for cell (B,A) 40 is the maximum of the first column and 25 is the maximum of the second row. For (A,B) 25 is the maximum of the second column and 40 is the maximum of the first row. Same for cell (C,C). For other cells, either one or both of the duplet members are not the maximum of the corresponding rows and columns.
This said, the actual mechanics of finding equilibrium cells is obvious: find the maximum of a column and check if the second member of the pair is the maximum of the row. If these conditions are met, the cell represents a Nash Equilibrium. Check all columns this way to find all NE cells. An N×N matrix may have between 0 and N×N pure strategy Nash equilibria.
Stability
The concept of stabilityStability theory
In mathematics, stability theory addresses the stability of solutions of differential equations and of trajectories of dynamical systems under small perturbations of initial conditions...
, useful in the analysis of many kinds of equilibria, can also be applied to Nash equilibria.
A Nash equilibrium for a mixed strategy game is stable if a small change (specifically, an infinitesimal change) in probabilities for one player leads to a situation where two conditions hold:
- the player who did not change has no better strategy in the new circumstance
- the player who did change is now playing with a strictly worse strategy.
If these cases are both met, then a player with the small change in his mixed-strategy will return immediately to the Nash equilibrium. The equilibrium is said to be stable. If condition one does not hold then the equilibrium is unstable. If only condition one holds then there are likely to be an infinite number of optimal strategies for the player who changed. John Nash
John Forbes Nash
John Forbes Nash, Jr. is an American mathematician whose works in game theory, differential geometry, and partial differential equations have provided insight into the forces that govern chance and events inside complex systems in daily life...
showed that the latter situation could not arise in a range of well-defined games.
In the "driving game" example above there are both stable and unstable equilibria. The equilibria involving mixed-strategies with 100% probabilities are stable. If either player changes his probabilities slightly, they will be both at a disadvantage, and his opponent will have no reason to change his strategy in turn. The (50%,50%) equilibrium is unstable. If either player changes his probabilities, then the other player immediately has a better strategy at either (0%, 100%) or (100%, 0%).
Stability is crucial in practical applications of Nash equilibria, since the mixed-strategy of each player is not perfectly known, but has to be inferred from statistical distribution of his actions in the game. In this case unstable equilibria are very unlikely to arise in practice, since any minute change in the proportions of each strategy seen will lead to a change in strategy and the breakdown of the equilibrium.
The Nash equilibrium defines stability only in terms of unilateral deviations. In cooperative games such a concept is not convincing enough. Strong Nash equilibrium
Strong Nash equilibrium
A strong Nash equilibrium is a Nash equilibrium in which no coalition, taking the actions of its complements as given, can cooperatively deviate in a way that benefits all of its members...
allows for deviations by every conceivable coalition. Formally, a Strong Nash equilibrium
Strong Nash equilibrium
A strong Nash equilibrium is a Nash equilibrium in which no coalition, taking the actions of its complements as given, can cooperatively deviate in a way that benefits all of its members...
is a Nash equilibrium in which no coalition, taking the actions of its complements as given, can cooperatively deviate in a way that benefits all of its members. However, the Strong Nash concept is sometimes perceived as too "strong" in that the environment allows for unlimited private communication. In fact, Strong Nash equilibrium has to be Pareto efficient. As a result of these requirements, Strong Nash almost never exists.
A refined Nash equilibrium known as coalition-proof Nash equilibrium
Coalition-Proof Nash Equilibrium
The concept of coalition-proof Nash equilibrium applies to certain "noncooperative" environments in which players can freely discuss their strategies but cannot make binding commitments .It emphasizes the immunization to deviations that are self-enforcing...
(CPNE) occurs when players cannot do better even if they are allowed to communicate and make "self-enforcing" agreement to deviate. Every correlated strategy supported by iterated strict dominance
Dominance (game theory)
In game theory, strategic dominance occurs when one strategy is better than another strategy for one player, no matter how that player's opponents may play...
and on the Pareto frontier is a CPNE. Further, it is possible for a game to have a Nash equilibrium that is resilient against coalitions less than a specified size, k. CPNE is related to the theory of the core
Core (economics)
The core is the set of feasible allocations that cannot be improved upon by a subset of the economy's consumers. A coalition is said to improve upon or block a feasible allocation if the members of that coalition are better off under another feasible allocation that is identical to the first...
.
Occurrence
If a game has a unique Nash equilibrium and is played among players under certain conditions, then the NE strategy set will be adopted. Sufficient conditions to guarantee that the Nash equilibrium is played are:- The players all will do their utmost to maximize their expected payoff as described by the game.
- The players are flawless in execution.
- The players have sufficient intelligence to deduce the solution.
- The players know the planned equilibrium strategy of all of the other players.
- The players believe that a deviation in their own strategy will not cause deviations by any other players.
- There is common knowledgeCommon knowledge (logic)Common knowledge is a special kind of knowledge for a group of agents. There is common knowledge of p in a group of agents G when all the agents in G know p, they all know that they know p, they all know that they all know that they know p, and so on ad infinitum.The concept was first introduced in...
that all players meet these conditions, including this one. So, not only must each player know the other players meet the conditions, but also they must know that they all know that they meet them, and know that they know that they know that they meet them, and so on.
Where the conditions are not met
Examples of game theoryGame theory
Game theory is a mathematical method for analyzing calculated circumstances, such as in games, where a person’s success is based upon the choices of others...
problems in which these conditions are not met:
- The first condition is not met if the game does not correctly describe the quantities a player wishes to maximize. In this case there is no particular reason for that player to adopt an equilibrium strategy. For instance, the prisoner’s dilemma is not a dilemma if either player is happy to be jailed indefinitely.
- Intentional or accidental imperfection in execution. For example, a computer capable of flawless logical play facing a second flawless computer will result in equilibrium. Introduction of imperfection will lead to its disruption either through loss to the player who makes the mistake, or through negation of the common knowledgeCommon knowledge (logic)Common knowledge is a special kind of knowledge for a group of agents. There is common knowledge of p in a group of agents G when all the agents in G know p, they all know that they know p, they all know that they all know that they know p, and so on ad infinitum.The concept was first introduced in...
criterion leading to possible victory for the player. (An example would be a player suddenly putting the car into reverse in the game of chicken, ensuring a no-loss no-win scenario). - In many cases, the third condition is not met because, even though the equilibrium must exist, it is unknown due to the complexity of the game, for instance in Chinese chess. Or, if known, it may not be known to all players, as when playing tic-tac-toeTic-tac-toeTic-tac-toe, also called wick wack woe and noughts and crosses , is a pencil-and-paper game for two players, X and O, who take turns marking the spaces in a 3×3 grid. The X player usually goes first...
with a small child who desperately wants to win (meeting the other criteria). - The criterion of common knowledge may not be met even if all players do, in fact, meet all the other criteria. Players wrongly distrusting each other's rationality may adopt counter-strategies to expected irrational play on their opponents’ behalf. This is a major consideration in “Chicken” or an arms raceArms raceThe term arms race, in its original usage, describes a competition between two or more parties for the best armed forces. Each party competes to produce larger numbers of weapons, greater armies, or superior military technology in a technological escalation...
, for example.
Where the conditions are met
Due to the limited conditions in which NE can actually be observed, they are rarely treated as a guide to day-to-day behaviour, or observed in practice in human negotiations. However, as a theoretical concept in economicsEconomics
Economics is the social science that analyzes the production, distribution, and consumption of goods and services. The term economics comes from the Ancient Greek from + , hence "rules of the house"...
and evolutionary biology, the NE has explanatory power. The payoff in economics is utility (or sometimes money), and in evolutionary biology gene transmission, both are the fundamental bottom line of survival. Researchers who apply games theory in these fields claim that strategies failing to maximize these for whatever reason will be competed out of the market or environment, which are ascribed the ability to test all strategies. This conclusion is drawn from the "stability" theory above. In these situations the assumption that the strategy observed is actually a NE has often been borne out by research.
NE and non-credible threats

The image to the right shows a simple sequential game that illustrates the issue with subgame imperfect Nash equilibria. In this game player one chooses left(L) or right(R), which is followed by player two being called upon to be kind (K) or unkind (U) to player one, However, player two only stands to gain from being unkind if player one goes left. If player one goes right the rational player two would de facto be kind to him in that subgame. However, The non-credible threat of being unkind at 2(2) is still part of the blue (L, (U,U)) Nash equilibrium. Therefore, if rational behavior can be expected by both parties the subgame perfect Nash equilibrium may be a more meaningful solution concept when such dynamic inconsistencies
Dynamic inconsistency
In economics, dynamic inconsistency, or time inconsistency, describes a situation where a decision-maker's preferences change over time in such a way that what is preferred at one point in time is inconsistent with what is preferred at another point in time...
arise.
Proof using the Kakutani fixed point theoremKakutani fixed point theoremIn mathematical analysis, the Kakutani fixed-point theorem is a fixed-point theorem for set-valued functions. It provides sufficient conditions for a set-valued function defined on a convex, compact subset of a Euclidean space to have a fixed point, i.e. a point which is mapped to a set containing...
Nash's original proof (in his thesis) used Brouwer's fixed point theorem (e.g., see below for a variant). We give a simpler proof via the Kakutani fixed point theoremKakutani fixed point theorem
In mathematical analysis, the Kakutani fixed-point theorem is a fixed-point theorem for set-valued functions. It provides sufficient conditions for a set-valued function defined on a convex, compact subset of a Euclidean space to have a fixed point, i.e. a point which is mapped to a set containing...
, following Nash's 1950 paper (he credits David Gale
David Gale
David Gale was a distinguished American mathematician and economist. He was a Professor Emeritus at University of California, Berkeley, affiliated with departments of Mathematics, Economics, and Industrial Engineering and Operations Research...
with the observation that such a simplification is possible).
To prove the existence of a Nash Equilibrium, let
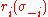 be the best response of player i to the strategies of all other players.
be the best response of player i to the strategies of all other players.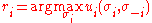
Here,
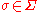 is a mixed strategy profile in the set of all mixed strategies and
is a mixed strategy profile in the set of all mixed strategies and 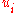 it the payoff function for player i. Define a function
it the payoff function for player i. Define a function 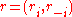 such that
such that  . The existence of a Nash Equilibrium is equivalent to
. The existence of a Nash Equilibrium is equivalent to  having a fixed point.
having a fixed point.Kakutani's fixed point theorem guarantees the existence of a fixed point if the following four conditions are satisfied.
-
 is compact, convex, and nonempty.
is compact, convex, and nonempty. -
 is nonempty.
is nonempty. -
 is convex.
is convex.  is upper hemicontinuous
is upper hemicontinuous
Condition 1. is satisfied from the fact that
 is a simplex and thus compact. Convexity follows from players' ability to mix strategies.
is a simplex and thus compact. Convexity follows from players' ability to mix strategies.  is nonempty as long as players have strategies.
is nonempty as long as players have strategies.Condition 2. is satisfied because players maximize expected payoffs which is continuous function over a compact set. The Weierstrauss Extreme Value Theorem
Extreme value theorem
In calculus, the extreme value theorem states that if a real-valued function f is continuous in the closed and bounded interval [a,b], then f must attain its maximum and minimum value, each at least once...
guarantees that there is always a maximum value.
Condition 3. is satisfied as a result of mixed strategies. Suppose
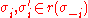 , then
, then 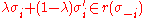 . i.e. if two strategies maximize payoffs, then a mix between the two strategies will yield the same payoff.
. i.e. if two strategies maximize payoffs, then a mix between the two strategies will yield the same payoff.Condition 4. is satisfied by way of Berge's Maximum Theorem. Because
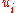 is continuos and compact,
is continuos and compact,  is upper hemicontinuous.
is upper hemicontinuous.Therefore, there exists a fixed point in
 and a Nash Equilibrium.
and a Nash Equilibrium.When Nash made this point to John von Neumann
John von Neumann
John von Neumann was a Hungarian-American mathematician and polymath who made major contributions to a vast number of fields, including set theory, functional analysis, quantum mechanics, ergodic theory, geometry, fluid dynamics, economics and game theory, computer science, numerical analysis,...
in 1949, von Neumann famously dismissed it with the words, "That's trivial, you know. That's just a fixed point
Fixed point (mathematics)
In mathematics, a fixed point of a function is a point that is mapped to itself by the function. A set of fixed points is sometimes called a fixed set...
theorem." (See Nasar, 1998, p. 94.)
Alternate proof using the Brouwer fixed-point theorem
We have a game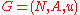 where
where  is the number of players and
is the number of players and is the
is theaction set for the players. All of the action sets
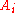 are finite. Let
are finite. Let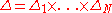 denote the set of mixed strategies
denote the set of mixed strategiesfor the players. The finiteness of the
 s ensures the compactness of
s ensures the compactness of  .
.We can now define the gain functions. For a mixed strategy
 , we let
, we letthe gain for player
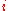 on action
on action  be
be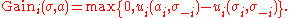
The gain function represents the benefit a player gets by unilaterally changing his strategy.
We now define
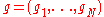 where
where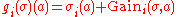
for
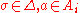 . We see that
. We see that
We now use
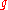 to define
to define 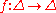 as follows.
as follows.Let
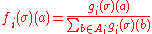
for
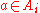 . It is easy to see that each
. It is easy to see that each 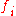 is a valid mixed strategy in
is a valid mixed strategy in 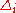 . It is also easy to check that each
. It is also easy to check that each 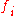 is a continuous function of
is a continuous function of  , and hence
, and hence  is a continuous function. Now
is a continuous function. Now  is the cross product of a finite number of compact convex sets, and so we get that
is the cross product of a finite number of compact convex sets, and so we get that  is also compact and convex. Therefore we may apply the Brouwer fixed point theorem to
is also compact and convex. Therefore we may apply the Brouwer fixed point theorem to  . So
. So  has a fixed point in
has a fixed point in  , call it
, call it  .
.I claim that
 is a Nash Equilibrium in
is a Nash Equilibrium in  . For this purpose, it suffices to show that
. For this purpose, it suffices to show that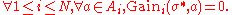
This simply states the each player gains no benefit by unilaterally changing his strategy which is exactly the
necessary condition for being a Nash Equilibrium.
Now assume that the gains are not all zero. Therefore,
 ,
, 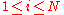 , and
, and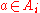 such that
such that 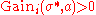 . Note then that
. Note then that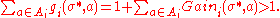
So let
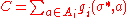 . Also we shall denote
. Also we shall denote  as the gain vector indexed by
as the gain vector indexed byactions in
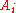 . Since
. Since  we clearly have that
we clearly have that 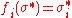 . Therefore we see that
. Therefore we see that

Since
 we have that
we have that 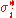 is some positive scaling of the vector
is some positive scaling of the vector 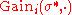 .
.Now I claim that
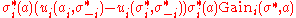
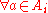 . To see this, we first note that if
. To see this, we first note that if 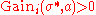 then this is true by definition of the
then this is true by definition of thegain function. Now assume that
 . By our previous statements we have that
. By our previous statements we have that
and so the left term is zero, giving us that the entire expression is
 as needed.
as needed.So we finally have that
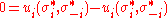
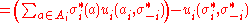
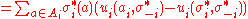
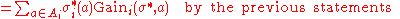
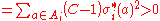
where the last inequality follows since
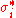 is a non-zero vector. But this is a clear contradiction,
is a non-zero vector. But this is a clear contradiction,so all the gains must indeed be zero. Therefore
 is a Nash Equilibrium for
is a Nash Equilibrium for  as needed.
as needed.Computing Nash equilibria
If a player A has a dominant strategy then there exists a Nash equilibrium in which A plays
then there exists a Nash equilibrium in which A plays  . In the case of two players A and B, there exists a Nash equilibrium in which A plays
. In the case of two players A and B, there exists a Nash equilibrium in which A plays  and B plays a best response
and B plays a best responseBest response
In game theory, the best response is the strategy which produces the most favorable outcome for a player, taking other players' strategies as given...
to
 . If
. If  is a strictly dominant strategy, A plays
is a strictly dominant strategy, A plays  in all Nash equilibria. If both A and B have strictly dominant strategies, there exists a unique Nash equilibrium in which each plays his strictly dominant strategy.
in all Nash equilibria. If both A and B have strictly dominant strategies, there exists a unique Nash equilibrium in which each plays his strictly dominant strategy.In games with mixed strategy Nash equilibria, the probability of a player choosing any particular strategy can be computed by assigning a variable to each strategy that represents a fixed probability for choosing that strategy. In order for a player to be willing to randomize, his expected payoff for each strategy should be the same. In addition, the sum of the probabilities for each strategy of a particular player should be 1. This creates a system of equations from which the probabilities of choosing each strategy can be derived.
Examples
| Player B plays H | Player B plays T | |
|---|---|---|
| Player A plays H | −1, +1 | +1, −1 |
| Player A plays T | +1, −1 | −1, +1 |
In the matching pennies game, player A loses a point to B if A and B play the same strategy and wins a point from B if they play different strategies. To compute the mixed strategy Nash equilibrium, assign A the probability p of playing H and (1−p) of playing T, and assign B the probability q of playing H and (1−q) of playing T.
- E[payoff for A playing H] = (−1)q + (+1)(1−q) = 1−2q
- E[payoff for A playing T] = (+1)q + (−1)(1−q) = 2q−1
- E[payoff for A playing H] = E[payoff for A playing T] ⇒ 1−2q = 2q−1 ⇒ q = 1/2
- E[payoff for B playing H] = (+1)p + (−1)(1−p) = 2p−1
- E[payoff for B playing T] = (−1)p + (+1)(1−p) = 1−2p
- E[payoff for B playing H] = E[payoff for B playing T] ⇒ 2p−1 = 1−2p ⇒ p = 1/2
Thus a mixed strategy Nash equilibrium in this game is for each player to randomly choose H or T with equal probability.
See also
- Adjusted winner procedureAdjusted Winner procedureIn problems of fair division, the adjusted winner procedure is used to partition a bundle of goods between two players in such a way as to minimize envy and maximize efficiency and equitability...
- Best responseBest responseIn game theory, the best response is the strategy which produces the most favorable outcome for a player, taking other players' strategies as given...
- Braess's paradox
- Complementarity theoryComplementarity theoryA complementarity problem is a type of mathematical optimization problem. It is the problem of optimizing a function of two vector variables subject to certain requirements which include: that the inner product of the two variables must equal zero, i.e. = 0...
- Conflict resolution researchConflict resolution researchConflict resolution is any reduction in the severity of a conflict. It may involve conflict management, in which the parties continue the conflict but adopt less extreme tactics; settlement, in which they reach agreement on enough issues that the conflict stops; or removal of the underlying causes...
- CooperationCooperationCooperation or co-operation is the process of working or acting together. In its simplest form it involves things working in harmony, side by side, while in its more complicated forms, it can involve something as complex as the inner workings of a human being or even the social patterns of a...
- Evolutionarily stable strategyEvolutionarily stable strategyIn game theory and behavioural ecology, an evolutionarily stable strategy , which is sometimes also called an evolutionary stable strategy, is a strategy which, if adopted by a population of players, cannot be invaded by any alternative strategy that is initially rare. An ESS is an equilibrium...
- Game theoryGame theoryGame theory is a mathematical method for analyzing calculated circumstances, such as in games, where a person’s success is based upon the choices of others...
- Glossary of game theory
- Hotelling's lawHotelling's lawHotelling's law is an observation in economics that in many markets it is rational for producers to make their products as similar as possible. This is also referred to as the principle of minimum differentiation as well as Hotelling's "linear city model"...
- Mexican StandoffMexican standoffA Mexican standoff is a slang term defined as a stalemate or impasse; a confrontation that neither side can foreseeably win. The term is most often used in lieu of "stalemate" when the confrontational situation is exceptionally dangerous for all parties involved.In popular culture, the Mexican...
- Minimax theorem
- Optimum contract and par contractOptimum contract and par contractOptimum contract and par contract are two closely related terms in the card game contract bridge:-Optimum contract:The optimum contract is that contract which offers the best chances, in unopposed bidding, of gaining bonus points for part-score, game or slam whilst minimising the risk of failure...
- Prisoner's dilemmaPrisoner's dilemmaThe prisoner’s dilemma is a canonical example of a game, analyzed in game theory that shows why two individuals might not cooperate, even if it appears that it is in their best interest to do so. It was originally framed by Merrill Flood and Melvin Dresher working at RAND in 1950. Albert W...
- Relations between equilibrium concepts
- Self-confirming equilibriumSelf-confirming equilibriumIn game theory, self-confirming equilibrium is a generalization of Nash equilibrium for extensive form games, in which players correctly predict the moves their opponents actually make, but may have misconceptions about what their opponents would do at information sets that are never reached when...
- Solution conceptSolution conceptIn game theory, a solution concept is a formal rule for predicting how the game will be played. These predictions are called "solutions", and describe which strategies will be adopted by players, therefore predicting the result of the game...
- Equilibrium selectionEquilibrium selectionEquilibrium selection is a concept from game theory which seeks to address reasons for players of a game to select a certain equilibrium over another...
- Stackelberg competitionStackelberg competitionThe Stackelberg leadership model is a strategic game in economics in which the leader firm moves first and then the follower firms move sequentially...
- Subgame perfect Nash equilibrium
- Wardrop's principle
Game theory textbooks
- Dixit, Avinash and Susan Skeath. Games of Strategy. W.W. Norton & Company. (Second edition in 2004). Suitable for undergraduate and business students.
- Fudenberg, Drew and Jean TiroleJean TiroleJean Marcel Tirole is a French professor of economics. He works on industrial organization, game theory, banking and finance, and economics and psychology. Tirole is director of the Jean-Jacques Laffont Foundation at the Toulouse School of Economics, and scientific director of the Industrial...
(1991) Game Theory MIT Press.. An 88-page mathematical introduction; see Chapter 2. Free online at many universities. - Morgenstern, OskarOskar MorgensternOskar Morgenstern was a German-born Austrian-School economist. He, along with John von Neumann, helped found the mathematical field of game theory ....
and John von NeumannJohn von NeumannJohn von Neumann was a Hungarian-American mathematician and polymath who made major contributions to a vast number of fields, including set theory, functional analysis, quantum mechanics, ergodic theory, geometry, fluid dynamics, economics and game theory, computer science, numerical analysis,...
(1947) The Theory of Games and Economic Behavior Princeton University Press. A modern introduction at the graduate level.. A comprehensive reference from a computational perspective; see Chapter 3. Downloadable free online.. Lucid and detailed introduction to game theory in an explicitly economic context.. Introduction to Nash equilibrium.
Original Nash papers
- Nash, JohnJohn Forbes NashJohn Forbes Nash, Jr. is an American mathematician whose works in game theory, differential geometry, and partial differential equations have provided insight into the forces that govern chance and events inside complex systems in daily life...
(1950) "Equilibrium points in n-person games" Proceedings of the National Academy of SciencesProceedings of the National Academy of SciencesThe Proceedings of the National Academy of Sciences of the United States of America, usually referred to as PNAS, is the official journal of the United States National Academy of Sciences...
36(1):48-49. - Nash, JohnJohn Forbes NashJohn Forbes Nash, Jr. is an American mathematician whose works in game theory, differential geometry, and partial differential equations have provided insight into the forces that govern chance and events inside complex systems in daily life...
(1951) "Non-Cooperative Games" The Annals of Mathematics 54(2):286-295.
Other references
- Mehlmann, A. The Game's Afoot! Game Theory in Myth and Paradox, American Mathematical Society (2000).
- Nasar, Sylvia (1998), "A Beautiful Mind", Simon and Schuster, Inc.

