
Nagata–Smirnov metrization theorem
Encyclopedia
The Nagata–Smirnov metrization theorem in topology
characterizes when a topological space
is metrizable. The theorem states that a topological space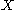 is metrizable if and only if it is regular
is metrizable if and only if it is regular
and Hausdorff
and has a countably locally finite (i.e., σ-locally finite) basis.
Unlike Urysohn's metrization theorem
, which provides a sufficient condition for metrization, this theorem provides both a necessary and sufficient condition for a topological space to be metrizable. The theorem is named after Junichi Nagata and Yuriĭ Mikhaĭlovich Smirnov.
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
characterizes when a topological space
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
is metrizable. The theorem states that a topological space
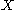 is metrizable if and only if it is regular
is metrizable if and only if it is regularRegular space
In topology and related fields of mathematics, a topological space X is called a regular space if every non-empty closed subset C of X and a point p not contained in C admit non-overlapping open neighborhoods. Thus p and C...
and Hausdorff
Hausdorff space
In topology and related branches of mathematics, a Hausdorff space, separated space or T2 space is a topological space in which distinct points have disjoint neighbourhoods. Of the many separation axioms that can be imposed on a topological space, the "Hausdorff condition" is the most frequently...
and has a countably locally finite (i.e., σ-locally finite) basis.
Unlike Urysohn's metrization theorem
Metrization theorem
In topology and related areas of mathematics, a metrizable space is a topological space that is homeomorphic to a metric space. That is, a topological space is said to be metrizable if there is a metricd\colon X \times X \to [0,\infty)...
, which provides a sufficient condition for metrization, this theorem provides both a necessary and sufficient condition for a topological space to be metrizable. The theorem is named after Junichi Nagata and Yuriĭ Mikhaĭlovich Smirnov.

