
Magnetosphere particle motion
Encyclopedia
Ion
An ion is an atom or molecule in which the total number of electrons is not equal to the total number of protons, giving it a net positive or negative electrical charge. The name was given by physicist Michael Faraday for the substances that allow a current to pass between electrodes in a...
s and electron
Electron
The electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
s of a plasma
Plasma (physics)
In physics and chemistry, plasma is a state of matter similar to gas in which a certain portion of the particles are ionized. Heating a gas may ionize its molecules or atoms , thus turning it into a plasma, which contains charged particles: positive ions and negative electrons or ions...
interacting with the Earth's magnetic field
Earth's magnetic field
Earth's magnetic field is the magnetic field that extends from the Earth's inner core to where it meets the solar wind, a stream of energetic particles emanating from the Sun...
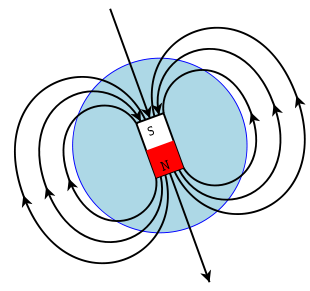
generally follow its magnetic field lines. These represent the force that a north magnetic pole would experience at any given point. (Denser lines indicate a stronger force.) Plasmas exhibit more complex second-order behaviors, studied as part of magnetohydrodynamics
Magnetohydrodynamics
Magnetohydrodynamics is an academic discipline which studies the dynamics of electrically conducting fluids. Examples of such fluids include plasmas, liquid metals, and salt water or electrolytes...
.
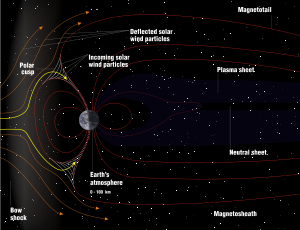
Magnetopause
The magnetopause is the abrupt boundary between a magnetosphere and the surrounding plasma. For planetary science, the magnetopause is the boundary between the planet’s magnetic field and the solar wind. The location of the magnetopause is determined by the balance between the pressure of the...
boundary between the magnetosphere and the solar wind
Solar wind
The solar wind is a stream of charged particles ejected from the upper atmosphere of the Sun. It mostly consists of electrons and protons with energies usually between 1.5 and 10 keV. The stream of particles varies in temperature and speed over time...
is outlined by field lines. Not much plasma can cross such a stiff boundary. Its only "weak points" are the two polar cusps, the points where field lines closing at noon (-z axis GSM) get separated from those closing at midnight (+z axis GSM); at such points the field intensity on the boundary is zero, posing no barrier to the entry of plasma. (This simple definition assumes a noon-midnight plane of symmetry, but closed fields lacking such symmetry also must have cusps, by the fixed point theorem.)
The amount of solar wind energy and plasma entering the actual magnetosphere depends on how far it departs from such a "closed" configuration, i.e. the extent to which Interplanetary Magnetic Field
Interplanetary Magnetic Field
The interplanetary magnetic field is the term for the solar magnetic field carried by the solar wind among the planets of the Solar System....
field lines manage to cross the boundary. As discussed further below, that extent depends very much on the direction of the Interplanetary Magnetic Field, in particular on its southward or northward slant.

Plasma (physics)
In physics and chemistry, plasma is a state of matter similar to gas in which a certain portion of the particles are ionized. Heating a gas may ionize its molecules or atoms , thus turning it into a plasma, which contains charged particles: positive ions and negative electrons or ions...
, e.g. of the ring current
Ring current
A ring current is an electric current carried by charged particles trapped in a planet's magnetosphere. It is caused by the longitudinal drift of energetic particles.-Earth's ring current:...
, also follows the structure of field lines. A particle interacting with this B field experiences a Lorentz Force
Lorentz force
In physics, the Lorentz force is the force on a point charge due to electromagnetic fields. It is given by the following equation in terms of the electric and magnetic fields:...
which is responsible for many of the particle motion in the magnetosphere. Furthermore, Birkeland currents and heat flow are also channeled by such lines — easy along them, blocked in perpendicular directions. Indeed, field lines in the magnetosphere have been likened to the grain in a log of wood, which defines an "easy" direction along which it easily gives way.
Motion of charged particles

Rg = mv/(qB)
If the initial velocity of the particle has a different direction, one only needs resolve it into a component v⊥perpendicular to B and a component v// parallel to B, and replace v in the above formula with v⊥.
If W⊥=m v⊥2/2 is the energy associated with the perpendicular motion in electron-volts (all calculations here are non-relativistic), in a field of B nT (nanotesla), then Rg in kilometers is
For protons Rg = (144/B) √W⊥
For electrons Rg = (3.37/B) √W⊥
The velocity parallel to the field v// is not affected by the field, because no magnetic force exists in that direction. That velocity just stays constant (as long as the field does!), and adding the two motions together gives a spiral around a central guiding field line. If the field curves or changes, the motion is modified, but the general character of spiraling around a central field line persists: hence the name "guiding center motion
Guiding center
In many cases of practical interest, the motion in a magnetic field of an electrically charged particle can be treated as the superposition of a relatively fast circular motion around a point called the guiding center and a relatively slow drift of this point...
."
Because the magnetic force is perpendicular to the velocity, it performs no work and requires no energy--nor does it provide any. Thus magnetic fields (like the Earth's) can profoundly affect particle motion in them, but need no energy input to maintain their effect. Particles may also get steered around, but their total energy remains the same.
Magnetic Mirroring and Magnetic Drift
The spacing between field lines is an indicator of the relative strength of the magnetic field. Where magnetic field lines converge the field grows stronger, and where they diverge, weaker.Now, it can be shown that in the motion of gyrating particles, the "magnetic moment" μ = W⊥/B (or relativistically, p⊥2/2B) stays very nearly constant. The "very nearly" qualifier sets it apart from true constants of motion, such as energy, reducing it to merely an "adiabatic invariant." For most plasmas in the magnetosphere, the deviation from constancy is negligible.
The conservation of μ is tremendously important (in laboratory plasmas as well as in space). Suppose the field line guiding a particle, the axis of its spiral path, belongs to a converging bundle of lines, so that the particle is led into an increasingly larger B. To keep μ constant, W⊥ must also grow.
However, as noted before, the total energy of a particle in a "purely magnetic" field remains constant. What therefore happens is that energy is converted, from the part associated with the parallel motion v// to the perpendicular part. As v// decreases, the angle between v and B then increases, until it reaches 90°. At that point W⊥ contains all the available energy, it can grow no more and no further advance into the stronger field can occur.
The result is known as magnetic mirror
Magnetic mirror
A magnetic mirror is a magnetic field configuration where the field strength changes when moving along a field line. The mirror effect results in a tendency for charged particles to bounce back from the high field region....
ing. The particle briefly gyrates perpendicular to its guiding field line, and then retreats back to the weaker field, the spiral unwinding again in the process. It may be noted that such motion was first derived by Henri Poincaré
Henri Poincaré
Jules Henri Poincaré was a French mathematician, theoretical physicist, engineer, and a philosopher of science...
in 1895, for a charged particle in the field of a magnetic monopole, whose field lines are all straight and converge to a point. The conservation of μ was only pointed by Alfvén
Hannes Alfvén
Hannes Olof Gösta Alfvén was a Swedish electrical engineer, plasma physicist and winner of the 1970 Nobel Prize in Physics for his work on magnetohydrodynamics . He described the class of MHD waves now known as Alfvén waves...
about 50 years later, and the connection to adiabatic invariant was only made afterwards.
Magnetic mirroring makes possible the "trapping" in the dipole-like field lines near Earth of particles in the radiation belt and in the ring current. On all such lines the field is much stronger at their ends near Earth, compared to its strength when it crosses the equatorial plane. Assuming such particles are somehow placed in the equatorial region of that field, most of them stay trapped, because every time their motion along the field line brings them into the strong field region, they "get mirrored" and bounce back and forth between hemispheres. Only particles whose motion is very close to parallel to the field line, with near-zero μ, avoid mirroring--and these are quickly absorbed by the atmosphere and lost. Their loss leaves a bundle of directions around the field line which is empty of particles--the "loss cone".
In addition to gyrating around their guiding field lines and bouncing back and forth between mirror points, trapped particles also drift slowly around Earth, switching guiding field lines but staying at approximately the same distance (another adiabatic invariant is involved, "the second invariant"). This motion
Guiding center
In many cases of practical interest, the motion in a magnetic field of an electrically charged particle can be treated as the superposition of a relatively fast circular motion around a point called the guiding center and a relatively slow drift of this point...
was mentioned earlier in connection with the ring current.
One reason for the drift is that the intensity of B increases as Earth is approached. The gyration around the guiding field line is therefore not a perfect circle, but curves a little more tightly on the side closer to the Earth, where the larger B gives a smaller Rg. This change in curvature makes ions advance sideways, while electrons, which gyrate in the opposite sense, advance sideways in the opposite direction. The net result, as already noted, produces the ring current, though additional effects (like non-uniform distribution of plasma density) also affect the result.
Plasma fountain
External links
- "3D Earth Magnetic Field Charged-Particle Simulator" Tool dedicated to the 3d simulation of charged particles in the magnetosphere.. [VRML Plug-in Required]

