
Incompressible surface
Encyclopedia
In mathematics
, an incompressible surface, in intuitive terms, is a surface
, embedded in a 3-manifold
, which has been simplified as much as possible while remaining "nontrivial" inside the 3-manifold.
For a precise definition, suppose that S is a compact surface properly embedded in a 3-manifold M. Suppose that D is a disk
, also embedded in M, with

Suppose finally that the curve in S does not bound a disk inside of S. Then D is called a compressing disk for S and we also call S a compressible surface in M. If no such disk exists and S is not the 2-sphere, then we call S incompressible (or geometrically incompressible).
in S does not bound a disk inside of S. Then D is called a compressing disk for S and we also call S a compressible surface in M. If no such disk exists and S is not the 2-sphere, then we call S incompressible (or geometrically incompressible).
Note that we must exclude the 2-sphere to get any interesting consequences for the 3-manifold. Every 3-manifold has many embedded 2-spheres, and a 2-sphere embedded in a 3-manifold never has a compressing disc.
Sometimes one defines an incompressible sphere to be a 2-sphere in a 3-manifold that does not bound a 3-ball. Thus, such a sphere either does not separate the 3-manifold or gives a nontrivial connected sum
decomposition. Since this notion of incompressibility for a sphere is quite different from the above definition for surfaces, often an incompressible sphere is instead referred to as an essential sphere or reducing sphere.
There is also an algebraic version of incompressibility: Suppose is a proper embedding of a compact surface. Then S is
is a proper embedding of a compact surface. Then S is  -injective (or algebraically incompressible) if the induced map on fundamental group
-injective (or algebraically incompressible) if the induced map on fundamental group
s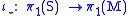
is injective.
In general, every -injective surface is incompressible, but the reverse implication is not always true. For instance, the Lens space
-injective surface is incompressible, but the reverse implication is not always true. For instance, the Lens space
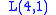 contains an incompressible Klein bottle that is not
contains an incompressible Klein bottle that is not  -injective. However, if
-injective. However, if  is a two-sided properly embedded, compact surface (not a 2-sphere), the loop theorem
is a two-sided properly embedded, compact surface (not a 2-sphere), the loop theorem
implies is incompressible if and only if it is
is incompressible if and only if it is  -injective.
-injective.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, an incompressible surface, in intuitive terms, is a surface
Surface
In mathematics, specifically in topology, a surface is a two-dimensional topological manifold. The most familiar examples are those that arise as the boundaries of solid objects in ordinary three-dimensional Euclidean space R3 — for example, the surface of a ball...
, embedded in a 3-manifold
3-manifold
In mathematics, a 3-manifold is a 3-dimensional manifold. The topological, piecewise-linear, and smooth categories are all equivalent in three dimensions, so little distinction is made in whether we are dealing with say, topological 3-manifolds, or smooth 3-manifolds.Phenomena in three dimensions...
, which has been simplified as much as possible while remaining "nontrivial" inside the 3-manifold.
For a precise definition, suppose that S is a compact surface properly embedded in a 3-manifold M. Suppose that D is a disk
Disk (mathematics)
In geometry, a disk is the region in a plane bounded by a circle.A disk is said to be closed or open according to whether or not it contains the circle that constitutes its boundary...
, also embedded in M, with

Suppose finally that the curve
 in S does not bound a disk inside of S. Then D is called a compressing disk for S and we also call S a compressible surface in M. If no such disk exists and S is not the 2-sphere, then we call S incompressible (or geometrically incompressible).
in S does not bound a disk inside of S. Then D is called a compressing disk for S and we also call S a compressible surface in M. If no such disk exists and S is not the 2-sphere, then we call S incompressible (or geometrically incompressible).Note that we must exclude the 2-sphere to get any interesting consequences for the 3-manifold. Every 3-manifold has many embedded 2-spheres, and a 2-sphere embedded in a 3-manifold never has a compressing disc.
Sometimes one defines an incompressible sphere to be a 2-sphere in a 3-manifold that does not bound a 3-ball. Thus, such a sphere either does not separate the 3-manifold or gives a nontrivial connected sum
Connected sum
In mathematics, specifically in topology, the operation of connected sum is a geometric modification on manifolds. Its effect is to join two given manifolds together near a chosen point on each...
decomposition. Since this notion of incompressibility for a sphere is quite different from the above definition for surfaces, often an incompressible sphere is instead referred to as an essential sphere or reducing sphere.
There is also an algebraic version of incompressibility: Suppose
 is a proper embedding of a compact surface. Then S is
is a proper embedding of a compact surface. Then S is  -injective (or algebraically incompressible) if the induced map on fundamental group
-injective (or algebraically incompressible) if the induced map on fundamental groupFundamental group
In mathematics, more specifically algebraic topology, the fundamental group is a group associated to any given pointed topological space that provides a way of determining when two paths, starting and ending at a fixed base point, can be continuously deformed into each other...
s
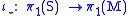
is injective.
In general, every
 -injective surface is incompressible, but the reverse implication is not always true. For instance, the Lens space
-injective surface is incompressible, but the reverse implication is not always true. For instance, the Lens spaceLens space
A lens space is an example of a topological space, considered in mathematics. The term often refers to a specific class of 3-manifolds, but in general can be defined for higher dimensions....
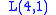 contains an incompressible Klein bottle that is not
contains an incompressible Klein bottle that is not  -injective. However, if
-injective. However, if  is a two-sided properly embedded, compact surface (not a 2-sphere), the loop theorem
is a two-sided properly embedded, compact surface (not a 2-sphere), the loop theoremLoop theorem
In mathematics, in the topology of 3-manifolds, the loop theorem is a generalization of Dehn's lemma. The loop theorem was first proven by Christos Papakyriakopoulos in 1956, along with Dehn's lemma and the Sphere theorem....
implies
 is incompressible if and only if it is
is incompressible if and only if it is  -injective.
-injective.See also
- Compression (3-manifold)
- Haken manifoldHaken manifoldIn mathematics, a Haken manifold is a compact, P²-irreducible 3-manifold that is sufficiently large, meaning that it contains a properly embedded two-sided incompressible surface...
- Virtually Haken conjectureVirtually Haken conjectureIn topology, an area of mathematics, the virtually Haken conjecture states that every compact, orientable, irreducible three-dimensional manifold with infinite fundamental group is virtually Haken...
- Thurston norm
- Boundary-incompressible surfaceBoundary-incompressible surfaceSuppose S is a compact surface with boundary that is properly embedded in a 3-manifold M with boundary. Suppose further that there exists a disk D in M such that D \cap S = \alpha and D \cap \partial M = \beta are arcs in \partial D , with \alpha \cup \beta = \partial D , \alpha \cap \beta...

