Imaginary time
Encyclopedia
Imaginary time is a concept derived from quantum mechanics
and is essential in connecting quantum mechanics with statistical mechanics.
is obtained from real time via a Wick rotation
by
in the complex plane: 
. It can be shown that at finite temperature T, the Green's function
s are periodic
in imaginary time with a period of
. Therefore their Fourier transform
s contain only a discrete set of frequencies called Matsubara frequencies. Another way to see the connection between statistical mechanics and quantum field theory is to consider the transition amplitude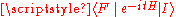
between an initial state I and a final state F. H is the Hamiltonian
of the system. If we compare this with the partition function
we see that to get the partition function
from the transition amplitudes we can replace
, set F = I = n and sum over n. This way we don't have to do twice the work by evaluating both the statistical properties and the transition amplitudes. Finally by using a Wick rotation one can show that the Euclidean quantum field theory
in (D + 1)-dimensional spacetime is nothing but quantum statistical mechanics
in D-dimensional space.
in physical cosmology
. Stephen Hawking
popularized the concept of imaginary time in his book A Brief History of Time
.
Imaginary time is difficult to visualize. If we imagine "regular time" as a horizontal line running between "past" in one direction and "future" in the other, then imaginary time would run perpendicular to this line as the imaginary number
s run perpendicular to the real number
s in the complex plane
. However, imaginary time is not imaginary in the sense that it is unreal or made-up — it simply runs in a direction different from the type of time we experience. In essence, imaginary time is a way of looking at the time dimension as if it were a dimension of space: you can move forward and backward along imaginary time, just like you can move right and left in space.
The concept is useful in cosmology because it can help smooth out gravitational singularities in models of the universe (see Hartle-Hawking state
). Singularities pose a problem for physicists because these are areas where known physical laws do not apply. The Big Bang
, for example, appears as a singularity in "regular time." But when visualized with imaginary time, the singularity is removed and the Big Bang functions like any other point in spacetime
.
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
and is essential in connecting quantum mechanics with statistical mechanics.
In quantum mechanics
Imaginary time is obtained from real time via a Wick rotationWick rotation
In physics, Wick rotation, named after Gian-Carlo Wick, is a method of finding a solution to a mathematical problem in Minkowski space from a solution to a related problem in Euclidean space by means of a transformation that substitutes an imaginary-number variable for a real-number variable...
by
in the complex plane: . It can be shown that at finite temperature T, the Green's functionGreen's function
In mathematics, a Green's function is a type of function used to solve inhomogeneous differential equations subject to specific initial conditions or boundary conditions...
s are periodic
Periodic function
In mathematics, a periodic function is a function that repeats its values in regular intervals or periods. The most important examples are the trigonometric functions, which repeat over intervals of length 2π radians. Periodic functions are used throughout science to describe oscillations,...
in imaginary time with a period of
. Therefore their Fourier transformFourier transform
In mathematics, Fourier analysis is a subject area which grew from the study of Fourier series. The subject began with the study of the way general functions may be represented by sums of simpler trigonometric functions...
s contain only a discrete set of frequencies called Matsubara frequencies. Another way to see the connection between statistical mechanics and quantum field theory is to consider the transition amplitude
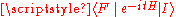between an initial state I and a final state F. H is the HamiltonianHamiltonian (quantum mechanics)
In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...
of the system. If we compare this with the partition function
we see that to get the partition functionPartition function (quantum field theory)
In quantum field theory, we have a generating functional, Z[J] of correlation functions and this value, called the partition function is usually expressed by something like the following functional integral:...
from the transition amplitudes we can replace
, set F = I = n and sum over n. This way we don't have to do twice the work by evaluating both the statistical properties and the transition amplitudes. Finally by using a Wick rotation one can show that the Euclidean quantum field theoryQuantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
in (D + 1)-dimensional spacetime is nothing but quantum statistical mechanics
Quantum statistical mechanics
Quantum statistical mechanics is the study of statistical ensembles of quantum mechanical systems. A statistical ensemble is described by a density operator S, which is a non-negative, self-adjoint, trace-class operator of trace 1 on the Hilbert space H describing the quantum system. This can be...
in D-dimensional space.
In cosmology
Imaginary time is also used in cosmology. It is used to describe models of the universeUniverse
The Universe is commonly defined as the totality of everything that exists, including all matter and energy, the planets, stars, galaxies, and the contents of intergalactic space. Definitions and usage vary and similar terms include the cosmos, the world and nature...
in physical cosmology
Physical cosmology
Physical cosmology, as a branch of astronomy, is the study of the largest-scale structures and dynamics of the universe and is concerned with fundamental questions about its formation and evolution. For most of human history, it was a branch of metaphysics and religion...
. Stephen Hawking
Stephen Hawking
Stephen William Hawking, CH, CBE, FRS, FRSA is an English theoretical physicist and cosmologist, whose scientific books and public appearances have made him an academic celebrity...
popularized the concept of imaginary time in his book A Brief History of Time
A Brief History of Time
A Brief History of Time is a popular science book written by renown physicist Stephen Hawking and first published by the Bantam Dell Publishing Group in 1988. It became a best-seller and has sold more than 10 million copies...
.
Imaginary time is difficult to visualize. If we imagine "regular time" as a horizontal line running between "past" in one direction and "future" in the other, then imaginary time would run perpendicular to this line as the imaginary number
Imaginary number
An imaginary number is any number whose square is a real number less than zero. When any real number is squared, the result is never negative, but the square of an imaginary number is always negative...
s run perpendicular to the real number
Real number
In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...
s in the complex plane
Complex plane
In mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...
. However, imaginary time is not imaginary in the sense that it is unreal or made-up — it simply runs in a direction different from the type of time we experience. In essence, imaginary time is a way of looking at the time dimension as if it were a dimension of space: you can move forward and backward along imaginary time, just like you can move right and left in space.
The concept is useful in cosmology because it can help smooth out gravitational singularities in models of the universe (see Hartle-Hawking state
Hartle-Hawking state
The Hartle-Hawking state is a proposal concerning the state of the universe prior to the Planck epoch. Hartle-Hawking is essentially a no-boundary proposal that the universe is infinitely finite: that there was no time before the Big Bang because time did not exist before the formation of spacetime...
). Singularities pose a problem for physicists because these are areas where known physical laws do not apply. The Big Bang
Big Bang
The Big Bang theory is the prevailing cosmological model that explains the early development of the Universe. According to the Big Bang theory, the Universe was once in an extremely hot and dense state which expanded rapidly. This rapid expansion caused the young Universe to cool and resulted in...
, for example, appears as a singularity in "regular time." But when visualized with imaginary time, the singularity is removed and the Big Bang functions like any other point in spacetime
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
.
Further reading
- Gerald D. Mahan. Many-Particle Physics, Chapter 3
- A. Zee Quantum field theory in a nutshell, Chapter V.2
External links
- The Beginning of Time — Lecture by Stephen Hawking which discusses imaginary time.
- Stephen Hawking's Universe: Strange Stuff Explained — PBS site on imaginary time