
Identity component
Encyclopedia
In mathematics
, the identity component of a topological group
G is the connected component G0 of G that contains the identity element
of the group. Similarly, the identity path component of a topological group G is the path component of G that contains the identity element of the group.
, normal subgroup
of G. It is closed since components are always closed. It is a subgroup since multiplication and inversion in a topological group are continuous maps by definition. Moreover, for any continuous automorphism
a of G we have
It follows that G0 is normal in G.
The identity component G0 of a topological group G need not be open
in G. In fact, we may have G0 = {e}, in which case G is totally disconnected
. However, the identity component of a locally path-connected space (for instance a Lie group
) is always open, since it contains a path-connected neighbourhood of {e}; and therefore is a clopen set
.
The identity path component may in general be smaller than the identity component (since path connectedness is a stronger condition than connectedness), but these agree if G is locally path-connected.
G/G0 is called the group of components or component group of G. Its elements are just the connected components of G. The component group G/G0 is a discrete group
if and only if G0 is open. If G is an affine algebraic group then G/G0 is actually a finite group
.
One may similarly define the path component group as the group of path components (quotient of G by the identity path component), and in general the component group is a quotient of the path component group, but if G is locally path connected these groups agree. The path component group can also be characterized as the zeroth homotopy group
,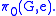
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the identity component of a topological group
Topological group
In mathematics, a topological group is a group G together with a topology on G such that the group's binary operation and the group's inverse function are continuous functions with respect to the topology. A topological group is a mathematical object with both an algebraic structure and a...
G is the connected component G0 of G that contains the identity element
Identity element
In mathematics, an identity element is a special type of element of a set with respect to a binary operation on that set. It leaves other elements unchanged when combined with them...
of the group. Similarly, the identity path component of a topological group G is the path component of G that contains the identity element of the group.
Properties
The identity component G0 of a topological group G is a closedClosed set
In geometry, topology, and related branches of mathematics, a closed set is a set whose complement is an open set. In a topological space, a closed set can be defined as a set which contains all its limit points...
, normal subgroup
Normal subgroup
In abstract algebra, a normal subgroup is a subgroup which is invariant under conjugation by members of the group. Normal subgroups can be used to construct quotient groups from a given group....
of G. It is closed since components are always closed. It is a subgroup since multiplication and inversion in a topological group are continuous maps by definition. Moreover, for any continuous automorphism
Automorphism
In mathematics, an automorphism is an isomorphism from a mathematical object to itself. It is, in some sense, a symmetry of the object, and a way of mapping the object to itself while preserving all of its structure. The set of all automorphisms of an object forms a group, called the automorphism...
a of G we have
- a(G0) = G0.
It follows that G0 is normal in G.
The identity component G0 of a topological group G need not be open
Open set
The concept of an open set is fundamental to many areas of mathematics, especially point-set topology and metric topology. Intuitively speaking, a set U is open if any point x in U can be "moved" a small amount in any direction and still be in the set U...
in G. In fact, we may have G0 = {e}, in which case G is totally disconnected
Totally disconnected group
In mathematics, a totally disconnected group is a topological group that is totally disconnected. Such topological groups are necessarily Hausdorff....
. However, the identity component of a locally path-connected space (for instance a Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
) is always open, since it contains a path-connected neighbourhood of {e}; and therefore is a clopen set
Clopen set
In topology, a clopen set in a topological space is a set which is both open and closed. That this is possible for a set is not as counter-intuitive as it might seem if the terms open and closed were thought of as antonyms; in fact they are not...
.
The identity path component may in general be smaller than the identity component (since path connectedness is a stronger condition than connectedness), but these agree if G is locally path-connected.
Component group
The quotient groupQuotient group
In mathematics, specifically group theory, a quotient group is a group obtained by identifying together elements of a larger group using an equivalence relation...
G/G0 is called the group of components or component group of G. Its elements are just the connected components of G. The component group G/G0 is a discrete group
Discrete group
In mathematics, a discrete group is a group G equipped with the discrete topology. With this topology G becomes a topological group. A discrete subgroup of a topological group G is a subgroup H whose relative topology is the discrete one...
if and only if G0 is open. If G is an affine algebraic group then G/G0 is actually a finite group
Finite group
In mathematics and abstract algebra, a finite group is a group whose underlying set G has finitely many elements. During the twentieth century, mathematicians investigated certain aspects of the theory of finite groups in great depth, especially the local theory of finite groups, and the theory of...
.
One may similarly define the path component group as the group of path components (quotient of G by the identity path component), and in general the component group is a quotient of the path component group, but if G is locally path connected these groups agree. The path component group can also be characterized as the zeroth homotopy group
Homotopy group
In mathematics, homotopy groups are used in algebraic topology to classify topological spaces. The first and simplest homotopy group is the fundamental group, which records information about loops in a space...
,
Examples
- The group of non-zero real numbers with multiplication (R*,•) has two components and the group of components is ({1,−1},•).
- Consider the group of units U in the ring of split-complex numberSplit-complex numberIn abstract algebra, the split-complex numbers are a two-dimensional commutative algebra over the real numbers different from the complex numbers. Every split-complex number has the formwhere x and y are real numbers...
s. In the ordinary topology of the plane {z = x + j y : x, y ∈ R}, U is divided into four components by the lines y = x and y = − x where z has no inverse. Then U0 = { z : |y| < x } . In this case the group of components of U is isomorphic to the Klein four-groupKlein four-groupIn mathematics, the Klein four-group is the group Z2 × Z2, the direct product of two copies of the cyclic group of order 2...
.

