In geometry

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

, the great truncated cuboctahedron (or quasitruncated cuboctahedron) is a nonconvex uniform polyhedron

Nonconvex uniform polyhedron

In geometry, a uniform star polyhedron is a self-intersecting uniform polyhedron. They are also sometimes called nonconvex polyhedra to imply self-intersecting...

, indexed as U₂₀. It is represented by Schläfli symbol t_0,1,2{4/3,3}, and Coxeter-Dynkin diagram

Coxeter-Dynkin diagram

In geometry, a Coxeter–Dynkin diagram is a graph with numerically labeled edges representing the spatial relations between a collection of mirrors...

, . It is sometimes called quasitruncated cuboctahedron because it is related to the truncated cuboctahedron

Truncated cuboctahedron

In geometry, the truncated cuboctahedron is an Archimedean solid. It has 12 square faces, 8 regular hexagonal faces, 6 regular octagonal faces, 48 vertices and 72 edges...

, , except that the octagonal faces are inverted into {8/3} octagrams.

Convex hull

Its convex hull

Convex hull

In mathematics, the convex hull or convex envelope for a set of points X in a real vector space V is the minimal convex set containing X....

 is a nonuniform truncated cuboctahedron

Truncated cuboctahedron

In geometry, the truncated cuboctahedron is an Archimedean solid. It has 12 square faces, 8 regular hexagonal faces, 6 regular octagonal faces, 48 vertices and 72 edges...

. The truncated cuboctahedron and the great truncated cuboctahedron form isomorphic graphs

Graph isomorphism

In graph theory, an isomorphism of graphs G and H is a bijection between the vertex sets of G and H f \colon V \to V \,\!such that any two vertices u and v of G are adjacent in G if and only if ƒ and ƒ are adjacent in H...

 despite their different geometric structure.

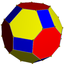Convex hull

Great truncated cuboctahedron

Cartesian coordinates

Cartesian coordinates for the vertices of a great truncated cuboctahedron centered at the origin are all permutations of

(±1, ±(1−√2), ±(1−2√2)).