
Gosper curve
Encyclopedia
The Gosper curve, named after Bill Gosper
, also known as the flowsnake (a spoonerism
of snowflake
), is a space-filling curve
. It is a fractal
object similar in its construction to the dragon curve
and the Hilbert curve
.
A Logo program to draw the Gosper curve using turtle graphics
(online version):
The program can be invoked, for example, with
The constant 2.6457 in the program code is an approximation of √7.
The Gosper Island can tile
the plane
. In fact, seven copies of the Gosper island can be joined together to form a shape that is similar
, but scaled up by a factor of √7 in all dimensions. As can be seen from the diagram below, performing this operation with an intermediate iteration of the island leads to a scaled-up version of the next iteration. Repeating this process indefinitely produces a tessellation
of the plane. The curve itself can likewise be extended to an infinite curve filling the whole plane.
Bill Gosper
Ralph William Gosper, Jr. , known as Bill Gosper, is an American mathematician and programmer from Pennsauken Township, New Jersey...
, also known as the flowsnake (a spoonerism
Spoonerism
A spoonerism is an error in speech or deliberate play on words in which corresponding consonants, vowels, or morphemes are switched . It is named after the Reverend William Archibald Spooner , Warden of New College, Oxford, who was notoriously prone to this tendency...
of snowflake
Snowflake
Snowflakes are conglomerations of frozen ice crystals which fall through the Earth's atmosphere. They begin as snow crystals which develop when microscopic supercooled cloud droplets freeze. Snowflakes come in a variety of sizes and shapes. Complex shapes emerge as the flake moves through...
), is a space-filling curve
Space-filling curve
In mathematical analysis, a space-filling curve is a curve whose range contains the entire 2-dimensional unit square...
. It is a fractal
Fractal
A fractal has been defined as "a rough or fragmented geometric shape that can be split into parts, each of which is a reduced-size copy of the whole," a property called self-similarity...
object similar in its construction to the dragon curve
Dragon curve
A dragon curve is any member of a family of self-similar fractal curves, which can be approximated by recursive methods such as Lindenmayer systems.-Heighway dragon:...
and the Hilbert curve
Hilbert curve
A Hilbert curve is a continuous fractal space-filling curve first described by the German mathematician David Hilbert in 1891, as a variant of the space-filling curves discovered by Giuseppe Peano in 1890....
.
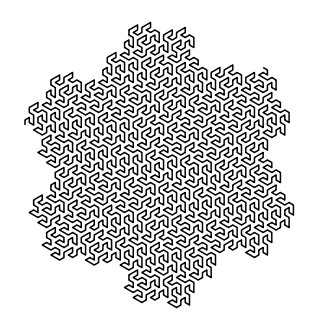 |
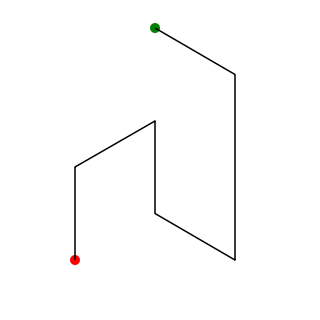 |
| A fourth-stage Gosper curve | The line from the red to the green point shows a single step of the Gosper curve construction. |
A Logo program to draw the Gosper curve using turtle graphics
Turtle graphics
Turtle graphics is a term in computer graphics for a method of programming vector graphics using a relative cursor upon a Cartesian plane...
(online version):
to rg :st :ln
make "st :st - 1
make "ln :ln / 2.6457
if :st > 0 [rg :st :ln rt 60 gl :st :ln rt 120 gl :st :ln lt 60 rg :st :ln lt 120 rg :st :ln rg :st :ln lt 60 gl :st :ln rt 60]
if :st = 0 [fd :ln rt 60 fd :ln rt 120 fd :ln lt 60 fd :ln lt 120 fd :ln fd :ln lt 60 fd :ln rt 60]
end
to gl :st :ln
make "st :st - 1
make "ln :ln / 2.6457
if :st > 0 [lt 60 rg :st :ln rt 60 gl :st :ln gl :st :ln rt 120 gl :st :ln rt 60 rg :st :ln lt 120 rg :st :ln lt 60 gl :st :ln]
if :st = 0 [lt 60 fd :ln rt 60 fd :ln fd :ln rt 120 fd :ln rt 60 fd :ln lt 120 fd :ln lt 60 fd :ln]
end
The program can be invoked, for example, with
rg 4 300, or alternatively gl 4 300.The constant 2.6457 in the program code is an approximation of √7.
Properties
The space filled by the curve is called the Gosper island. The first few iterations of it are shown below: |
 |
 |
 |
 |
The Gosper Island can tile
Tessellation
A tessellation or tiling of the plane is a pattern of plane figures that fills the plane with no overlaps and no gaps. One may also speak of tessellations of parts of the plane or of other surfaces. Generalizations to higher dimensions are also possible. Tessellations frequently appeared in the art...
the plane
Plane (mathematics)
In mathematics, a plane is a flat, two-dimensional surface. A plane is the two dimensional analogue of a point , a line and a space...
. In fact, seven copies of the Gosper island can be joined together to form a shape that is similar
Similarity (geometry)
Two geometrical objects are called similar if they both have the same shape. More precisely, either one is congruent to the result of a uniform scaling of the other...
, but scaled up by a factor of √7 in all dimensions. As can be seen from the diagram below, performing this operation with an intermediate iteration of the island leads to a scaled-up version of the next iteration. Repeating this process indefinitely produces a tessellation
Tessellation
A tessellation or tiling of the plane is a pattern of plane figures that fills the plane with no overlaps and no gaps. One may also speak of tessellations of parts of the plane or of other surfaces. Generalizations to higher dimensions are also possible. Tessellations frequently appeared in the art...
of the plane. The curve itself can likewise be extended to an infinite curve filling the whole plane.
 |
 |
External links
- http://kilin.u-shizuoka-ken.ac.jp/museum/gosperex/343-024.pdf
- http://kilin.clas.kitasato-u.ac.jp/museum/gosperex/343-024.pdf
- http://www.mathcurve.com/fractals/gosper/gosper.shtml (in French)
- http://mathworld.wolfram.com/GosperIsland.html
- http://logo.twentygototen.org/mJjiNzK0
- http://80386.nl/projects/flowsnake/

