Generalized mean
Encyclopedia
In mathematics, a generalized mean, also known as power mean or Hölder mean (named after Otto Hölder
), is an abstraction of the Pythagorean means
including arithmetic
, geometric
, and harmonic
mean
s.
, we can define the generalized mean with exponent p (or power mean with exponent p) of the positive real numbers
as:
While for p equal to 0 we assume that it's equal to the geometric mean (which is, in fact, the limit of means with exponents approaching zero):
Furthermore, for a sequence of positive weights
with sum 
we can define weighted power means as follows:
For the sake of simplicity, we might assume that the weights are normalized so that they sum up to 1 (which can be easily done by dividing each weight by their sum), thus allowing some terms in the above formulae to be omitted:
The unweighted means can be easily produced by assuming that all weights equal 1/n. For exponents equal to positive or negative infinity the means are maximum and minimum, respectively, regardless of weights (and they are actually the limit points for exponents approaching the respective extremes):
Otto Hölder
Otto Ludwig Hölder was a German mathematician born in Stuttgart.Hölder first studied at the Polytechnikum and then in 1877 went to Berlin where he was a student of Leopold Kronecker, Karl Weierstraß, and Ernst Kummer.He is famous for many things including: Hölder's inequality, the Jordan–Hölder...
), is an abstraction of the Pythagorean means
Pythagorean means
In mathematics, the three classical Pythagorean means are the arithmetic mean , the geometric mean , and the harmonic mean...
including arithmetic
Arithmetic mean
In mathematics and statistics, the arithmetic mean, often referred to as simply the mean or average when the context is clear, is a method to derive the central tendency of a sample space...
, geometric
Geometric mean
The geometric mean, in mathematics, is a type of mean or average, which indicates the central tendency or typical value of a set of numbers. It is similar to the arithmetic mean, except that the numbers are multiplied and then the nth root of the resulting product is taken.For instance, the...
, and harmonic
Harmonic mean
In mathematics, the harmonic mean is one of several kinds of average. Typically, it is appropriate for situations when the average of rates is desired....
mean
Mean
In statistics, mean has two related meanings:* the arithmetic mean .* the expected value of a random variable, which is also called the population mean....
s.
Definition
If p is a non-zero real numberReal number
In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...
, we can define the generalized mean with exponent p (or power mean with exponent p) of the positive real numbers
as:While for p equal to 0 we assume that it's equal to the geometric mean (which is, in fact, the limit of means with exponents approaching zero):
Furthermore, for a sequence of positive weights
with sum we can define weighted power means as follows:For the sake of simplicity, we might assume that the weights are normalized so that they sum up to 1 (which can be easily done by dividing each weight by their sum), thus allowing some terms in the above formulae to be omitted:
The unweighted means can be easily produced by assuming that all weights equal 1/n. For exponents equal to positive or negative infinity the means are maximum and minimum, respectively, regardless of weights (and they are actually the limit points for exponents approaching the respective extremes):
Properties
- Like most means, the generalized mean is a homogeneous functionHomogeneous functionIn mathematics, a homogeneous function is a function with multiplicative scaling behaviour: if the argument is multiplied by a factor, then the result is multiplied by some power of this factor. More precisely, if is a function between two vector spaces over a field F, and k is an integer, then...
of its arguments
. That is, if b is a positive real number, then the generalized mean with exponent p of the numbers 
is equal to b times the generalized mean of the numbers 
. - Like the quasi-arithmetic meanQuasi-arithmetic meanIn mathematics and statistics, the quasi-arithmetic mean or generalised f-mean is one generalisation of the more familiar means such as the arithmetic mean and the geometric mean, using a function f...
s, the computation of the mean can be split into computations of equal sized sub-blocks.
-
-
Generalized mean inequality
In general, if p < q, then
and the two means are equal if and only if 
.
It is true for real nonzero p, as well as zero, positive and negative infinity p, as defined above.
This follows from the fact that, for all p in
,
which can be proved using Jensen's inequalityJensen's inequalityIn mathematics, Jensen's inequality, named after the Danish mathematician Johan Jensen, relates the value of a convex function of an integral to the integral of the convex function. It was proved by Jensen in 1906. Given its generality, the inequality appears in many forms depending on the context,...
. In particular, for
, the generalized mean inequality implies the Pythagorean meansPythagorean meansIn mathematics, the three classical Pythagorean means are the arithmetic mean , the geometric mean , and the harmonic mean...
inequality as well as the inequality of arithmetic and geometric meansInequality of arithmetic and geometric meansIn mathematics, the inequality of arithmetic and geometric means, or more briefly the AM–GM inequality, states that the arithmetic mean of a list of non-negative real numbers is greater than or equal to the geometric mean of the same list; and further, that the two means are equal if and only if...
.
Special cases
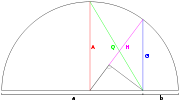
minimum 
harmonic mean Harmonic meanIn mathematics, the harmonic mean is one of several kinds of average. Typically, it is appropriate for situations when the average of rates is desired....
geometric mean Geometric meanThe geometric mean, in mathematics, is a type of mean or average, which indicates the central tendency or typical value of a set of numbers. It is similar to the arithmetic mean, except that the numbers are multiplied and then the nth root of the resulting product is taken.For instance, the...
arithmetic mean Arithmetic meanIn mathematics and statistics, the arithmetic mean, often referred to as simply the mean or average when the context is clear, is a method to derive the central tendency of a sample space...
quadratic mean, a.k.a. root mean square Root mean squareIn mathematics, the root mean square , also known as the quadratic mean, is a statistical measure of the magnitude of a varying quantity. It is especially useful when variates are positive and negative, e.g., sinusoids...
maximum
Proof of power means inequality
We will prove weighted power means inequality, for the purpose of the proof we will assume without loss of generality that:
and
Proof for unweighted power means is easily obtained by substituting
.
Equivalence of inequalities between means of opposite signs
Suppose an average between power means with exponents p and q holds:
then:
We raise both sides to the power of −1 (strictly decreasing function in positive reals):
We get the inequality for means with exponents −p and −q, and we can use the same reasoning backwards, thus proving the inequalities to be equivalent, which will be used in some of the later proofs.
Geometric mean
For any q the inequality between mean with exponent q and geometric mean can be transformed in the following way:
(the first inequality is to be proven for positive q, and the latter otherwise)
We raise both sides to the power of q:
in both cases we get the inequality between weighted arithmetic and geometric means for the sequence
, which can be proved by Jensen's inequalityJensen's inequalityIn mathematics, Jensen's inequality, named after the Danish mathematician Johan Jensen, relates the value of a convex function of an integral to the integral of the convex function. It was proved by Jensen in 1906. Given its generality, the inequality appears in many forms depending on the context,...
, making use of the fact the logarithmLogarithmThe logarithm of a number is the exponent by which another fixed value, the base, has to be raised to produce that number. For example, the logarithm of 1000 to base 10 is 3, because 1000 is 10 to the power 3: More generally, if x = by, then y is the logarithm of x to base b, and is written...
ic function is concave:
By applying (strictly increasing) expExpExp may stand for:* Exponential function, in mathematics* Expiry date of organic compounds like food or medicines* Experience points, in role-playing games* EXPTIME, a complexity class in computing* Ford EXP, a car manufactured in the 1980s...
function to both sides we get the inequality:
Thus for any positive q it is true that:
thus we have proved the inequality between geometric mean and any power mean.
Geometric mean as a limit
Furthermore, we can prove that the geometric mean is the limit of power means for exponent approaching zero. Firstly, we will prove the limit:
It's easy to conclude that the limits of both the numerator and the denominator are both 0, so we can use L'Hôpital's ruleL'Hôpital's ruleIn calculus, l'Hôpital's rule uses derivatives to help evaluate limits involving indeterminate forms. Application of the rule often converts an indeterminate form to a determinate form, allowing easy evaluation of the limit...
:
Then we make use of the exponential functionExponential functionIn mathematics, the exponential function is the function ex, where e is the number such that the function ex is its own derivative. The exponential function is used to model a relationship in which a constant change in the independent variable gives the same proportional change In mathematics,...
's continuity:
which was to be proven.
Inequality between any two power means
We are to prove that for any p < q the following inequality holds:
if p is negative, and q is positive, the inequality is equivalent to the one proved above:
The proof for positive p and q is as follows:
Define the following function:
. f is a power function, so it does have a second derivative:
which is strictly positive within the domain of f, since q > p, so we know f is convex.
Using this, and the Jensen's inequality we get:
after raising both side to the power of 1/q (an increasing function, since 1/q is positive) we get the inequality which was to be proven:
Using the previously shown equivalence we can prove the inequality for negative p and q by substituting them with, respectively, −q and −p, QED.
Minimum and maximum
Minimum and maximum are the limits of power means at, respectively,
and 
. The proof is as follows:
Suppose without loss of generality that x1 is the largest, while xn is the smallest of xi.
First, using the squeeze theoremSqueeze theoremIn calculus, the squeeze theorem is a theorem regarding the limit of a function....
we will prove that:
It suffices to notice that for positive p the inequalities hold:
Then, making use of the limit:
and finally, we use the fact that the exponential function is continuous:
Similarly, for negative p:
since (for p < 0):
Thus:
and again, by continuity of the exp function:
Generalized
The power mean could be generalized further to the generalized
-mean 
-mean:
which covers e.g. the geometric mean without using a limit. The power mean is obtained for
.
Signal processing
A power mean serves a non-linear moving average which is shifted towards small signal values for small
and emphasizes big signal values for big 
. Given an efficient implementation of a moving arithmetic mean called smoothyou can implement a moving power mean according to the following HaskellHaskell (programming language)Haskell is a standardized, general-purpose purely functional programming language, with non-strict semantics and strong static typing. It is named after logician Haskell Curry. In Haskell, "a function is a first-class citizen" of the programming language. As a functional programming language, the...
code.
powerSmooth :: Floating a => ([a] -> [a]) -> a -> [a] -> [a]
powerSmooth smooth p = map (** recip p) . smooth . map (**p)
- For big
it can serve an envelope detectorEnvelope detectorAn envelope detector is an electronic circuit that takes a high-frequency signal as input and provides an output which is the "envelope" of the original signal. The capacitor in the circuit stores up charge on the rising edge, and releases it slowly through the resistor when the signal falls...
on a rectifiedRectifierA rectifier is an electrical device that converts alternating current , which periodically reverses direction, to direct current , which flows in only one direction. The process is known as rectification...
signal. - For small
it can serve an baseline detector on a mass spectrumMass spectrumA mass spectrum is an intensity vs. m/z plot representing a chemical analysis. Hence, the mass spectrum of a sample is a pattern representing the distribution of ions by mass in a sample. It is a histogram usually acquired using an instrument called a mass spectrometer...
.
See also
- Arithmetic meanArithmetic meanIn mathematics and statistics, the arithmetic mean, often referred to as simply the mean or average when the context is clear, is a method to derive the central tendency of a sample space...
- Arithmetic-geometric mean
- AverageAverageIn mathematics, an average, or central tendency of a data set is a measure of the "middle" value of the data set. Average is one form of central tendency. Not all central tendencies should be considered definitions of average....
- Geometric meanGeometric meanThe geometric mean, in mathematics, is a type of mean or average, which indicates the central tendency or typical value of a set of numbers. It is similar to the arithmetic mean, except that the numbers are multiplied and then the nth root of the resulting product is taken.For instance, the...
- Harmonic meanHarmonic meanIn mathematics, the harmonic mean is one of several kinds of average. Typically, it is appropriate for situations when the average of rates is desired....
- Heronian mean
- Inequality of arithmetic and geometric meansInequality of arithmetic and geometric meansIn mathematics, the inequality of arithmetic and geometric means, or more briefly the AM–GM inequality, states that the arithmetic mean of a list of non-negative real numbers is greater than or equal to the geometric mean of the same list; and further, that the two means are equal if and only if...
- Lehmer mean – also a mean related to powers
- Root mean squareRoot mean squareIn mathematics, the root mean square , also known as the quadratic mean, is a statistical measure of the magnitude of a varying quantity. It is especially useful when variates are positive and negative, e.g., sinusoids...
External links
- Power mean at MathWorld
- Examples of Generalized Mean
- A proof of the Generalized Mean on PlanetMathPlanetMathPlanetMath is a free, collaborative, online mathematics encyclopedia. The emphasis is on rigour, openness, pedagogy, real-time content, interlinked content, and also community of about 24,000 people with various maths interests. Intended to be comprehensive, the project is hosted by the Digital...
-
-
