A family of curves is a set of curve

Curve

In mathematics, a curve is, generally speaking, an object similar to a line but which is not required to be straight...

s, each of which is given by a function

Function (mathematics)

In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...

 or parametrization

Parametric equation

In mathematics, parametric equation is a method of defining a relation using parameters. A simple kinematic example is when one uses a time parameter to determine the position, velocity, and other information about a body in motion....

 in which one or more of the parameters is variable. In general, the parameter(s) influence the shape of the curve in a way that is more complicated than a simple linear transformation

Linear transformation

In mathematics, a linear map, linear mapping, linear transformation, or linear operator is a function between two vector spaces that preserves the operations of vector addition and scalar multiplication. As a result, it always maps straight lines to straight lines or 0...

. Sets of curves given by an implicit relation may also represent families of curves.

Families of curves appear frequently in solutions of differential equation

Differential equation

A differential equation is a mathematical equation for an unknown function of one or several variables that relates the values of the function itself and its derivatives of various orders...

s; when an additive constant of integration is introduced, it will usually be manipulated algebraically until it no longer represents a simple linear transformation.

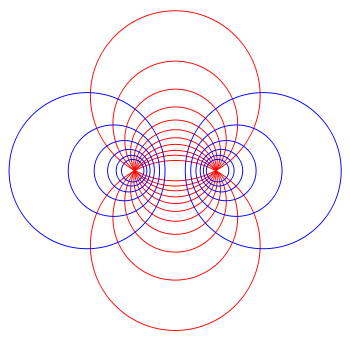
Families of curves may also arise in other areas. For example, all non-degenerate conic sections can be represented using a single polar equation with one parameter, the eccentricity of the curve:


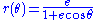
as the value of e changes, the appearance of the curve varies in a relatively complicated way.

Applications

Families of curves may arise in various topics in geometry, including the envelope

Envelope (mathematics)

In geometry, an envelope of a family of curves in the plane is a curve that is tangent to each member of the family at some point. Classically, a point on the envelope can be thought of as the intersection of two "adjacent" curves, meaning the limit of intersections of nearby curves...

 of a set of curves and the caustic

Caustic (optics)

In optics, a caustic or caustic network is the envelope of light rays reflected or refracted by a curved surface or object, or the projection of that envelope of rays on another surface. The caustic is a curve or surface to which each of the light rays is tangent, defining a boundary of an...

 of a given curve.

Generalizations

In algebraic geometry

Algebraic geometry

Algebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...

, an algebraic generalization is given by the notion of a linear system of divisors

Linear system of divisors

In algebraic geometry, a linear system of divisors is an algebraic generalization of the geometric notion of a family of curves; the dimension of the linear system corresponds to the number of parameters of the family....

.