Dual polygon
Encyclopedia
In geometry
, polygon
s are associated into pairs called duals, where the vertices
of one correspond to the edge
s of the other.
 Regular polygon
Regular polygon
s are self-dual.
The dual of an isogonal (vertex-transitive) polygon is an isotoxal (edge-transitive) polygon. For example, the (isogonal) rectangle
and (isotoxal) rhombus
are duals.
In a cyclic polygon, smaller angles correspond to shorter sides, and bigger angles to bigger sides – further, congruent sides in the original yield congruent angles in the dual, and conversely. For example the dual of an acute isosceles triangle is an obtuse isosceles triangle.
In the Dorman Luke construction, each face of a dual polyhedron
is the dual polygon of the corresponding vertex figure
.
operation, where the edges of a polygon are truncated
down to vertices at the center of each original edge. New edges are formed between these new vertices.
This construction is not reversible. That is, the polygon generated by applying it twice is not, in general, congruent to the original polygon.
) and perform polar reciprocation in it.
From the point of view of the dual curve
, where to each point on a curve one associates the point dual to its tangent line at that point, the projective dual can be interpreted thus:
Thus for the triangle with vertices {A,B,C} and edges {AB,BC,CA}, the dual triangle has vertices {AB,BC,CA}, and edges {B,C,A}, where B connects AB & BC, and so forth.
This is not a particularly fruitful avenue, as combinatorially, there is a single family of polygons (given by number of sides); geometric duality of polygons is more varied, as are combinatorial dual polyhedra.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, polygon
Polygon
In geometry a polygon is a flat shape consisting of straight lines that are joined to form a closed chain orcircuit.A polygon is traditionally a plane figure that is bounded by a closed path, composed of a finite sequence of straight line segments...
s are associated into pairs called duals, where the vertices
Vertex (geometry)
In geometry, a vertex is a special kind of point that describes the corners or intersections of geometric shapes.-Of an angle:...
of one correspond to the edge
Edge (geometry)
In geometry, an edge is a one-dimensional line segment joining two adjacent zero-dimensional vertices in a polygon. Thus applied, an edge is a connector for a one-dimensional line segment and two zero-dimensional objects....
s of the other.
Properties

Regular polygon
A regular polygon is a polygon that is equiangular and equilateral . Regular polygons may be convex or star.-General properties:...
s are self-dual.
The dual of an isogonal (vertex-transitive) polygon is an isotoxal (edge-transitive) polygon. For example, the (isogonal) rectangle
Rectangle
In Euclidean plane geometry, a rectangle is any quadrilateral with four right angles. The term "oblong" is occasionally used to refer to a non-square rectangle...
and (isotoxal) rhombus
Rhombus
In Euclidean geometry, a rhombus or rhomb is a convex quadrilateral whose four sides all have the same length. The rhombus is often called a diamond, after the diamonds suit in playing cards, or a lozenge, though the latter sometimes refers specifically to a rhombus with a 45° angle.Every...
are duals.
In a cyclic polygon, smaller angles correspond to shorter sides, and bigger angles to bigger sides – further, congruent sides in the original yield congruent angles in the dual, and conversely. For example the dual of an acute isosceles triangle is an obtuse isosceles triangle.
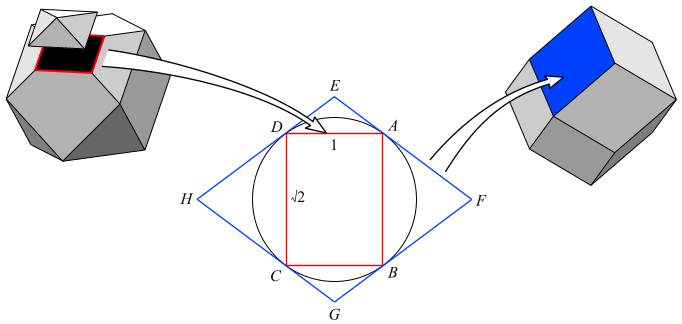
In the Dorman Luke construction, each face of a dual polyhedron
Dual polyhedron
In geometry, polyhedra are associated into pairs called duals, where the vertices of one correspond to the faces of the other. The dual of the dual is the original polyhedron. The dual of a polyhedron with equivalent vertices is one with equivalent faces, and of one with equivalent edges is another...
is the dual polygon of the corresponding vertex figure
Vertex figure
In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...
.
Rectification
The simplest qualitative construction of a dual polygon is a rectificationRectification (geometry)
In Euclidean geometry, rectification is the process of truncating a polytope by marking the midpoints of all its edges, and cutting off its vertices at those points...
operation, where the edges of a polygon are truncated
Truncation (geometry)
In geometry, a truncation is an operation in any dimension that cuts polytope vertices, creating a new facet in place of each vertex.- Uniform truncation :...
down to vertices at the center of each original edge. New edges are formed between these new vertices.
This construction is not reversible. That is, the polygon generated by applying it twice is not, in general, congruent to the original polygon.
Polar reciprocation
As with dual polyhedra, one can take a circle (be it the inscribed circle, midcircle, or circumscribed circleCircumscribed circle
In geometry, the circumscribed circle or circumcircle of a polygon is a circle which passes through all the vertices of the polygon. The center of this circle is called the circumcenter....
) and perform polar reciprocation in it.
Projective duality
Under projective duality, the dual of a point is a line, and of a line is a point – thus the dual of a polygon is a polygon, with edges of the original corresponding to vertices of the dual and conversely.From the point of view of the dual curve
Dual curve
In projective geometry, a dual curve of a given plane curve C is a curve in the dual projective plane consisting of the set of lines tangent to C. There is a map from a curve to its dual, sending each point to the point dual to its tangent line. If C is algebraic then so is its dual and the degree...
, where to each point on a curve one associates the point dual to its tangent line at that point, the projective dual can be interpreted thus:
- every point on a side of a polygon has the same tangent line, which agrees with the side itself – they thus all map to the same vertex in the dual polygon
- at a vertex, the "tangent lines" to that vertex are all lines through that point with angle between the two edges – the dual points to these lines are then the edge in the dual polygon.
Combinatorially
Combinatorially, one can define a polygon as a set of vertices, a set of edges, and an incidence relation (which vertices and edges touch): two adjacent vertices determine an edge, and dually, two adjacent edges determine a vertex. Then the dual polygon is obtained by simply switching the vertices and edges.Thus for the triangle with vertices {A,B,C} and edges {AB,BC,CA}, the dual triangle has vertices {AB,BC,CA}, and edges {B,C,A}, where B connects AB & BC, and so forth.
This is not a particularly fruitful avenue, as combinatorially, there is a single family of polygons (given by number of sides); geometric duality of polygons is more varied, as are combinatorial dual polyhedra.
See also
- Dual curveDual curveIn projective geometry, a dual curve of a given plane curve C is a curve in the dual projective plane consisting of the set of lines tangent to C. There is a map from a curve to its dual, sending each point to the point dual to its tangent line. If C is algebraic then so is its dual and the degree...
- Dual polyhedronDual polyhedronIn geometry, polyhedra are associated into pairs called duals, where the vertices of one correspond to the faces of the other. The dual of the dual is the original polyhedron. The dual of a polyhedron with equivalent vertices is one with equivalent faces, and of one with equivalent edges is another...
- Self-dual polygon

