
Small ditrigonal icosidodecahedron
Encyclopedia
In geometry
, the small ditrigonal icosidodecahedron is a nonconvex uniform polyhedron
, indexed as U30.
is a regular dodecahedron. It additionally shares its edge arrangement with the great ditrigonal icosidodecahedron (having the triangular faces in common), the ditrigonal dodecadodecahedron
(having the pentagrammic faces in common), and the regular compound of five cubes
.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, the small ditrigonal icosidodecahedron is a nonconvex uniform polyhedron
Nonconvex uniform polyhedron
In geometry, a uniform star polyhedron is a self-intersecting uniform polyhedron. They are also sometimes called nonconvex polyhedra to imply self-intersecting...
, indexed as U30.
Related polyhedra
Its convex hullConvex hull
In mathematics, the convex hull or convex envelope for a set of points X in a real vector space V is the minimal convex set containing X....
is a regular dodecahedron. It additionally shares its edge arrangement with the great ditrigonal icosidodecahedron (having the triangular faces in common), the ditrigonal dodecadodecahedron
Ditrigonal dodecadodecahedron
In geometry, the Ditrigonal dodecadodecahedron is a nonconvex uniform polyhedron, indexed as U41.- Related polyhedra :Its convex hull is a regular dodecahedron...
(having the pentagrammic faces in common), and the regular compound of five cubes
Compound of five cubes
This polyhedral compound is a symmetric arrangement of five cubes. This compound was first described by Edmund Hess in 1876.It is one of five regular compounds, and dual to the compound of five octahedra....
.
 Small ditrigonal icosidodecahedron |
 Great ditrigonal icosidodecahedron |
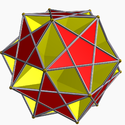 Ditrigonal dodecadodecahedron Ditrigonal dodecadodecahedron In geometry, the Ditrigonal dodecadodecahedron is a nonconvex uniform polyhedron, indexed as U41.- Related polyhedra :Its convex hull is a regular dodecahedron... |
 Dodecahedron (convex hull Convex hull In mathematics, the convex hull or convex envelope for a set of points X in a real vector space V is the minimal convex set containing X.... ) |
 Compound of five cubes Compound of five cubes This polyhedral compound is a symmetric arrangement of five cubes. This compound was first described by Edmund Hess in 1876.It is one of five regular compounds, and dual to the compound of five octahedra.... |

