
Realization (probability)
Encyclopedia
In probability
and statistics
, a realization, or observed value, of a random variable
is the value that is actually observed (what actually happened). The random variable itself should be thought of as the process how the observation comes about. Statistical quantities computed from realizations without deploying a statistical model are often called "empirical
", as in empirical distribution function
or empirical probability
.
Conventionally, upper case letters denote random variables; the corresponding lower case letters denote their realizations. Confusion results when this important convention is not strictly observed.
In more formal probability theory
, a random variable is a function
X defined from a sample space Ω to a measurable space called the state space. If an element in Ω is sent to an element in state space by X, then that element in state space is a realization. (In fact, a random variable
cannot be an arbitrary function and it needs to satisfy another condition: it needs to be measurable.) Elements of the sample space can be thought of as all the different possibilities that could happen; while a realization (an element of the state space) can be thought of as the value X attains when one of the possibilities did happen. Probability
is a mapping
that assigns numbers between zero and one to certain subset
s of the sample space. Subsets of the sample space that contain only one element are called elementary event
s. The value of the random variable (that is, the function) X at a point ω ∈ Ω,
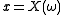
is called a realization of X.
Probability
Probability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
and statistics
Statistics
Statistics is the study of the collection, organization, analysis, and interpretation of data. It deals with all aspects of this, including the planning of data collection in terms of the design of surveys and experiments....
, a realization, or observed value, of a random variable
Random variable
In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
is the value that is actually observed (what actually happened). The random variable itself should be thought of as the process how the observation comes about. Statistical quantities computed from realizations without deploying a statistical model are often called "empirical
Empirical
The word empirical denotes information gained by means of observation or experimentation. Empirical data are data produced by an experiment or observation....
", as in empirical distribution function
Empirical distribution function
In statistics, the empirical distribution function, or empirical cdf, is the cumulative distribution function associated with the empirical measure of the sample. This cdf is a step function that jumps up by 1/n at each of the n data points. The empirical distribution function estimates the true...
or empirical probability
Empirical probability
Empirical probability, also known as relative frequency, or experimental probability, is the ratio of the number of "favorable" outcomes to the total number of trials, not in a sample space but in an actual sequence of experiments...
.
Conventionally, upper case letters denote random variables; the corresponding lower case letters denote their realizations. Confusion results when this important convention is not strictly observed.
In more formal probability theory
Probability theory
Probability theory is the branch of mathematics concerned with analysis of random phenomena. The central objects of probability theory are random variables, stochastic processes, and events: mathematical abstractions of non-deterministic events or measured quantities that may either be single...
, a random variable is a function
Function (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
X defined from a sample space Ω to a measurable space called the state space. If an element in Ω is sent to an element in state space by X, then that element in state space is a realization. (In fact, a random variable
Random variable
In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
cannot be an arbitrary function and it needs to satisfy another condition: it needs to be measurable.) Elements of the sample space can be thought of as all the different possibilities that could happen; while a realization (an element of the state space) can be thought of as the value X attains when one of the possibilities did happen. Probability
Probability
Probability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
is a mapping
Map (mathematics)
In most of mathematics and in some related technical fields, the term mapping, usually shortened to map, is either a synonym for function, or denotes a particular kind of function which is important in that branch, or denotes something conceptually similar to a function.In graph theory, a map is a...
that assigns numbers between zero and one to certain subset
Subset
In mathematics, especially in set theory, a set A is a subset of a set B if A is "contained" inside B. A and B may coincide. The relationship of one set being a subset of another is called inclusion or sometimes containment...
s of the sample space. Subsets of the sample space that contain only one element are called elementary event
Elementary event
In probability theory, an elementary event or atomic event is a singleton of a sample space. An outcome is an element of a sample space. An elementary event is a set containing exactly one outcome, not the outcome itself...
s. The value of the random variable (that is, the function) X at a point ω ∈ Ω,
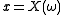
is called a realization of X.

