
Polyominoid
Encyclopedia
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, a polyominoid (or minoid for short) is a set of equal square
Square (geometry)
In geometry, a square is a regular quadrilateral. This means that it has four equal sides and four equal angles...
s in 3D
Three-dimensional space
Three-dimensional space is a geometric 3-parameters model of the physical universe in which we live. These three dimensions are commonly called length, width, and depth , although any three directions can be chosen, provided that they do not lie in the same plane.In physics and mathematics, a...
space, joined edge to edge at 90- or 180-degree angles. The polyominoids include the polyomino
Polyomino
A polyomino is a plane geometric figure formed by joining one or more equal squares edge to edge. It is a polyform whose cells are squares. It may be regarded as a finite subset of the regular square tiling with a connected interior....
es, which are just the planar polyominoids. The surface of a cube
Cube
In geometry, a cube is a three-dimensional solid object bounded by six square faces, facets or sides, with three meeting at each vertex. The cube can also be called a regular hexahedron and is one of the five Platonic solids. It is a special kind of square prism, of rectangular parallelepiped and...
is an example of a hexominoid, or 6-cell polyominoid. Polyominoids appear to have been first proposed by Richard A. Epstein.
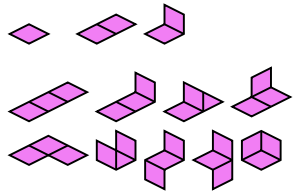
90-degree connections are called hard; 180-degree connections are called soft. This is because, in manufacturing a model of the polyominoid, a hard connection would be easier to realize than a soft one. Polyominoids may be classified as hard, soft, and mixed according to how their edges are joined, except that the unique monominoid has no connections of either kind, which makes it both hard and soft by default.
The number of soft polyominoids for each n equals the number of polyominoes for the same n.
As with other polyform
Polyform
In recreational mathematics, a polyform is a plane figure constructed by joining together identical basic polygons. The basic polygon is often a convex plane-filling polygon, such as a square or a triangle. More specific names have been given to polyforms resulting from specific basic polygons, as...
s, two polyominoids that are mirror images may be distinguished. One-sided polyominoids distinguish mirror images; free polyominoids do not.
The table below enumerates soft, hard and mixed free polyominoids of up to 4 cells and the total number of free and one-sided polyominoids of up to 6 cells.
| |One-sided Total |
|||||
|---|---|---|---|---|---|
| Cells | Soft | Hard | Mixed | Total | |
| 1 | 0 | 1 | 0 | 1 | 1 |
| 2 | 1 | 1 | 0 | 2 | 2 |
| 3 | 2 | 5 | 2 | 9 | 11 |
| 4 | 5 | 15 | 34 | 54 | 80 |
| 5 | ? | ? | ? | 448 | 780 |
| 6 | ? | ? | ? | 4650 | 8781 |
Generalization to higher dimensions
In general one can define an n,k-polyominoid as a polyformPolyform
In recreational mathematics, a polyform is a plane figure constructed by joining together identical basic polygons. The basic polygon is often a convex plane-filling polygon, such as a square or a triangle. More specific names have been given to polyforms resulting from specific basic polygons, as...
made by joining k-dimensional hypercubes at 90° or 180° angles in n-dimensional space, where 1≤k≤n.
- PolystickPolystickIn recreational mathematics, a polystick is a polyform with a line segment as the basic shape. Polysticks result when identical line segments are joined together end-to-end at 0° or 90° angles....
s are 2,1-polyominoids. - PolyominoPolyominoA polyomino is a plane geometric figure formed by joining one or more equal squares edge to edge. It is a polyform whose cells are squares. It may be regarded as a finite subset of the regular square tiling with a connected interior....
es are 2,2-polyominoids. - The polyforms described above are 3,2-polyominoids.
- PolycubePolycubethumb|200px|right|The seven free tetracubesthumb|200px|right|A [[Chirality |chiral]] pentacubethumb|200px|right|Puzzle with a unique solution...
s are 3,3-polyominoids.

