Piano acoustics
Encyclopedia
Piano acoustics are those physical properties of the piano
which affect its acoustics
.
The strings of a piano
vary in thickness, and therefore in mass per length, with bass strings thicker than treble. A typical range is from 1/30 inch (.85 mm) for the highest treble strings to 1/3 inch (8.5 mm) for the lowest bass. These differences in string thickness follow from well-understood acoustic properties of strings.
Given two strings, equally taut and heavy, one twice as long as the other, the longer would vibrate with a pitch one octave
lower than the shorter. However, if one were to use this principle to design a piano it would be impossible to fit the bass strings onto a frame of any reasonable size. Furthermore, in such a hypothetical, gigantic piano, the lowest strings would travel so far in vibrating that they would strike one another. Instead, piano makers take advantage of the fact that a heavy string vibrates more slowly than a light string of identical length and tension; thus, the bass strings on the piano are much thicker than the others.
In an ideal vibrating string, when the wavelength of a wave on a stretched string is much greater than the thickness of the string, the wave velocity on the string is constant and the overtones are at the harmonics. That is why so many instruments are constructed of strings or thin columns of air. However, for high overtones with very short wavelengths, the thin string behaves more like a thick metal bar. The mechanical resistance of the string to bending becomes an additional force. Unless this bending force is much smaller than the tension of the string, it will raise the wave speed. This raises the frequency of the overtones above the harmonics of the fundamental, producing an unpleasant effect called "inharmonicity
".
Basic strategies to reduce inharmonicity include decreasing the thickness or increasing the wavelength of the string, choosing a flexible material with a low bending force, and increasing the tension force so that it stays much bigger than the bending force.
Winding a string allows an effective decrease in the thickness of the string. In a wound string, only the inner core resists bending while the windings function only to increase the linear density of the string. The thickness of the inner core is limited by its strength and by its tension; stronger materials allow for thinner cores at higher tensions, reducing inharmonicity. Hence, piano designers choose high quality steel for their strings.
A larger piano allows for longer wires with longer wavelengths. Piano designers strive to fit the longest strings possible within the case; moreover, all else being equal, the sensible piano buyer tries to obtain the largest instrument compatible with budget and space.
Inharmonicity largely affects the lowest and highest notes in the piano and is one of the limits on the total range of a piano. The lowest strings, which would have to be the longest, are most limited by the size of the piano. The designer of a short piano is forced to use thick strings to increase mass density and is thus driven into inharmonicity.
The highest strings have to be under the greatest tension, yet must also be thin to allow for a low mass density. The limited strength of steel forces the piano designer to use very short strings whose short wavelengths thus generate inharmonicity.
The natural inharmonicity of a piano is used by the tuner
to make slight adjustments in the tuning of a piano. The tuner will stretch
the notes, slightly sharpening the high notes and lowering the low notes so that the overtones of low notes have the same frequency as the fundamentals of high notes.
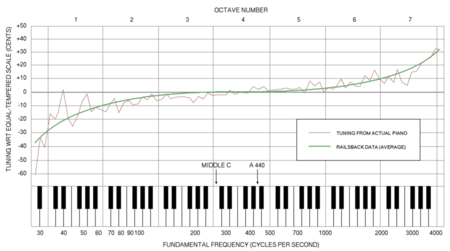The Railsback curve, first measured by O.L. Railsback, expresses the difference between normal piano tuning
and an equal-tempered
scale (one in which the frequencies of successive notes are related by a constant ratio, equal to the twelfth root of two). For any given note
on the piano
, the deviation between the normal pitch of that note and its equal-tempered pitch is given in cent
s (hundredths of a semitone
).
As the Railsback curve shows, octave
s are normally stretched
on a well-tuned piano. That is, the high notes are higher, and the low notes lower, than they are in an equal-tempered scale. Not all octaves are equally stretched: the middle octaves are barely stretched at all, whereas the octaves on either end of the piano are stretched considerably.
Railsback discovered that pianos were typically tuned in this manner not because of a lack of precision, but because of inharmonicity
in the strings. Ideally, the overtone
series of a note consists of frequencies that are integer multiples of the note's fundamental frequency
. Inharmonicity causes the successive overtones to be higher than they "should" be.
In order to tune an octave, a piano technician must reduce the speed of beating
between the first overtone of a lower note and a higher note until it disappears. Because of inharmonicity, this first overtone will be sharper than a harmonic octave (which has the ratio of 2/1), making either the lower note flatter, or the higher note sharper, depending on which one is being tuned to. To produce an even tuning, the technician begins by tuning an octave in the middle of the piano first, and proceeds to tune outwards from there; notes from the upper range are not compared to notes in the lower range for the purposes of tuning.
of the inharmonicity at an octave, is monotonically increasing
. Because the inharmonicity is lower in the middle octaves of the piano, the Railsback curve has a shallower slope in this area.
The inharmonicity in a string is caused primarily by its stiffness. Decreased length and increased thickness both contribute to inharmonicity. For the middle to high part of the piano range, string thickness remains constant as length decreases, contributing to greater inharmonicity in the higher notes. For the low range of the instrument, string thickness is drastically increased, especially in shorter pianos which cannot compensate with longer strings, producing greater inharmonicity in this range as well.
In the bass register, a second factor affecting the inharmonicity is the resonance
caused by the acoustic impedance
of the piano sounding board
. These resonances exhibit positive feedback on the inharmonic effect: if a string vibrates at a frequency just below that of a resonance, the impedance will cause it to vibrate even lower, and if it vibrates just above a resonance, the impedance causes it to vibrate higher. The sounding board has multiple resonant frequencies which are unique to any particular piano. This contributes to the greater variance in the empirically measured Railsback curve in the lower octaves.
in the ADSR system.
The three strings create a coupled oscillator with three normal modes (with two polarizations each). Since the strings are only weakly coupled, the normal modes have imperceptibly different frequencies. But they transfer their vibrational energy to the sounding board at significantly different rates.
The normal mode in which the three strings oscillate together is most efficient at transferring energy since all three strings pull in the same direction at the same time. It sounds loud, but decays quickly. This normal mode is responsible for the rapid staccato "Attack" part of the note.
In the other two normal modes the strings do not all pull together, e.g., one will pull up while the other two pull down. There is slow transfer of energy to the sounding board, generating a soft but near-constant "Sustain".
Piano
The piano is a musical instrument played by means of a keyboard. It is one of the most popular instruments in the world. Widely used in classical and jazz music for solo performances, ensemble use, chamber music and accompaniment, the piano is also very popular as an aid to composing and rehearsal...
which affect its acoustics
Acoustics
Acoustics is the interdisciplinary science that deals with the study of all mechanical waves in gases, liquids, and solids including vibration, sound, ultrasound and infrasound. A scientist who works in the field of acoustics is an acoustician while someone working in the field of acoustics...
.
String length and mass
Piano wire
Piano wire, or "music wire", is a specialized type of wire made for use in piano strings, as well as many other purposes. It is made from tempered high-carbon steel, also known as spring steel.-Manufacture and use:...
vary in thickness, and therefore in mass per length, with bass strings thicker than treble. A typical range is from 1/30 inch (.85 mm) for the highest treble strings to 1/3 inch (8.5 mm) for the lowest bass. These differences in string thickness follow from well-understood acoustic properties of strings.
Given two strings, equally taut and heavy, one twice as long as the other, the longer would vibrate with a pitch one octave
Octave
In music, an octave is the interval between one musical pitch and another with half or double its frequency. The octave relationship is a natural phenomenon that has been referred to as the "basic miracle of music", the use of which is "common in most musical systems"...
lower than the shorter. However, if one were to use this principle to design a piano it would be impossible to fit the bass strings onto a frame of any reasonable size. Furthermore, in such a hypothetical, gigantic piano, the lowest strings would travel so far in vibrating that they would strike one another. Instead, piano makers take advantage of the fact that a heavy string vibrates more slowly than a light string of identical length and tension; thus, the bass strings on the piano are much thicker than the others.
Inharmonicity and piano size
Any vibrating object will vibrate at a number of frequencies above the fundamental, called overtones. When the overtones are integer multiples (e.g., 2 x or 6 x ) of the fundamental frequency (called harmonics), then the oscillation is periodic, i.e., it vibrates in exactly the same way over and over again. Humans seem to enjoy the sound of periodic oscillations. For this reason, many musical instruments, including pianos, are designed to produce nearly periodic oscillations, that is, to have overtones as close as possible to the harmonics of the fundamental tone.In an ideal vibrating string, when the wavelength of a wave on a stretched string is much greater than the thickness of the string, the wave velocity on the string is constant and the overtones are at the harmonics. That is why so many instruments are constructed of strings or thin columns of air. However, for high overtones with very short wavelengths, the thin string behaves more like a thick metal bar. The mechanical resistance of the string to bending becomes an additional force. Unless this bending force is much smaller than the tension of the string, it will raise the wave speed. This raises the frequency of the overtones above the harmonics of the fundamental, producing an unpleasant effect called "inharmonicity
Inharmonicity
In music, inharmonicity is the degree to which the frequencies of overtones depart from whole multiples of the fundamental frequency....
".
Basic strategies to reduce inharmonicity include decreasing the thickness or increasing the wavelength of the string, choosing a flexible material with a low bending force, and increasing the tension force so that it stays much bigger than the bending force.
Winding a string allows an effective decrease in the thickness of the string. In a wound string, only the inner core resists bending while the windings function only to increase the linear density of the string. The thickness of the inner core is limited by its strength and by its tension; stronger materials allow for thinner cores at higher tensions, reducing inharmonicity. Hence, piano designers choose high quality steel for their strings.
A larger piano allows for longer wires with longer wavelengths. Piano designers strive to fit the longest strings possible within the case; moreover, all else being equal, the sensible piano buyer tries to obtain the largest instrument compatible with budget and space.
Inharmonicity largely affects the lowest and highest notes in the piano and is one of the limits on the total range of a piano. The lowest strings, which would have to be the longest, are most limited by the size of the piano. The designer of a short piano is forced to use thick strings to increase mass density and is thus driven into inharmonicity.
The highest strings have to be under the greatest tension, yet must also be thin to allow for a low mass density. The limited strength of steel forces the piano designer to use very short strings whose short wavelengths thus generate inharmonicity.
The natural inharmonicity of a piano is used by the tuner
Piano tuning
Piano tuning is the act of making minute adjustments to the tensions of the strings of a piano to properly align the intervals between their tones so that the instrument is in tune. The meaning of the term in tune in the context of piano tuning is not simply a particular fixed set of pitches...
to make slight adjustments in the tuning of a piano. The tuner will stretch
Stretched tuning
Stretched tuning is a detail of musical tuning, applied to wire-stringed musical instruments, older, non-digital electric pianos , and some sample-based synthesizers based on these instruments, to accommodate the natural inharmonicity of their vibrating elements...
the notes, slightly sharpening the high notes and lowering the low notes so that the overtones of low notes have the same frequency as the fundamentals of high notes.
- See also Piano wirePiano wirePiano wire, or "music wire", is a specialized type of wire made for use in piano strings, as well as many other purposes. It is made from tempered high-carbon steel, also known as spring steel.-Manufacture and use:...
, Piano tuningPiano tuningPiano tuning is the act of making minute adjustments to the tensions of the strings of a piano to properly align the intervals between their tones so that the instrument is in tune. The meaning of the term in tune in the context of piano tuning is not simply a particular fixed set of pitches...
, PsychoacousticsPsychoacousticsPsychoacoustics is the scientific study of sound perception. More specifically, it is the branch of science studying the psychological and physiological responses associated with sound...
.
The Railsback curve
Piano tuning
Piano tuning is the act of making minute adjustments to the tensions of the strings of a piano to properly align the intervals between their tones so that the instrument is in tune. The meaning of the term in tune in the context of piano tuning is not simply a particular fixed set of pitches...
and an equal-tempered
Equal temperament
An equal temperament is a musical temperament, or a system of tuning, in which every pair of adjacent notes has an identical frequency ratio. As pitch is perceived roughly as the logarithm of frequency, this means that the perceived "distance" from every note to its nearest neighbor is the same for...
scale (one in which the frequencies of successive notes are related by a constant ratio, equal to the twelfth root of two). For any given note
Note
In music, the term note has two primary meanings:#A sign used in musical notation to represent the relative duration and pitch of a sound;#A pitched sound itself....
on the piano
Piano
The piano is a musical instrument played by means of a keyboard. It is one of the most popular instruments in the world. Widely used in classical and jazz music for solo performances, ensemble use, chamber music and accompaniment, the piano is also very popular as an aid to composing and rehearsal...
, the deviation between the normal pitch of that note and its equal-tempered pitch is given in cent
Cent (music)
The cent is a logarithmic unit of measure used for musical intervals. Twelve-tone equal temperament divides the octave into 12 semitones of 100 cents each...
s (hundredths of a semitone
Semitone
A semitone, also called a half step or a half tone, is the smallest musical interval commonly used in Western tonal music, and it is considered the most dissonant when sounded harmonically....
).
As the Railsback curve shows, octave
Octave
In music, an octave is the interval between one musical pitch and another with half or double its frequency. The octave relationship is a natural phenomenon that has been referred to as the "basic miracle of music", the use of which is "common in most musical systems"...
s are normally stretched
Stretched tuning
Stretched tuning is a detail of musical tuning, applied to wire-stringed musical instruments, older, non-digital electric pianos , and some sample-based synthesizers based on these instruments, to accommodate the natural inharmonicity of their vibrating elements...
on a well-tuned piano. That is, the high notes are higher, and the low notes lower, than they are in an equal-tempered scale. Not all octaves are equally stretched: the middle octaves are barely stretched at all, whereas the octaves on either end of the piano are stretched considerably.
Railsback discovered that pianos were typically tuned in this manner not because of a lack of precision, but because of inharmonicity
Inharmonicity
In music, inharmonicity is the degree to which the frequencies of overtones depart from whole multiples of the fundamental frequency....
in the strings. Ideally, the overtone
Overtone
An overtone is any frequency higher than the fundamental frequency of a sound. The fundamental and the overtones together are called partials. Harmonics are partials whose frequencies are whole number multiples of the fundamental These overlapping terms are variously used when discussing the...
series of a note consists of frequencies that are integer multiples of the note's fundamental frequency
Fundamental frequency
The fundamental frequency, often referred to simply as the fundamental and abbreviated f0, is defined as the lowest frequency of a periodic waveform. In terms of a superposition of sinusoids The fundamental frequency, often referred to simply as the fundamental and abbreviated f0, is defined as the...
. Inharmonicity causes the successive overtones to be higher than they "should" be.
In order to tune an octave, a piano technician must reduce the speed of beating
Beat (acoustics)
In acoustics, a beat is an interference between two sounds of slightly different frequencies, perceived as periodic variations in volume whose rate is the difference between the two frequencies....
between the first overtone of a lower note and a higher note until it disappears. Because of inharmonicity, this first overtone will be sharper than a harmonic octave (which has the ratio of 2/1), making either the lower note flatter, or the higher note sharper, depending on which one is being tuned to. To produce an even tuning, the technician begins by tuning an octave in the middle of the piano first, and proceeds to tune outwards from there; notes from the upper range are not compared to notes in the lower range for the purposes of tuning.
Shape of the curve
Because string inharmonicity only causes harmonics to be sharper, the Railsback curve, which is functionally the integralIntegral
Integration is an important concept in mathematics and, together with its inverse, differentiation, is one of the two main operations in calculus...
of the inharmonicity at an octave, is monotonically increasing
Monotonic function
In mathematics, a monotonic function is a function that preserves the given order. This concept first arose in calculus, and was later generalized to the more abstract setting of order theory....
. Because the inharmonicity is lower in the middle octaves of the piano, the Railsback curve has a shallower slope in this area.
The inharmonicity in a string is caused primarily by its stiffness. Decreased length and increased thickness both contribute to inharmonicity. For the middle to high part of the piano range, string thickness remains constant as length decreases, contributing to greater inharmonicity in the higher notes. For the low range of the instrument, string thickness is drastically increased, especially in shorter pianos which cannot compensate with longer strings, producing greater inharmonicity in this range as well.
In the bass register, a second factor affecting the inharmonicity is the resonance
Resonance
In physics, resonance is the tendency of a system to oscillate at a greater amplitude at some frequencies than at others. These are known as the system's resonant frequencies...
caused by the acoustic impedance
Acoustic impedance
The acoustic impedance at a particular frequency indicates how much sound pressure is generated by a given air vibration at that frequency. The acoustic impedance Z is frequency dependent and is very useful, for example, for describing the behaviour of musical wind instruments...
of the piano sounding board
Sounding board
A sound board, or soundboard, is the surface of a string instrument that the strings vibrate against, usually via some sort of bridge. The resonant properties of the sound board and the interior of the instrument greatly increase loudness over the string alone.The sound board operates by the...
. These resonances exhibit positive feedback on the inharmonic effect: if a string vibrates at a frequency just below that of a resonance, the impedance will cause it to vibrate even lower, and if it vibrates just above a resonance, the impedance causes it to vibrate higher. The sounding board has multiple resonant frequencies which are unique to any particular piano. This contributes to the greater variance in the empirically measured Railsback curve in the lower octaves.
Multiple strings
All but the lowest notes of a piano have multiple strings tuned to the same frequency. This allows the piano to have a loud attack with a fast decay but a long sustainSustain
In music, sustain is a parameter of musical sound over time. As its name implies, it denotes the period of time during which the sound remains before it becomes inaudible, or silent.Additionally, sustain is the third of the four segments in an ADSR envelope...
in the ADSR system.
The three strings create a coupled oscillator with three normal modes (with two polarizations each). Since the strings are only weakly coupled, the normal modes have imperceptibly different frequencies. But they transfer their vibrational energy to the sounding board at significantly different rates.
The normal mode in which the three strings oscillate together is most efficient at transferring energy since all three strings pull in the same direction at the same time. It sounds loud, but decays quickly. This normal mode is responsible for the rapid staccato "Attack" part of the note.
In the other two normal modes the strings do not all pull together, e.g., one will pull up while the other two pull down. There is slow transfer of energy to the sounding board, generating a soft but near-constant "Sustain".
External links
- Five lectures on the acoustics of the piano
- A. H. Benade Sound Production in Pianos
- Robert W. Young, Inharmonicity of Plain Wire Piano Strings' The Journal of the Acoustical Society of America, vol 24 no. 3 (May 1952)
- "The Engineering of Concert Grand Pianos," by Richard Dain, FRENG
- D. Clausen, B. Hughes and W. Stuart "A design analysis of a Stuart and Sons grand piano frame"