Nome (mathematics)
Encyclopedia
In mathematics
, specifically the theory of elliptic functions, the nome is a special function and is given by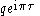
where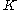
and 
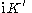are the quarter periods, and 
and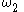 
are the fundamental pair of periods
. Notationally, the quarter periods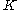
and 
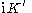are usually used only in the context of the Jacobian elliptic functions, whereas the half-periods 
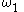and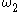 
are usually used only in the context of Weierstrass elliptic functions. Some authors, notably Apostol, use 
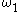and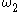 
to denote whole periods rather than half-periods.
The nome is frequently used as a value with which elliptic functions and modular forms can be described; on the other hand, it can also be thought of as function, because the quarter periods are functions of the elliptic modulus. This ambiguity occurs because for real values of the elliptic modulus, the quarter periods and thus the nome are uniquely determined.
The function
is sometimes called the half-period ratio because it is the ratio of the two half-periods 
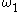and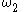 
of an elliptic function.
The complementary nome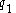
is given by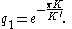
See the articles on quarter period and elliptic integral
s for additional definitions and relations on the nome.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, specifically the theory of elliptic functions, the nome is a special function and is given by
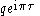
where
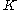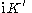and are the quarter periods, and and 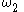are the fundamental pair of periodsFundamental pair of periods
In mathematics, a fundamental pair of periods is an ordered pair of complex numbers that define a lattice in the complex plane. This type of lattice is the underlying object with which elliptic functions and modular forms are defined....
. Notationally, the quarter periods
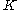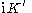and are usually used only in the context of the Jacobian elliptic functions, whereas the half-periods 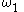and 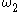are usually used only in the context of Weierstrass elliptic functions. Some authors, notably Apostol, use 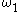and 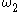to denote whole periods rather than half-periods.The nome is frequently used as a value with which elliptic functions and modular forms can be described; on the other hand, it can also be thought of as function, because the quarter periods are functions of the elliptic modulus. This ambiguity occurs because for real values of the elliptic modulus, the quarter periods and thus the nome are uniquely determined.
The function
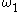is sometimes called the half-period ratio because it is the ratio of the two half-periods and 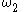of an elliptic function.The complementary nome
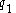is given by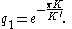See the articles on quarter period and elliptic integral
Elliptic integral
In integral calculus, elliptic integrals originally arose in connection with the problem of giving the arc length of an ellipse. They were first studied by Giulio Fagnano and Leonhard Euler...
s for additional definitions and relations on the nome.