Quarter period
Encyclopedia
In mathematics
, the quarter periods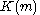
and 
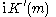are special functions that appear in the theory of elliptic functions.
The quarter periods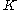
and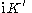 
are given by
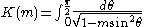
and
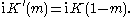
Note that when m is a real number,
, then both K and K′ are real numbers. By convention, K is called the real quarter period and iK′ is called the imaginary quarter period. Note that any one of the numbers m, K, K′, or K′/K uniquely determines the others.
These functions appear in the theory of Jacobian elliptic functions; they are called quarter periods because the elliptic functions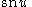
and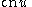 
are periodic functions with periods 
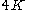and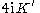 
.
Note that the quarter periods are essentially the elliptic integral
of the first kind, by making the substitution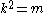
. In this case, one writes 
instead of 
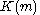, understanding the difference between the two depends notationally on whether 
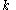or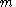 
is used. This notational difference has spawned a terminology to go with it:
The elliptic modulus can be expressed in terms of the quarter periods as
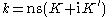
and
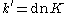
where ns and dn Jacobian elliptic functions.
The nome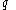
is given by
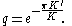
The complementary nome is given by
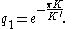
The real quarter period can be expressed as a Lambert series involving the nome:
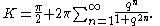
Additional expansions and relations can be found on the page for elliptic integral
s.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the quarter periods
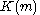and 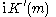are special functions that appear in the theory of elliptic functions.The quarter periods
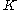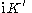and are given by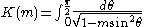and
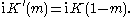
Note that when m is a real number,
, then both K and K′ are real numbers. By convention, K is called the real quarter period and iK′ is called the imaginary quarter period. Note that any one of the numbers m, K, K′, or K′/K uniquely determines the others.These functions appear in the theory of Jacobian elliptic functions; they are called quarter periods because the elliptic functions
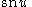and 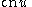are periodic functions with periods 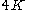and 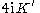.Note that the quarter periods are essentially the elliptic integral
Elliptic integral
In integral calculus, elliptic integrals originally arose in connection with the problem of giving the arc length of an ellipse. They were first studied by Giulio Fagnano and Leonhard Euler...
of the first kind, by making the substitution
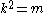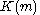. In this case, one writes instead of , understanding the difference between the two depends notationally on whethe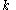r or 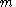is used. This notational difference has spawned a terminology to go with it:
-
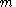is called the parameter -
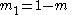is called the complementary parameter -
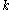is called the elliptic modulus -
is called the complementary elliptic modulus, where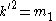 
-
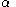the modular angle, where 
-
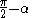the complementary modular angle. Note that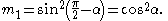
The elliptic modulus can be expressed in terms of the quarter periods as
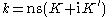
and
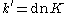
where ns and dn Jacobian elliptic functions.
The nome
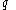is given by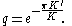The complementary nome is given by
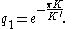
The real quarter period can be expressed as a Lambert series involving the nome:
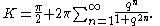
Additional expansions and relations can be found on the page for elliptic integral
Elliptic integral
In integral calculus, elliptic integrals originally arose in connection with the problem of giving the arc length of an ellipse. They were first studied by Giulio Fagnano and Leonhard Euler...
s.