
Mathematical modelling in epidemiology
Encyclopedia
It is possible to mathematically model the progress of most infectious diseases to discover the likely outcome of an epidemic
or to help manage them by vaccination
. This article uses some basic assumptions and some simple mathematics to find parameter
s for various infectious disease
s and to use those parameters to make useful calculations about the effects of a mass vaccination
programme.
, who in the early twentieth century applied the law of mass action to explain epidemic behaviour. Lowell Reed and Wade Hampton Frost developed the Reed-Frost epidemic model which described the relationship between susceptible, infected and immune individuals in a population.
S
A
L
when it can be sustained in a population without the need for external inputs. This means that, on average, each infected person is infecting exactly one other person (any more and the number of people infected will grow exponentially
and there will be an epidemic
, any less and the disease will die out). In mathematical terms, that is:

The basic reproduction number
(R0) of the disease, assuming everyone is susceptible, multiplied by the proportion of the population that is actually susceptible (S) must be one (since those who are not susceptible do not feature in our calculations as they cannot contract the disease). Notice that this relation means that for a disease to be in the endemic steady state, the higher the basic reproduction number, the lower the proportion of the population susceptible must be, and vice versa; a mathematical basis for a result that might have been intuitively obvious.
The first assumption (above) lets us say that everyone in the population lives to age L and then dies. If the average age of infection is A, then on average individuals younger than A are susceptible and those older than A are immune (or infectious). Thus the proportion of the population that is susceptible is given by:
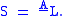
But the mathematical definition of the endemic steady state can be rearranged to give:
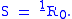
Therefore, since things equal to the same thing are equal to each other:
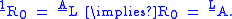
This provides a simple way to estimate the parameter R0 using easily available data.
For a population with an exponential age distribution
,
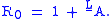
This allows for the basic reproduction number of a disease given A and L in either type of population distribution.
-pathogen
interactions. This has resulted in a growing body of research covering quantitative or theoretical studies of the population dynamics
, structure and evolution of infectious disease
s of plants and animals, including humans.
Specific topics in this area include:
level for the disease, then the disease can no longer persist in the population. Thus, if this level can be exceeded by vaccination, the disease can be eliminated. An example of this being successfully achieved worldwide is the global eradication of smallpox
, with the last wild case in 1977. Currently, the WHO
is carrying out a similar campaign of vaccination in an attempt to eradicate polio.
The herd immunity level will be denoted q. Recall that, for a stable state:
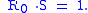
S will be (1 − q), since q is the proportion of the population that is immune and q + S must equal one (since in this simplified model, everyone is either susceptible or immune). Then:
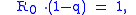
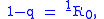
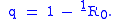
Remember that this is the threshold level. If the proportion of immune individuals exceeds this level due to a mass vaccination programme, the disease will die out.
We have just calculated the critical immunisation threshold (denoted qc). It is the minimum proportion of the population that must be immunised at birth (or close to birth) in order for the infection to die out in the population.
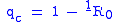
) the programme may not be able to exceed qc. Such a programme can, however, disturb the balance of the infection without eliminating it, often causing unforeseen problems.
Suppose that a proportion of the population q (where q < qc) is immunised at birth against an infection with R0>1. The vaccination
programme changes R0 to Rq where
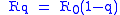
This change occurs simply because there are now fewer susceptibles in the population who can be infected. Rq is simply R0 minus those that would normally be infected but that cannot be now since they are immune.
As a consequence of this lower basic reproduction number, the average age of infection A will also change to some new value Aq in those who have been left unvaccinated.
Recall the relation that linked R0, A and L. Assuming that life expectancy has not changed, now:
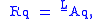
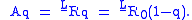
But R0 = L/A so:

Thus the vaccination programme will produce an increase in the average age of infection, another mathematical justification for a result that might have been intuitively obvious. Unvaccinated individuals now experience a reduced force of infection
due to the presence of the vaccinated group.
However, it is important to consider this effect when vaccinating against diseases which increase in severity with age. A vaccination programme against such a disease that does not exceed qc may cause more deaths and complications than there were before the programme was brought into force as individuals will be catching the disease later in life. These unforeseen outcomes of a vaccination programme are called perverse effects
.
Elimination
Eradication
Epidemic
In epidemiology, an epidemic , occurs when new cases of a certain disease, in a given human population, and during a given period, substantially exceed what is expected based on recent experience...
or to help manage them by vaccination
Vaccination
Vaccination is the administration of antigenic material to stimulate the immune system of an individual to develop adaptive immunity to a disease. Vaccines can prevent or ameliorate the effects of infection by many pathogens...
. This article uses some basic assumptions and some simple mathematics to find parameter
Parameter
Parameter from Ancient Greek παρά also “para” meaning “beside, subsidiary” and μέτρον also “metron” meaning “measure”, can be interpreted in mathematics, logic, linguistics, environmental science and other disciplines....
s for various infectious disease
Infectious disease
Infectious diseases, also known as communicable diseases, contagious diseases or transmissible diseases comprise clinically evident illness resulting from the infection, presence and growth of pathogenic biological agents in an individual host organism...
s and to use those parameters to make useful calculations about the effects of a mass vaccination
Vaccination
Vaccination is the administration of antigenic material to stimulate the immune system of an individual to develop adaptive immunity to a disease. Vaccines can prevent or ameliorate the effects of infection by many pathogens...
programme.
History
Early pioneers in infectious disease modelling were William Hamer and Ronald RossRonald Ross
Sir Ronald Ross KCB FRS was a British doctor who received the Nobel Prize for Physiology or Medicine in 1902 for his work on malaria. He was the first Indian-born person to win a Nobel Prize...
, who in the early twentieth century applied the law of mass action to explain epidemic behaviour. Lowell Reed and Wade Hampton Frost developed the Reed-Frost epidemic model which described the relationship between susceptible, infected and immune individuals in a population.
Concepts
R0, the basic reproduction numberBasic reproduction number
In epidemiology, the basic reproduction number of an infection is the mean number of secondary cases caused by an individual infected soon after disease introduction into a population with no pre-existing immunity to the disease in the absence of interventions to control...
- The average number of other individuals each infected individual will infect in a population that has no immunityImmune systemAn immune system is a system of biological structures and processes within an organism that protects against disease by identifying and killing pathogens and tumor cells. It detects a wide variety of agents, from viruses to parasitic worms, and needs to distinguish them from the organism's own...
to the disease.
S
- The proportion of the population who are susceptible to the disease (neither immune nor infected).
A
- The average age at which the disease is contracted in a given population.
L
- The average life expectancy in a given population.
Assumptions
Models are only as good as the assumptions on which they are based. If a model makes predictions which are out of line with observed results and the mathematics is correct, the initial assumptions must change to make the model useful.- Rectangular age distributionPopulation pyramidA population pyramid, also called an age structure diagram, is a graphical illustration that shows the distribution of various age groups in a population , which forms the shape of a pyramid when the population is growing...
: Typically found in developed countries where there is a low infant mortality and much of the population lives to the life expectancy. In developed countries this assumption is often well justified. - Homogeneous mixing of the population, i.e., individuals of the population under scrutiny assort and make contactTransmission risks and ratesTransmission of an infection requires three conditions:*an infectious individual*a susceptible individual*an effective contact between themAn effective contact is defined as any kind of contact between two individuals such that, if one individual is infectious and the other susceptible, then the...
at random and do not mix mostly in a smaller subgroup. This assumption is rarely justified, when dealing with a country such as the UK, because most people in London, say, only make contact with other Londoners. If we deal only with London, then there will be smaller subgroups such as the Turkish community or teenagers (just to give two examples) who will mix with each other more than people outside their group. However, homogeneous mixing is a standard assumption to make the mathematics tractable.
Endemic steady state
An infectious disease is said to be endemicEndemic (epidemiology)
In epidemiology, an infection is said to be endemic in a population when that infection is maintained in the population without the need for external inputs. For example, chickenpox is endemic in the UK, but malaria is not...
when it can be sustained in a population without the need for external inputs. This means that, on average, each infected person is infecting exactly one other person (any more and the number of people infected will grow exponentially
Exponential growth
Exponential growth occurs when the growth rate of a mathematical function is proportional to the function's current value...
and there will be an epidemic
Epidemic
In epidemiology, an epidemic , occurs when new cases of a certain disease, in a given human population, and during a given period, substantially exceed what is expected based on recent experience...
, any less and the disease will die out). In mathematical terms, that is:

The basic reproduction number
Basic reproduction number
In epidemiology, the basic reproduction number of an infection is the mean number of secondary cases caused by an individual infected soon after disease introduction into a population with no pre-existing immunity to the disease in the absence of interventions to control...
(R0) of the disease, assuming everyone is susceptible, multiplied by the proportion of the population that is actually susceptible (S) must be one (since those who are not susceptible do not feature in our calculations as they cannot contract the disease). Notice that this relation means that for a disease to be in the endemic steady state, the higher the basic reproduction number, the lower the proportion of the population susceptible must be, and vice versa; a mathematical basis for a result that might have been intuitively obvious.
The first assumption (above) lets us say that everyone in the population lives to age L and then dies. If the average age of infection is A, then on average individuals younger than A are susceptible and those older than A are immune (or infectious). Thus the proportion of the population that is susceptible is given by:
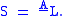
But the mathematical definition of the endemic steady state can be rearranged to give:
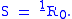
Therefore, since things equal to the same thing are equal to each other:
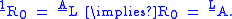
This provides a simple way to estimate the parameter R0 using easily available data.
For a population with an exponential age distribution
Population pyramid
A population pyramid, also called an age structure diagram, is a graphical illustration that shows the distribution of various age groups in a population , which forms the shape of a pyramid when the population is growing...
,
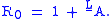
This allows for the basic reproduction number of a disease given A and L in either type of population distribution.
Infectious disease dynamics
In recent years it has become obvious that there is a need to accommodate the growing integration of quantitative methods with the increasing volume of data being generated on hostHost (biology)
In biology, a host is an organism that harbors a parasite, or a mutual or commensal symbiont, typically providing nourishment and shelter. In botany, a host plant is one that supplies food resources and substrate for certain insects or other fauna...
-pathogen
Pathogen
A pathogen gignomai "I give birth to") or infectious agent — colloquially, a germ — is a microbe or microorganism such as a virus, bacterium, prion, or fungus that causes disease in its animal or plant host...
interactions. This has resulted in a growing body of research covering quantitative or theoretical studies of the population dynamics
Population dynamics
Population dynamics is the branch of life sciences that studies short-term and long-term changes in the size and age composition of populations, and the biological and environmental processes influencing those changes...
, structure and evolution of infectious disease
Infectious disease
Infectious diseases, also known as communicable diseases, contagious diseases or transmissible diseases comprise clinically evident illness resulting from the infection, presence and growth of pathogenic biological agents in an individual host organism...
s of plants and animals, including humans.
Specific topics in this area include:
- transmissionTransmission (medicine)In medicine and biology, transmission is the passing of a communicable disease from an infected host individual or group to a conspecific individual or group, regardless of whether the other individual was previously infected...
, spread and control of infection - epidemiological networks
- spatial epidemiologySpatial epidemiologySpatial epidemiology is a subfield of health geography focused on the study of the spatial distribution of disease.-See also:General topics* Cluster * Complete spatial randomness* Geographic information system* Geographic information science...
- persistencePersistencePersistence may refer to:* Image persistence, in LCD monitors* Multidrug tolerance, a dormant, persistent state of a bacterial population* Persistence , the characteristic of data that outlives the execution of the program that created it...
of pathogens within hosts - intra-host dynamics
- immunoImmune systemAn immune system is a system of biological structures and processes within an organism that protects against disease by identifying and killing pathogens and tumor cells. It detects a wide variety of agents, from viruses to parasitic worms, and needs to distinguish them from the organism's own...
-epidemiology - virulenceVirulenceVirulence is by MeSH definition the degree of pathogenicity within a group or species of parasites as indicated by case fatality rates and/or the ability of the organism to invade the tissues of the host. The pathogenicity of an organism - its ability to cause disease - is determined by its...
- Strain (biology)Strain (biology)In biology, a strain is a low-level taxonomic rank used in three related ways.-Microbiology and virology:A strain is a genetic variant or subtype of a micro-organism . For example, a "flu strain" is a certain biological form of the influenza or "flu" virus...
structure and interactions - antigenic shiftAntigenic shiftAntigenic shift is the process by which two or more different strains of a virus, or strains of two or more different viruses, combine to form a new subtype having a mixture of the surface antigens of the two or more original strains...
- phylodynamicsPhylodynamicsPhylodynamics is a term coined to describe how pathogen genetic variation is affected by—and itself affects—influences such as host immunity, transmission and epidemic dynamics...
- pathogen population geneticsPopulation geneticsPopulation genetics is the study of allele frequency distribution and change under the influence of the four main evolutionary processes: natural selection, genetic drift, mutation and gene flow. It also takes into account the factors of recombination, population subdivision and population...
- evolutionEvolutionEvolution is any change across successive generations in the heritable characteristics of biological populations. Evolutionary processes give rise to diversity at every level of biological organisation, including species, individual organisms and molecules such as DNA and proteins.Life on Earth...
and spread of resistanceDrug resistanceDrug resistance is the reduction in effectiveness of a drug such as an antimicrobial or an antineoplastic in curing a disease or condition. When the drug is not intended to kill or inhibit a pathogen, then the term is equivalent to dosage failure or drug tolerance. More commonly, the term is used... - role of host genetic factors
- statistical and mathematical tools and innovations
- role and identification of infection reservoirs
Mathematics of mass vaccination
If the proportion of the population that is immune exceeds the herd immunityHerd immunity
Herd immunity describes a form of immunity that occurs when the vaccination of a significant portion of a population provides a measure of protection for individuals who have not developed immunity...
level for the disease, then the disease can no longer persist in the population. Thus, if this level can be exceeded by vaccination, the disease can be eliminated. An example of this being successfully achieved worldwide is the global eradication of smallpox
Smallpox
Smallpox was an infectious disease unique to humans, caused by either of two virus variants, Variola major and Variola minor. The disease is also known by the Latin names Variola or Variola vera, which is a derivative of the Latin varius, meaning "spotted", or varus, meaning "pimple"...
, with the last wild case in 1977. Currently, the WHO
Who
Who may refer to:* Who , an English-language pronoun* who , a Unix command* Who?, one of the Five Ws in journalism- Art and entertainment :* Who? , a 1958 novel by Algis Budrys...
is carrying out a similar campaign of vaccination in an attempt to eradicate polio.
The herd immunity level will be denoted q. Recall that, for a stable state:
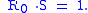
S will be (1 − q), since q is the proportion of the population that is immune and q + S must equal one (since in this simplified model, everyone is either susceptible or immune). Then:

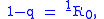
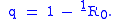
Remember that this is the threshold level. If the proportion of immune individuals exceeds this level due to a mass vaccination programme, the disease will die out.
We have just calculated the critical immunisation threshold (denoted qc). It is the minimum proportion of the population that must be immunised at birth (or close to birth) in order for the infection to die out in the population.
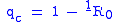
When mass vaccination cannot exceed the herd immunity
If the vaccine used is insufficiently effective or the required coverage cannot be reached (for example due to popular resistanceMMR vaccine controversy
The MMR vaccine controversy was a case of scientific misconduct which triggered a health scare. It followed the publication in 1998 of a paper in the medical journal The Lancet which presented apparent evidence that autism spectrum disorders could be caused by the MMR vaccine, an immunization...
) the programme may not be able to exceed qc. Such a programme can, however, disturb the balance of the infection without eliminating it, often causing unforeseen problems.
Suppose that a proportion of the population q (where q < qc) is immunised at birth against an infection with R0>1. The vaccination
Vaccination
Vaccination is the administration of antigenic material to stimulate the immune system of an individual to develop adaptive immunity to a disease. Vaccines can prevent or ameliorate the effects of infection by many pathogens...
programme changes R0 to Rq where
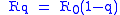
This change occurs simply because there are now fewer susceptibles in the population who can be infected. Rq is simply R0 minus those that would normally be infected but that cannot be now since they are immune.
As a consequence of this lower basic reproduction number, the average age of infection A will also change to some new value Aq in those who have been left unvaccinated.
Recall the relation that linked R0, A and L. Assuming that life expectancy has not changed, now:
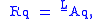
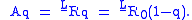
But R0 = L/A so:

Thus the vaccination programme will produce an increase in the average age of infection, another mathematical justification for a result that might have been intuitively obvious. Unvaccinated individuals now experience a reduced force of infection
Force of infection
In epidemiology, force of infection is the rate at which susceptible individuals become infected by an infectious disease. Because it takes account of susceptibility it can be used to compare the rate of transmission between different groups of the population for the same infectious disease, or...
due to the presence of the vaccinated group.
However, it is important to consider this effect when vaccinating against diseases which increase in severity with age. A vaccination programme against such a disease that does not exceed qc may cause more deaths and complications than there were before the programme was brought into force as individuals will be catching the disease later in life. These unforeseen outcomes of a vaccination programme are called perverse effects
Perverse effects of vaccination
Perverse effects of vaccination occur when a vaccination program causes more harm than it cures. This can happen if too few are vaccinated, allowing the disease to spread, although more slowly than in an unvaccinated population...
.
When mass vaccination exceeds the herd immunity
If a vaccination programme causes the proportion of immune individuals in a population to exceed the critical threshold for a significant length of time, transmission of the infectious disease in that population will gradually come to a halt. This is known as elimination of the infection and is different from eradication.Elimination
- Interruption of endemic transmission of an infectious disease, which occurs if each infected individual infects less than one other and is achieved by maintaining vaccination coverage to keep the proportion of immune individuals above the critical immunisation threshold.
Eradication
- Reduction of infective organisms in the wild worldwide to zero. So far, this has only been achieved for smallpoxSmallpoxSmallpox was an infectious disease unique to humans, caused by either of two virus variants, Variola major and Variola minor. The disease is also known by the Latin names Variola or Variola vera, which is a derivative of the Latin varius, meaning "spotted", or varus, meaning "pimple"...
and rinderpestRinderpestRinderpest was an infectious viral disease of cattle, domestic buffalo, and some other species of even-toed ungulates, including buffaloes, large antelopes and deer, giraffes, wildebeests and warthogs. After a global eradication campaign, the last confirmed case of rinderpest was diagnosed in 2001...
. To get to eradication, elimination in all world regions must first be progressed through.
See also
- Compartmental models in epidemiologyCompartmental models in epidemiologyIn order to model the progress of an epidemic in a large population, comprising many different individuals in various fields, the population diversity must be reduced to a few key characteristics which are relevant to the infection under consideration...
- Ecosystem modelEcosystem modelAn ecosystem model is an abstract, usually mathematical, representation of an ecological system , which is studied to gain a deeper understanding of the real system.Ecosystem models are formed by combining known ecological relations An ecosystem model is an abstract, usually mathematical,...
- EndemicEndemic (epidemiology)In epidemiology, an infection is said to be endemic in a population when that infection is maintained in the population without the need for external inputs. For example, chickenpox is endemic in the UK, but malaria is not...
- Epidemic modelEpidemic modelAn Epidemic model is a simplified means of describing the transmission of communicable disease through individuals.-Introduction:The outbreak and spread of disease has been questioned and studied for many years...
- Force of infectionForce of infectionIn epidemiology, force of infection is the rate at which susceptible individuals become infected by an infectious disease. Because it takes account of susceptibility it can be used to compare the rate of transmission between different groups of the population for the same infectious disease, or...
- Landscape epidemiologyLandscape epidemiologyLandscape epidemiology draws some of its roots from the field of landscape ecology. Just as the discipline of landscape ecology is concerned with analyzing both pattern and process in ecosystems across time and space, landscape epidemiology can be used to analyze both risk patterns and...
- Perverse effects of vaccinationPerverse effects of vaccinationPerverse effects of vaccination occur when a vaccination program causes more harm than it cures. This can happen if too few are vaccinated, allowing the disease to spread, although more slowly than in an unvaccinated population...
- Risk factorRisk factorIn epidemiology, a risk factor is a variable associated with an increased risk of disease or infection. Sometimes, determinant is also used, being a variable associated with either increased or decreased risk.-Correlation vs causation:...
- Sexual networkSexual networkA sexual network is a social network that is defined by the sexual relationships within a set of individuals.-Studies and discoveries:Like other forms of social networks, sexual networks can be formally studied using the mathematics of graph theory and network theory.Recent epidemiological studies ...
- Susceptible
- Transmission risks and ratesTransmission risks and ratesTransmission of an infection requires three conditions:*an infectious individual*a susceptible individual*an effective contact between themAn effective contact is defined as any kind of contact between two individuals such that, if one individual is infectious and the other susceptible, then the...
Further reading
- "Infectious Diseases of Humans" Roy M. Anderson and Robert M. May (ISBN 0-19-854040-X)
- An Introduction to Infectious Disease Modelling by Emilia Vynnycky and Richard G White. An introductory book on infectious disease modelling and its applications.
- "Smallpox and its eradication" Jenner
- Cross-scale influences on epidemiological dynamics: from genes to ecosystems: A theme issue of J. R. Soc. Interface which is free to access.
External links
- Scientific American (March 2005) If Smallpox Strikes Portland ... (an article about "Episims")
- Institute for Emerging Infections, University of Oxford
- Center for Infectious Disease Dynamics, The Pennsylvania State University
- Cambridge Infectious Diseases Consortium
- Journal of the Royal Society Interface
- Models of Infectious Disease Agent Study
- Infectious Disease Modeling: Measles Virus
- Model-Builder: Interactive (GUI-based) software to build, simulate, and analyze ODE models.
- AnyLogic: Enables both Agent Based and System Dynamics modelling and simulation of disease spread.
- GLEaMviz Simulator: Enables simulation of emerging infectious diseases spreading across the world.

