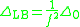For Liouville's equation in quantum mechanics, see Density operator#Von Neumann equation.

In differential geometry, Liouville's equation, named after Joseph Liouville

Joseph Liouville

- Life and work :Liouville graduated from the École Polytechnique in 1827. After some years as an assistant at various institutions including the Ecole Centrale Paris, he was appointed as professor at the École Polytechnique in 1838...

, is the nonlinear ordinary differential equation satisfied by the conformal factor f of a metric 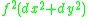on a surface of constant Gaussian curvature

Gaussian curvature

In differential geometry, the Gaussian curvature or Gauss curvature of a point on a surface is the product of the principal curvatures, κ1 and κ2, of the given point. It is an intrinsic measure of curvature, i.e., its value depends only on how distances are measured on the surface, not on the way...

 K:


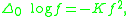
where 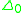is the flat Laplace operator

Laplace operator

In mathematics the Laplace operator or Laplacian is a differential operator given by the divergence of the gradient of a function on Euclidean space. It is usually denoted by the symbols ∇·∇, ∇2 or Δ...

.


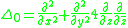
Liouville's equation typically appears in differential geometry books under the heading isothermal coordinates

Isothermal coordinates

In mathematics, specifically in differential geometry, isothermal coordinates on a Riemannian manifoldare local coordinates where the metric isconformal to the Euclidean metric...

. This term refers to the coordinates x,y, while f can be described as the conformal factor with respect to the flat metric (sometimes the square 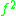is referred to as the conformal factor, instead of f itself).

Replacing f using 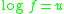, we obtain another commonly found form of the same equation: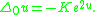

Laplace-Beltrami operator

In a more invariant fashion, the equation can be written in terms of the intrinsic Laplace-Beltrami operator

Laplace-Beltrami operator

In differential geometry, the Laplace operator, named after Pierre-Simon Laplace, can be generalized to operate on functions defined on surfaces in Euclidean space and, more generally, on Riemannian and pseudo-Riemannian manifolds. This more general operator goes by the name Laplace–Beltrami...

as follows:

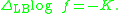
Relation to Gauss–Codazzi equations

Liouville's equation is a consequence of the Gauss–Codazzi equations

Gauss–Codazzi equations

In Riemannian geometry, the Gauss–Codazzi–Mainardi equations are fundamental equations in the theory of embedded hypersurfaces in a Euclidean space, and more generally submanifolds of Riemannian manifolds...

 when the metric is written in isothermal coordinates

Isothermal coordinates

In mathematics, specifically in differential geometry, isothermal coordinates on a Riemannian manifoldare local coordinates where the metric isconformal to the Euclidean metric...

.

General solution

In a simply connected domain the general solution is given by

where 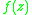is a locally univalent meromorphic function

Meromorphic function

In complex analysis, a meromorphic function on an open subset D of the complex plane is a function that is holomorphic on all D except a set of isolated points, which are poles for the function...

and 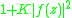when 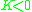.