Ice Ih
Encyclopedia
Ice
Ice is water frozen into the solid state. Usually ice is the phase known as ice Ih, which is the most abundant of the varying solid phases on the Earth's surface. It can appear transparent or opaque bluish-white color, depending on the presence of impurities or air inclusions...
, or frozen water. Virtually all ice in the biosphere
Biosphere
The biosphere is the global sum of all ecosystems. It can also be called the zone of life on Earth, a closed and self-regulating system...
is ice Ih, with the exception only of a small amount of ice Ic
Ice Ic
Ice Ic is a metastable cubic crystalline variant of ice. The oxygen atoms are arranged in a diamond structure. It is produced at temperatures between 130 and 220 K , and can exist up to 240 K, when it transforms into ice Ih. It may occasionally be present in the upper atmosphere.Ordinary water ice...
which is occasionally present in the upper atmosphere. Ice Ih exhibits many peculiar properties which are relevant to the existence of life and regulation of global climate.
Ice Ih is stable down to -200 C and can exist at pressures up to 0.2 GPa
Pascal (unit)
The pascal is the SI derived unit of pressure, internal pressure, stress, Young's modulus and tensile strength, named after the French mathematician, physicist, inventor, writer, and philosopher Blaise Pascal. It is a measure of force per unit area, defined as one newton per square metre...
. The crystal structure is characterized by hexagonal symmetry and near tetrahedral bonding angles.
Physical properties
Ice Ih has a densityDensity
The mass density or density of a material is defined as its mass per unit volume. The symbol most often used for density is ρ . In some cases , density is also defined as its weight per unit volume; although, this quantity is more properly called specific weight...
less than liquid water, of 0.917 g/cm³, due
to the extremely low density of its crystal lattice. The density of ice Ih increases with decreasing temperature (density of ice at -180 °C is 0.9340 g/cm³).
The latent heat of melting is 5987 J/mol, and its latent heat of sublimation is 50911 J/mol.
The high latent heat of sublimation is principally indicative of the strength of
the hydrogen bond
Hydrogen bond
A hydrogen bond is the attractive interaction of a hydrogen atom with an electronegative atom, such as nitrogen, oxygen or fluorine, that comes from another molecule or chemical group. The hydrogen must be covalently bonded to another electronegative atom to create the bond...
s in the crystal lattice. The latent heat
of melting is much smaller partly because water near 0 °C is very strongly
H-bonded already.
The refractive index of ice Ih is 1.31.
Crystal structure
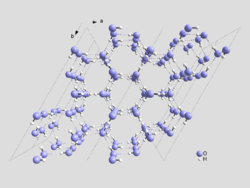
Crystal structure
In mineralogy and crystallography, crystal structure is a unique arrangement of atoms or molecules in a crystalline liquid or solid. A crystal structure is composed of a pattern, a set of atoms arranged in a particular way, and a lattice exhibiting long-range order and symmetry...
of ordinary ice was first proposed by Linus Pauling
Linus Pauling
Linus Carl Pauling was an American chemist, biochemist, peace activist, author, and educator. He was one of the most influential chemists in history and ranks among the most important scientists of the 20th century...
in 1935. The structure of ice Ih is roughly one of crinkled planes composed of tessellating
Tessellation
A tessellation or tiling of the plane is a pattern of plane figures that fills the plane with no overlaps and no gaps. One may also speak of tessellations of parts of the plane or of other surfaces. Generalizations to higher dimensions are also possible. Tessellations frequently appeared in the art...
hexagonal rings, with an oxygen
Oxygen
Oxygen is the element with atomic number 8 and represented by the symbol O. Its name derives from the Greek roots ὀξύς and -γενής , because at the time of naming, it was mistakenly thought that all acids required oxygen in their composition...
atom on each vertex, and the edges of the rings formed by hydrogen bond
Hydrogen bond
A hydrogen bond is the attractive interaction of a hydrogen atom with an electronegative atom, such as nitrogen, oxygen or fluorine, that comes from another molecule or chemical group. The hydrogen must be covalently bonded to another electronegative atom to create the bond...
s. The planes alternate in an ABAB pattern, with B planes being reflections of the A planes along the same axes as the planes themselves. The distance between oxygen atoms along each bond is about 275 pm
Picometre
A picometre is a unit of length in the metric system, equal to one trillionth, i.e. of a metre, which is the current SI base unit of length...
and is the same between any two bonded oxygen atoms in the lattice. The angle between bonds in the crystal lattice is very close to the tetrahedral
Tetrahedron
In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids...
angle of 109.5° which is also quite close to the angle between hydrogen atoms in the water molecule (in the gas phase), which is 105°. This tetrahedral bonding angle of the water molecule essentially accounts for the unusually low density of the crystal lattice -- it is beneficial for the lattice to be arranged with tetrahedral angles even though there is an energy penalty in the increased volume of the crystal lattice. As a result, the large hexagonal rings leave almost enough room for another water molecule to exist inside. This gives naturally occurring ice its unique property of being less dense than its liquid form. The tetrahedral-angled hydrogen-bonded hexagonal rings are also the mechanism which causes liquid water to be most dense at 4 °C. Close to 0 °C, tiny hexagonal ice Ih-like lattices form in liquid water, with greater frequency closer to 0 °C. This effect decreases the density of the water, causing it to be most dense at 4 °C when the structures form infrequently.
Proton disorder
The protons (hydrogenHydrogen
Hydrogen is the chemical element with atomic number 1. It is represented by the symbol H. With an average atomic weight of , hydrogen is the lightest and most abundant chemical element, constituting roughly 75% of the Universe's chemical elemental mass. Stars in the main sequence are mainly...
atoms) in the crystal lattice lie very nearly along the hydrogen bonds, and in such a way that each water molecule is preserved. This means that each oxygen atom in the lattice has two protons adjacent to it, at about 101 pm along the 275 pm length of the bond. The crystal lattice allows a substantial amount of disorder in the positions of the protons frozen into the structure as it cools to absolute zero. As a result, the crystal structure contains some residual entropy
Residual entropy
Residual entropy is small amount of entropy which is present even after a substance is cooled arbitrarily close to absolute zero. It occurs if a material can exist in many different microscopic states when cooled to absolute zero...
inherent to the lattice and determined by the number of possible configurations of proton positions which can be formed while still maintaining the requirement for each oxygen atom to have only two protons in closest proximity, and each H-bond joining two oxygen atoms having only one proton. This residual entropy S0 is equal to 3.5 J mol−1 K−1. There are various ways of approximating this number from first principles. Assuming a given N water molecules each has 6 possible arrangements this yields 6N possible combinations. Given random orientations of molecules, a given bond will have only a ½ chance that it has exactly one proton, or in other words, each molecule has a ¼ chance that its protons lie on bonds containing exactly one proton, leaving a total number of
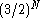possible valid combinations. Using Boltzmann's principle, we find that 
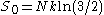, where 
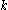is the Boltzmann constant, which yields a value of 3.37 J mol−1 K−1, a value very close to the measured value. More complexmethods can be employed to better approximate the exact number of possible configurations, and achieve results closer to measured values.
By contrast, the structure of ice II
Ice II
Ice II is a rhombohedral crystalline form of ice with highly ordered structure. It is formed from ice Ih by compressing it at temperature of 198 K at 300 MPa or by decompressing ice V. When heated it undergoes transformation to ice III....
is very proton-ordered, which helps to explain the entropy change of 3.22 J/mol when the crystal structure changes to that of ice II. Also, ice XI, an orthorhombic, proton-ordered form of ice Ih, is considered the most stable form.