General Formal Ontology
Encyclopedia
The general formal ontology (GFO) is an upper ontology
integrating processes and objects. GFO has been developed by Heinrich Herre, Barbara Heller and collaborators (research group Onto-Med) in Leipzig
. Although GFO provides one taxonomic tree, different axiom systems may be chosen for its modules. In this sense, GFO provides a framework for building custom, domain-specific ontologies. GFO exhibits a three-layered meta-ontological architecture consisting of an abstract top level, an abstract core level, and a basic level.
Primarily, the ontology GFO:
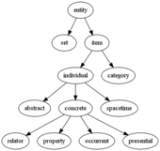
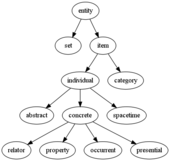GFO (General Formal ontology) draws a fundamental distinction between concrete entities, categories and sets. Sets are described by an axiomatic fragment of set theory
of Zermelo-Fraenkel, although fragments of anti-foundation axiom set theories such as ZF-AFA
are considered.
Concrete entities are entities which are in time
and space
, while categories have universal
character.
Categories in GFO are further divided in immanent universals, conceptual structures and symbolic structures. Immanent universals are so-called Aristotlian
universals
, in the sense that they are considered in re. This means, that these universals exist in all the entities which instantiate an immanent universal, independent of an observer. An example of an immanent universal could be APPLE. The universal APPLE exists in all apples, independent of perception
by an agent.
Concept
ual structures are mental representations of entities or universals, and they exist in an agent's mind
. For example, the individual representation of the (linguistic) term "apple" inside an agent's mind (determined by the agent's experience, knowledge and belief, etc.).
Symbolic structures are signs which may be instantiated by token
s. They have the property to stand for something beyond themselves. An example is the physical pattern "apple", which instantiates the "APPLE" symbolic structure.
. For time, time-intervals, called chronoids, are taken as primitive. Existentially dependent on these time-intervals are time-boundaries. Time-boundaries of different time-intervals may coincide. This notion of coincidence is equivalent to a formalization of time-based on the meets relation (due to Allen and Hayes).
Connected three-dimensional parts of space are called "topoids". As chronoids, topoids may coincide at a two-dimensional boundary. This boundary may coincide with other (two-dimensional) boundaries at a one-dimensional boundary, and so on.
and object
s. Processes unfold in time, they have temporal parts. Objects (called presentials) have no temporal parts, and may only exist on time-boundaries. Presentials are dependent on processes. This can be seen as a derivation of the dependency-relations in the formalization of time: processes are always framed by a chronoid; and as time-boundaries are dependent on chronoids, so are presentials dependent on processes.
DOLCE and other ontologies face the problem of "identity": how is it possible to model the persistence of an object through time. In GFO, this problem is made explicit: all presentials explicitly exist only at a single time-boundary; persistence is modelled by a special type of category, a persistent.
Upper ontology (computer science)
In information science, an upper ontology is an ontology which describes very general concepts that are the same across all knowledge domains. The most important function of an upper ontology is to support very broad semantic interoperability between a large number of ontologies accessible...
integrating processes and objects. GFO has been developed by Heinrich Herre, Barbara Heller and collaborators (research group Onto-Med) in Leipzig
Leipzig
Leipzig Leipzig has always been a trade city, situated during the time of the Holy Roman Empire at the intersection of the Via Regia and Via Imperii, two important trade routes. At one time, Leipzig was one of the major European centres of learning and culture in fields such as music and publishing...
. Although GFO provides one taxonomic tree, different axiom systems may be chosen for its modules. In this sense, GFO provides a framework for building custom, domain-specific ontologies. GFO exhibits a three-layered meta-ontological architecture consisting of an abstract top level, an abstract core level, and a basic level.
Primarily, the ontology GFO:
- includes objects as well as processes and both are integrated into one coherent system,
- includes levels of reality,
- is designed to support interoperability by principles of ontological mapping and reduction,
- contains several novel ontological modules, in particular, a module for functions and a module for roles, and
- is designed for applications, firstly in medical, biological, and biomedical areas, but also in the fields of economics and sociology.
Taxonomic tree of GFO
Set theory
Set theory is the branch of mathematics that studies sets, which are collections of objects. Although any type of object can be collected into a set, set theory is applied most often to objects that are relevant to mathematics...
of Zermelo-Fraenkel, although fragments of anti-foundation axiom set theories such as ZF-AFA
Non-well-founded set theory
Non-well-founded set theories are variants of axiomatic set theory which allow sets to contain themselves and otherwise violate the rule of well-foundedness...
are considered.
Concrete entities are entities which are in time
Time
Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects....
and space
Space
Space is the boundless, three-dimensional extent in which objects and events occur and have relative position and direction. Physical space is often conceived in three linear dimensions, although modern physicists usually consider it, with time, to be part of a boundless four-dimensional continuum...
, while categories have universal
Universal (metaphysics)
In metaphysics, a universal is what particular things have in common, namely characteristics or qualities. In other words, universals are repeatable or recurrent entities that can be instantiated or exemplified by many particular things. For example, suppose there are two chairs in a room, each of...
character.
Categories
The common property of all categories is, that they can be predicated of an entity.Categories in GFO are further divided in immanent universals, conceptual structures and symbolic structures. Immanent universals are so-called Aristotlian
Aristotle
Aristotle was a Greek philosopher and polymath, a student of Plato and teacher of Alexander the Great. His writings cover many subjects, including physics, metaphysics, poetry, theater, music, logic, rhetoric, linguistics, politics, government, ethics, biology, and zoology...
universals
Universal (metaphysics)
In metaphysics, a universal is what particular things have in common, namely characteristics or qualities. In other words, universals are repeatable or recurrent entities that can be instantiated or exemplified by many particular things. For example, suppose there are two chairs in a room, each of...
, in the sense that they are considered in re. This means, that these universals exist in all the entities which instantiate an immanent universal, independent of an observer. An example of an immanent universal could be APPLE. The universal APPLE exists in all apples, independent of perception
Perception
Perception is the process of attaining awareness or understanding of the environment by organizing and interpreting sensory information. All perception involves signals in the nervous system, which in turn result from physical stimulation of the sense organs...
by an agent.
Concept
Concept
The word concept is used in ordinary language as well as in almost all academic disciplines. Particularly in philosophy, psychology and cognitive sciences the term is much used and much discussed. WordNet defines concept: "conception, construct ". However, the meaning of the term concept is much...
ual structures are mental representations of entities or universals, and they exist in an agent's mind
Mind
The concept of mind is understood in many different ways by many different traditions, ranging from panpsychism and animism to traditional and organized religious views, as well as secular and materialist philosophies. Most agree that minds are constituted by conscious experience and intelligent...
. For example, the individual representation of the (linguistic) term "apple" inside an agent's mind (determined by the agent's experience, knowledge and belief, etc.).
Symbolic structures are signs which may be instantiated by token
Token
A token is an object of value, and may refer to:* In logic, computational linguistics, and information retrieval, a token is an instance of a type; see Type-token distinction...
s. They have the property to stand for something beyond themselves. An example is the physical pattern "apple", which instantiates the "APPLE" symbolic structure.
Space and time
GFO uses a theory of space and time which is inspired by the philosophy of BrentanoBrentano
There are some famous people named Brentano or von Brentano:* Antonie Brentano* August Brentano, bookseller* Bernard von Brentano, novelist* Christian Brentano* Clemens Brentano, poet and novelist, brother of Bettina von Arnim There are some famous people named Brentano or von Brentano:* Antonie...
. For time, time-intervals, called chronoids, are taken as primitive. Existentially dependent on these time-intervals are time-boundaries. Time-boundaries of different time-intervals may coincide. This notion of coincidence is equivalent to a formalization of time-based on the meets relation (due to Allen and Hayes).
Connected three-dimensional parts of space are called "topoids". As chronoids, topoids may coincide at a two-dimensional boundary. This boundary may coincide with other (two-dimensional) boundaries at a one-dimensional boundary, and so on.
Processes and objects
GFO distinguishes processesProcess (philosophy)
In philosophy and systems theory, basic processes, or logical homologies as they were termed by Ludwig von Bertalanffy, are unifying principles which operate in many different systemic contexts. For example, feedback is a principle that figures prominently in the science of cybernetics...
and object
Object (philosophy)
An object in philosophy is a technical term often used in contrast to the term subject. Consciousness is a state of cognition that includes the subject, which can never be doubted as only it can be the one who doubts, and some object or objects that may or may not have real existence without...
s. Processes unfold in time, they have temporal parts. Objects (called presentials) have no temporal parts, and may only exist on time-boundaries. Presentials are dependent on processes. This can be seen as a derivation of the dependency-relations in the formalization of time: processes are always framed by a chronoid; and as time-boundaries are dependent on chronoids, so are presentials dependent on processes.
DOLCE and other ontologies face the problem of "identity": how is it possible to model the persistence of an object through time. In GFO, this problem is made explicit: all presentials explicitly exist only at a single time-boundary; persistence is modelled by a special type of category, a persistent.