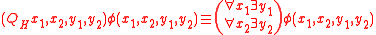Dependence logic
Encyclopedia
Dependence logic is a logical formalism, created by Jouko Väänänen, which adds dependence atoms to the language of first-order logic. A dependence atom is an expression of the form 
, where 
are terms, and corresponds to the statement that the value of 
is functionally dependent
on the values of
.
Dependence logic is a logic of imperfect information, like branching quantifier logic
or independence-friendly logic
: in other words, its game theoretic semantics
can be obtained from that of first-order logic by restricting the availability of information to the players, thus allowing for non-linearly ordered patterns of dependence and independence between variables. However, dependence logic differs from these logics in that it separates the notions of dependence and independence from the notion of quantification.
σ = (Sfunc, Srel, ar), the set of all well-formed dependence logic formulas is defined according to the following rules:
Nothing else is an atomic formula of dependence logic.
Relational and equality atoms are also called first order atoms.
of dependence logic and their respective sets of free variables 
are defined as follows:
Nothing is a dependence logic formula unless it can be obtained through a finite number of applications of these four rules.
A formula
such that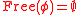 
is a sentence of dependence logic.
Therefore,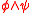
is taken as a shorthand for 
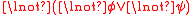, and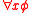 
is taken as a shorthand for 
.
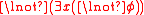' compositional semantics for IF logic
. There exist equivalent game-theoretic semantics for dependence logic, both in terms of imperfect information games and in terms of perfect information games.
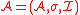be a first-order structure
and let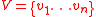
be a finite set of variables. Then a team over 
with domain 
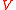is a set of assignments over 
with domain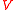 
, that is, a set of functions 
from 
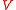to 
.
It may be helpful to visualize such a team as a database relation
with attributes
and with only one data type, corresponding to the domain 
of the structure: for example, if the team 
consists of four assignments 
with domain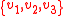 
then one may represent it as the relation
and 
between structures, teams and formulas.
Given a structure
, a team 
over it and a dependence logic formula 
whose free variables
are contained in the domain of
, if 
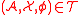we say that 
is a trump for 
in 
, and we write that 
; and analogously, if 
we say that 
is a cotrump for 
in 
, and we write that 
.
If
one can also say that 
is positively satisfied by 
in 
, and if instead 
one can say that 
is negatively satisfied by 
in 
.
The necessity of considering positive and negative satisfaction separately is a consequence of the fact that in dependence logic, as in the logic of branching quantifiers
or in IF logic
, the law of the excluded middle does not hold; alternatively, one may assume that all formulas are in negation normal form, using De Morgan's relations in order to define universal quantification and conjunction from existential quantification and disjunction respectively, and consider positive satisfaction alone.
Given a sentence
, we say that 
is true in 
if and only if 
, and we say that 
is false in 
if and only if 
.
's satisfiability relation for first-order formulas, the positive and negative satisfiability relations of the team semantics for dependence logic are defined by structural induction
over the formulas of the language. Since the negation operator interchanges positive and negative satisfiability, the two inductions corresponding to
and 
need to be performed simultaneously:
of first-order logic: in other words, for every first order sentence
and structure 
we have that 
if and only if 
is true in 
according to the usual first order semantics. Furthemore, for any first order formula 
, 
if and only if all assignments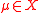 
satisfy 
in 
according to the usual first order semantics.
However, dependence logic is strictly more expressive than first order logic: for example, the sentence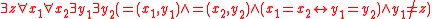
is true in a model
if and only if the domain of this model is infinite, even though no first order formula 
has this property.
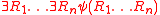
where
does not contain second order quantifiers.
Conversely, every second-order sentence in the above form is equivalent to some dependence logic sentence.
As for open formulas, dependence logic corresponds to the downwards monotone fragment of existential second order logic, in the sense that a nonempty class of teams is definable by a dependence logic formula if and only if the corresponding class of relations is downwards monotone and definable by an existential second order formula.
is equivalent to the dependence logic sentence
, in the sense that the former expression is true in a model if and only if the latter expression is true.
Conversely, any dependence logic sentence is equivalent to some sentence in the logic of branching quantifiers, since all existential second order sentences are expressible in branching quantifier logic.
However, the issue is subtler when it comes to open formulas. Translations between IF logic and dependence logic formulas, and vice versa, exist as long as the domain of the team is fixed: in other words, for all sets of variables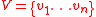
and all IF logic formulas 
with free variables in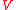 
there exists a dependence logic formula 
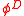such that
for all structures
and for all teams 
with domain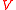 
, and conversely, for every dependence logic formula 
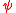with free variables in 
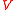there exists an IF logic formula 
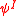such that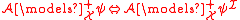
for all structures
and for all teams 
with domain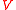 
. These translations cannot be compositional.
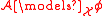and 
then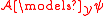 
. Furthermore, the empty team (but not the team containing the empty assignment) satisfies all formulas of Dependence Logic, both positively and negatively.
The law of the excluded middle fails in dependence logic: for example, the formula
is neither positively nor negatively satisfied by the team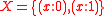 
. Furthermore, disjunction is not idempotent and does not distribute over conjunction.
Both the compactness theorem
and the Löwenheim-Skolem theorem are true for dependence logic. Craig's interpolation theorem
also holds, but, due to the nature of negation in dependence logic, in a slightly modified formulation: if two dependence logic formulas
and 
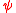are contradictory, that is, it is never the case that both 
and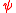 
hold in the same model, then there exists a first order sentence 
in the common language of the two sentences such that 
implies 
and 
is contradictory with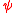 
.
As IF logic, Dependence logic can define its own truth operator: more precisely, there exists a formula
such that for every sentence 
of dependence logic and all models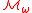 
which satisfy Peano's axioms, if 
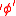is the Gödel number of 
then
This does not contradict Tarski's undefinability theorem, since the negation of dependence logic is not the usual contradictory one.
, the model checking problem for dependence logic is NP-complete
. Furthermore, its inconsistency problem is semidecidable, and in fact equivalent to the inconsistency problem for first-order logic.
However, the decision problem for dependence logic is non-arithmetical
, and is in fact complete with respect to the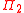
class of the Levy hierarchy.
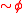. Its expressive power is equivalent to that of full second order logic.
, thus obtaining modal dependence logic.
, whose name derives from the similarity between its definition and that of the implication of intuitionistic logic
, can be defined as follows:
Intuitionistic dependence logic, that is, dependence logic supplemented with the intuitionistic implication, is equivalent to second-order logic.
, where are terms, and corresponds to the statement that the value of is functionally dependentFunctional dependency
A functional dependency is a constraint between two sets of attributes in a relation from a database.Given a relation R, a set of attributes X in R is said to functionally determine another attribute Y, also in R, if, and only if, each X value is associated with precisely one Y value...
on the values of
.Dependence logic is a logic of imperfect information, like branching quantifier logic
Branching quantifier
In logic a branching quantifier, also called a Henkin quantifier, finite partially ordered quantifier or even nonlinear quantifier, is a partial ordering\langle Qx_1\dots Qx_n\rangle...
or independence-friendly logic
Independence-friendly logic
Independence-friendly logic , proposed by Jaakko Hintikka and Gabriel Sandu, aims at being a more natural and intuitive alternative to classical first-order logic . IF logic is characterized by branching quantifiers...
: in other words, its game theoretic semantics
Game semantics
Game semantics is an approach to formal semantics that grounds the concepts of truth or validity on game-theoretic concepts, such as the existence of a winning strategy for a player, somewhat resembling Socratic dialogues or medieval theory of Obligationes. In the late 1950s Paul Lorenzen was the...
can be obtained from that of first-order logic by restricting the availability of information to the players, thus allowing for non-linearly ordered patterns of dependence and independence between variables. However, dependence logic differs from these logics in that it separates the notions of dependence and independence from the notion of quantification.
Syntax
The syntax of dependence logic is an extension of that of first-order logic. For a fixed signatureSignature (logic)
In logic, especially mathematical logic, a signature lists and describes the non-logical symbols of a formal language. In universal algebra, a signature lists the operations that characterize an algebraic structure. In model theory, signatures are used for both purposes.Signatures play the same...
σ = (Sfunc, Srel, ar), the set of all well-formed dependence logic formulas is defined according to the following rules:
Terms
Terms in dependence logic are defined precisely as in first-order logic.Atomic formulas
There are three types of atomic formulas in dependence logic:- A relational atom is an expression of the form
for any n-ary relation 
in our signature and for any n-uple of terms 
; - An equality atom is an expression of the form
, for any two terms 
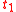and 
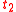; - A dependence atom is an expression of the form
, for any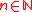 
and for any n-uple of terms 
.
Nothing else is an atomic formula of dependence logic.
Relational and equality atoms are also called first order atoms.
Complex formulas and sentences
For a fixed signature σ, the set of all formulas of dependence logic and their respective sets of free variables are defined as follows:- Any atomic formula
is a formula, and 
is the set of all variables occurring in it; - If
is a formula, so is 
and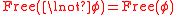 
; - If
and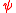 
are formulas, so is 
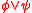and 
; - If
is a formula and 
is a variable, 
is also a formula and 
.
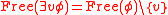Nothing is a dependence logic formula unless it can be obtained through a finite number of applications of these four rules.
A formula
such that 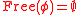is a sentence of dependence logic.Conjunction and universal quantification
In the above presentation of the syntax of dependence logic, conjunction and universal quantification are not treated as primitive operators; rather, they are defined in terms of disjunction and negation and existential quantification respectively, by means of De Morgan's Laws.Therefore,
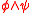is taken as a shorthand for 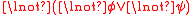, and 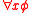is taken as a shorthand for 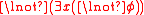.Semantics
The team semantics for dependence logic is a variant of Wilfrid HodgesWilfrid Hodges
Wilfrid Augustine Hodges is a British mathematician, known for his work in model theory. He was Professor of Mathematics at Queen Mary, University of London from 1987 to 2006, and is the author of numerous books on logic....
' compositional semantics for IF logic
Independence-friendly logic
Independence-friendly logic , proposed by Jaakko Hintikka and Gabriel Sandu, aims at being a more natural and intuitive alternative to classical first-order logic . IF logic is characterized by branching quantifiers...
. There exist equivalent game-theoretic semantics for dependence logic, both in terms of imperfect information games and in terms of perfect information games.
Teams
Let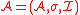 be a first-order structureStructure (mathematical logic)
In universal algebra and in model theory, a structure consists of a set along with a collection of finitary operations and relations which are defined on it....
and let
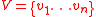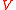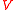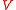be a finite set of variables. Then a team over with domain is a set of assignments over with domain , that is, a set of functions from to .It may be helpful to visualize such a team as a database relation
Relation (database)
In relational model:A relation value, which is assigned to a certain relation variable, is time-varying. By using a Data Definition Language , it is able to define relation variables.The following is an example of a heading which consists of three attributes....
with attributes
and with only one data type, corresponding to the domain of the structure: for example, if the team consists of four assignments with domain 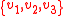then one may represent it as the relation
 |
 |
 |
|
|---|---|---|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
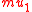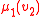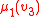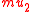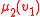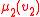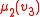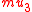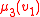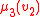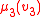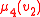Positive and negative satisfaction
Team semantics can be defined in terms of two relations and between structures, teams and formulas.Given a structure
, a team over it and a dependence logic formula whose free variablesFree variables and bound variables
In mathematics, and in other disciplines involving formal languages, including mathematical logic and computer science, a free variable is a notation that specifies places in an expression where substitution may take place...
are contained in the domain of
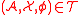, if we say that is a trump for in , and we write that ; and analogously, if we say that is a cotrump for in , and we write that .If
one can also say that is positively satisfied by in , and if instead one can say that is negatively satisfied by in .The necessity of considering positive and negative satisfaction separately is a consequence of the fact that in dependence logic, as in the logic of branching quantifiers
Branching quantifier
In logic a branching quantifier, also called a Henkin quantifier, finite partially ordered quantifier or even nonlinear quantifier, is a partial ordering\langle Qx_1\dots Qx_n\rangle...
or in IF logic
Independence-friendly logic
Independence-friendly logic , proposed by Jaakko Hintikka and Gabriel Sandu, aims at being a more natural and intuitive alternative to classical first-order logic . IF logic is characterized by branching quantifiers...
, the law of the excluded middle does not hold; alternatively, one may assume that all formulas are in negation normal form, using De Morgan's relations in order to define universal quantification and conjunction from existential quantification and disjunction respectively, and consider positive satisfaction alone.
Given a sentence
, we say that is true in if and only if , and we say that is false in if and only if .Semantic rules
As for the case of Alfred TarskiAlfred Tarski
Alfred Tarski was a Polish logician and mathematician. Educated at the University of Warsaw and a member of the Lwow-Warsaw School of Logic and the Warsaw School of Mathematics and philosophy, he emigrated to the USA in 1939, and taught and carried out research in mathematics at the University of...
's satisfiability relation for first-order formulas, the positive and negative satisfiability relations of the team semantics for dependence logic are defined by structural induction
Structural induction
Structural induction is a proof method that is used in mathematical logic , computer science, graph theory, and some other mathematical fields. It is a generalization of mathematical induction...
over the formulas of the language. Since the negation operator interchanges positive and negative satisfiability, the two inductions corresponding to
and need to be performed simultaneously:Positive satisfiability
-
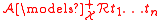if and only if
-
is a n-ary symbol in the signature of 
; - All variables occurring in the terms
are in the domain of 
; - For every assignment
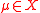, the evaluation of the tuple 
according to 
is in the interpretation of 
in 
;
-
-
if and only if
- All variables occurring in the terms
and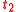 
are in the domain of 
; - For every assignment
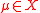, the evaluations of 
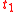and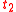 
according to 
are the same;
- All variables occurring in the terms
-
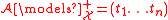if and only if any two assignments 
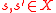whose evaluations of the tuple 
coincide assign the same value to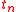 
; -
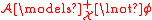if and only if 
; -
if and only if there exist teams 
and 
such that
-
' -
; -
;
-
-
if and only if there exists a function 
from 
to the domain of 
such that 
, where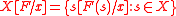 
.
Negative satisfiability
-
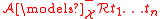if and only if
-
is a n-ary symbol in the signature of 
; - All variables occurring in the terms
are in the domain of 
; - For every assignment
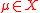, the evaluation of the tuple 
according to 
is not in the interpretation of 
in 
;
-
-
if and only if
- All variables occurring in the terms
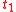and 
are in the domain of 
; - For every assignment
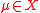, the evaluations of 
and 
according to 
are different;
- All variables occurring in the terms
-
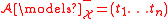if and only if 
is the empty team; -
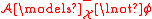if and only if 
; -
if and only if 
and 
; -
if and only if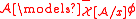 
, where 
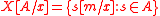and 
is the domain of 
.
Dependence logic and first-order logic
Dependence logic is a conservative extensionConservative extension
In mathematical logic, a logical theory T_2 is a conservative extension of a theory T_1 if the language of T_2 extends the language of T_1; every theorem of T_1 is a theorem of T_2; and any theorem of T_2 which is in the language of T_1 is already a theorem of T_1.More generally, if Γ is a set of...
of first-order logic: in other words, for every first order sentence
and structure we have that if and only if is true in according to the usual first order semantics. Furthemore, for any first order formula , if and only if all assignments 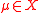satisfy in according to the usual first order semantics.However, dependence logic is strictly more expressive than first order logic: for example, the sentence
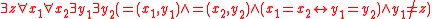
is true in a model
if and only if the domain of this model is infinite, even though no first order formula has this property.Dependence logic and second-order logic
Every dependence logic sentence is equivalent to some sentence in the existential fragment of second order logic, that is, to some second order sentence of the form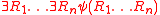where
does not contain second order quantifiers.Conversely, every second-order sentence in the above form is equivalent to some dependence logic sentence.
As for open formulas, dependence logic corresponds to the downwards monotone fragment of existential second order logic, in the sense that a nonempty class of teams is definable by a dependence logic formula if and only if the corresponding class of relations is downwards monotone and definable by an existential second order formula.
Dependence logic and branching quantifiers
Branching quantifiers are expressible in terms of dependence atoms: for example, the expression
is equivalent to the dependence logic sentence
, in the sense that the former expression is true in a model if and only if the latter expression is true.Conversely, any dependence logic sentence is equivalent to some sentence in the logic of branching quantifiers, since all existential second order sentences are expressible in branching quantifier logic.
Dependence logic and IF logic
Any dependence logic sentence is logically equivalent to some IF logic sentence, and vice versa.However, the issue is subtler when it comes to open formulas. Translations between IF logic and dependence logic formulas, and vice versa, exist as long as the domain of the team is fixed: in other words, for all sets of variables
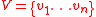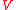and all IF logic formulas with free variables in there exists a dependence logic formula 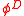such thatfor all structures
and for all teams with domain 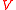, and conversely, for every dependence logic formula 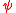with free variables in 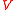there exists an IF logic formula 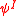such that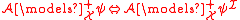for all structures
and for all teams with domain 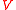. These translations cannot be compositional.Properties
Dependence logic formulas are downwards closed: if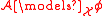 and then 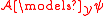. Furthermore, the empty team (but not the team containing the empty assignment) satisfies all formulas of Dependence Logic, both positively and negatively.The law of the excluded middle fails in dependence logic: for example, the formula
is neither positively nor negatively satisfied by the team 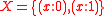. Furthermore, disjunction is not idempotent and does not distribute over conjunction.Both the compactness theorem
Compactness theorem
In mathematical logic, the compactness theorem states that a set of first-order sentences has a model if and only if every finite subset of it has a model...
and the Löwenheim-Skolem theorem are true for dependence logic. Craig's interpolation theorem
Craig interpolation
In mathematical logic, Craig's interpolation theorem is a result about the relationship between different logical theories. Roughly stated, the theorem says that if a formula φ implies a formula ψ then there is a third formula ρ, called an interpolant, such that every nonlogical symbol...
also holds, but, due to the nature of negation in dependence logic, in a slightly modified formulation: if two dependence logic formulas
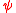and are contradictory, that is, it is never the case that both and 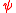hold in the same model, then there exists a first order sentence in the common language of the two sentences such that implies and is contradictory with 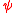.As IF logic, Dependence logic can define its own truth operator: more precisely, there exists a formula
such that for every sentence of dependence logic and all models 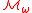which satisfy Peano's axioms, if 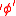is the Gödel number of then
-
if and only if 
This does not contradict Tarski's undefinability theorem, since the negation of dependence logic is not the usual contradictory one.
Complexity
As a consequence of Fagin's theoremFagin's theorem
Fagin's theorem is a result in descriptive complexity theory that states that the set of all properties expressible in existential second-order logic is precisely the complexity class NP...
, the model checking problem for dependence logic is NP-complete
NP-complete
In computational complexity theory, the complexity class NP-complete is a class of decision problems. A decision problem L is NP-complete if it is in the set of NP problems so that any given solution to the decision problem can be verified in polynomial time, and also in the set of NP-hard...
. Furthermore, its inconsistency problem is semidecidable, and in fact equivalent to the inconsistency problem for first-order logic.
However, the decision problem for dependence logic is non-arithmetical
Arithmetical hierarchy
In mathematical logic, the arithmetical hierarchy, arithmetic hierarchy or Kleene-Mostowski hierarchy classifies certain sets based on the complexity of formulas that define them...
, and is in fact complete with respect to the
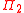class of the Levy hierarchy.Team logic
Team logic extends dependence logic with a contradictory negation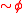. Its expressive power is equivalent to that of full second order logic.Modal dependence logic
The dependence atom, or a suitable variant thereof, can be added to the language of modal logicModal logic
Modal logic is a type of formal logic that extends classical propositional and predicate logic to include operators expressing modality. Modals — words that express modalities — qualify a statement. For example, the statement "John is happy" might be qualified by saying that John is...
, thus obtaining modal dependence logic.
Intuitionistic dependence logic
As it is, dependence logic lacks an implication. The intuitionistic implication, whose name derives from the similarity between its definition and that of the implication of intuitionistic logicIntuitionistic logic
Intuitionistic logic, or constructive logic, is a symbolic logic system differing from classical logic in its definition of the meaning of a statement being true. In classical logic, all well-formed statements are assumed to be either true or false, even if we do not have a proof of either...
, can be defined as follows:
-
if and only if for all 
such that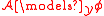 
it holds that 
.
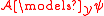Intuitionistic dependence logic, that is, dependence logic supplemented with the intuitionistic implication, is equivalent to second-order logic.