Debye–Hückel theory
Encyclopedia
The Debye–Hückel theory was proposed by Peter Debye
and Erich Hückel
as a theoretical explanation for departures from ideality in solutions of electrolyte
s. It was based on an extremely simplified model of the electrolyte solution but nevertheless gave accurate predictions of mean activity coefficient
s for ions in dilute solution. The Debye-Hückel equation provides a starting point for modern treatments of non-ideality of electrolyte solutions.
of electrolyte
solutions, an ideal solution
is a solution whose colligative properties
are proportional to the concentration
of the solute
. Real solutions show departures from this kind of ideality at all but the very lowest concentrations (see, for example, Raoult's law). In order to accommodate these effects in the thermodynamics
of solutions, the concept of activity
was introduced: the properties are then proportional to the activities of the ions. Activity, a, is proportional to concentration, c. The proportionality constant is known as an activity coefficient
, γ.
In an ideal electrolyte solution the activity coefficients of all the ions are equal to one. Non-ideality arises principally (but not exclusively) because ions of opposite charge attract each other due to electrostatic forces, while ions of the same charge repel each other. In consequence ions are not randomly distributed throughout the solution, as they would be in an ideal solution.
Activity coefficients of single ions cannot be measured experimentally because an electrolyte solution must contain both positively charged ions and negatively charged ions. Instead, a mean activity coefficient,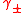
is defined. For example, with the electrolyte NaCl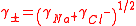
In general, the mean activity coefficient of a fully dissociated electrolyte of formula AnBm is given by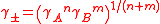
Activity coefficients are themselves functions of concentration as the amount of inter-ionic interaction increases as the concentration of the electrolyte increases. Debye and Hückel developed a theory with which single ion activity coefficients could be calculated. By calculating the mean activity coefficients from them the theory could be tested against experimental data. It was found to give excellent agreement for "dilute" solutions.
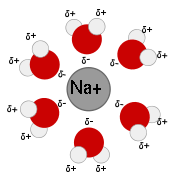
A snapshot of a 2-dimensional section of an idealized electrolyte solution is shown in the adjacent picture. The ions are shown as spheres with unit electrical charge. The solvent (pale blue) is shown as a uniform medium, without structure. On average, each ion is surrounded more closely by ions of opposite charge than by ions of like charge. These concepts were developed into a quantitative theory involving ions of charge z1e+ and z2e-, where z can be any integer. The principal assumption is that departure from non-ideality is due to electrostatic interactions between ions, mediated by Coulomb's law
: the force of interaction between two ions, separated by a distance, r in a medium of relative permittivity εr is given by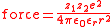
It is also assumed that
The last assumption means that each cation is surrounded by a spherically symmetric cloud of other ions. The cloud has a net negative charge. Similarly each anion is surrounded by a cloud with net positive charge.
The first step is to specify the electrostatic potential for ion j by means of Poisson's equation
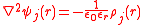
ψ(r) is the total potential at a distance, r, from the central ion and ρ(r) is the averaged charge density of the surrounding cloud at that distance. To apply this formula it is essential that the cloud has spherical symmetry, that is, the charge density is a function only of distance from the central ion as this allows the Poisson equation to be cast in terms of spherical coordinates with no angular dependence.
The second step is to calculate the charge density by means of a Maxwell-Boltzmann distribution.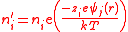
This distribution also depends on the potential ψ(r) and this introduces a serious difficulty in terms of the superposition principle
. Nevertheless, the two equations can be combined to produce the Poisson-Boltzmann equation
.
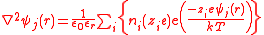
Solution of this equation is far from straightforward. It can be solved by successive approximations using modern electronic computers, but these were not available to Debye and Hückel. Instead they expanded the exponential as a truncated Taylor series
. This results in the truncated Poisson-Boltzmann equation,
which is a type of differential equation
which has an analytical solution. This equation applies to 1:1, 2:2 and 3:3 electrolytes; for unsymmetrical electrolytes another term in ψ2 must be included. Incidentally, the truncated expansion may also remove the difficulty with the superposition principle.
The solution for a symmetrical electrolyte, in terms of the mean activity coefficient is given as
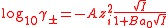
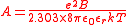
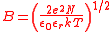
I is the ionic strength
and a0 is a parameter which represent the distance of closest approach of ions. For aqueous solutions at 25 °C A = 0.051 mol-1/2dm3/2 and B = 3.29 mol-1/2dm3/2
The most significant aspect of these algebraic manipulations is the prediction that the mean activity coefficient is a function of ionic strength. Of course the ionic strength of a 1:1 electrolyte is equal to its concentration, but that is not true for other types of electrolyte. Generally, activity coefficients are discussed in terms of ionic strength rather than the electrolyte concentration. For very low values of the ionic strength the value of the denominator in the expression above becomes nearly equal to one. In this situation the mean activity coefficient is proportional to the square root of the ionic strength. This is known as the Debye-Hückel limiting law.
The equation for log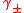 
gives satisfactory agreement with experimental measurements for low electrolyte concentrations, typically less than 10−3 mol dm−3. Deviations from the theory occur at higher concentrations and with electrolytes that produce ions of higher charges, particularly unsymmetrical electrolytes. Essentially these deviations occur because the model is hopelessly over-simplified, so there is little to be gained making small adjustments to the model. The individual assumptions can be challenged in turn.
Moreover, we assume that the ionic radius is negligible, but at higher concentrations, the ionic radius becomes comparable to the radius of the ionic cloud!
Most extensions to Debye–Hückel theory are empirical in nature. They usually allow the Debye–Hückel equation to be followed at low concentration and add further terms in some power of the ionic strength to fit experimental observations. The main extensions are the Davies equation
, Pitzer equations and Specific ion interaction theory
.
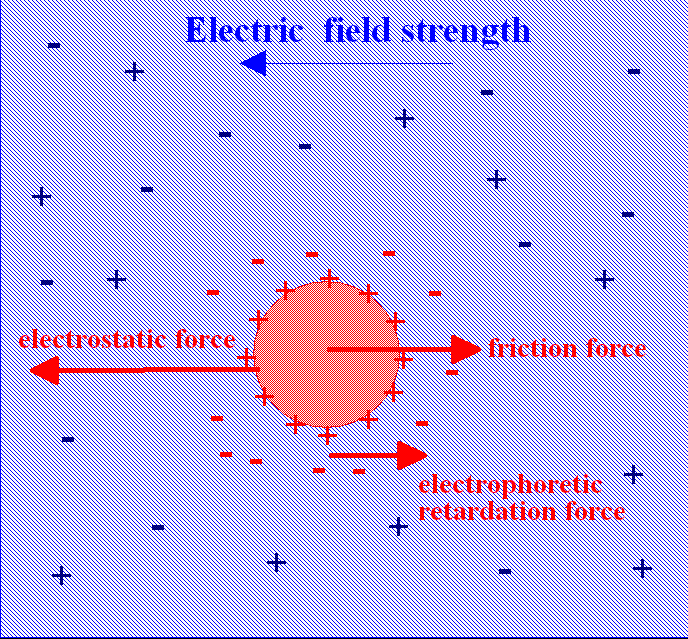The treatment given so far is for a system not subject to an external electric field. When conductivity
is measured the system is subject to an oscillating external field due to the application of an AC
voltage to electrodes immersed in the solution. Debye and Hückel modified their theory in 1926 and their theory was further modified by Lars Onsager
in 1927. All the postulates of the original theory were retained. In addition it was assumed that the electric field causes the charge cloud to be distorted away from spherical symmetry. After taking this into account, together with the specific requirements of moving ions, such as viscosity
and electrophoretic effects, Onsager was able to derive a theoretical expression to account for the empirical relation known as Kohlrausch's Law, for the molar conductivity, Λm.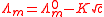
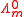is known as the limiting molar conductivity, K is an empirical constant and c is the electrolyte concentration Limiting here means "at the limit of the infinite dilution").
Onsager's expression is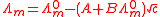
where A and B are constants that depend only on known quantities such as temperature, the charges on the ions and the dielectric constant and viscosity of the solvent. This is known as the Debye-Hückel-Onsager equation. However, this equation only applies to very dilute solutions and has been largely superseded by other equations due to Fuoss and Onsager, 1932 and 1957 and later.
Peter Debye
Peter Joseph William Debye FRS was a Dutch physicist and physical chemist, and Nobel laureate in Chemistry.-Early life:...
and Erich Hückel
Erich Hückel
Erich Armand Arthur Joseph Hückel was a German physicist and physical chemist. He is known for two major contributions:*The Debye–Hückel theory of electrolytic solutions...
as a theoretical explanation for departures from ideality in solutions of electrolyte
Electrolyte
In chemistry, an electrolyte is any substance containing free ions that make the substance electrically conductive. The most typical electrolyte is an ionic solution, but molten electrolytes and solid electrolytes are also possible....
s. It was based on an extremely simplified model of the electrolyte solution but nevertheless gave accurate predictions of mean activity coefficient
Activity coefficient
An activity coefficient is a factor used in thermodynamics to account for deviations from ideal behaviour in a mixture of chemical substances. In an ideal mixture, the interactions between each pair of chemical species are the same and, as a result, properties of the mixtures can be expressed...
s for ions in dilute solution. The Debye-Hückel equation provides a starting point for modern treatments of non-ideality of electrolyte solutions.
Overview
In the chemistryChemistry
Chemistry is the science of matter, especially its chemical reactions, but also its composition, structure and properties. Chemistry is concerned with atoms and their interactions with other atoms, and particularly with the properties of chemical bonds....
of electrolyte
Electrolyte
In chemistry, an electrolyte is any substance containing free ions that make the substance electrically conductive. The most typical electrolyte is an ionic solution, but molten electrolytes and solid electrolytes are also possible....
solutions, an ideal solution
Ideal solution
In chemistry, an ideal solution or ideal mixture is a solution with thermodynamic properties analogous to those of a mixture of ideal gases. The enthalpy of solution is zero as is the volume change on mixing; the closer to zero the enthalpy of solution is, the more "ideal" the behavior of the...
is a solution whose colligative properties
Colligative properties
Colligative properties are properties of solutions that depend on the number of molecules in a given volume of solvent and not on the properties/identity of the molecules. Colligative properties include: relative lowering of vapor pressure; elevation of boiling point; depression of freezing point...
are proportional to the concentration
Concentration
In chemistry, concentration is defined as the abundance of a constituent divided by the total volume of a mixture. Four types can be distinguished: mass concentration, molar concentration, number concentration, and volume concentration...
of the solute
Solute
Solute may refer to:* Solute, UMIK or UBOOK desolving in a substance,forming INT/INTY* Solute , a group of Paleozoic echinoderms...
. Real solutions show departures from this kind of ideality at all but the very lowest concentrations (see, for example, Raoult's law). In order to accommodate these effects in the thermodynamics
Thermodynamics
Thermodynamics is a physical science that studies the effects on material bodies, and on radiation in regions of space, of transfer of heat and of work done on or by the bodies or radiation...
of solutions, the concept of activity
Activity (chemistry)
In chemical thermodynamics, activity is a measure of the “effective concentration” of a species in a mixture, meaning that the species' chemical potential depends on the activity of a real solution in the same way that it would depend on concentration for an ideal solution.By convention, activity...
was introduced: the properties are then proportional to the activities of the ions. Activity, a, is proportional to concentration, c. The proportionality constant is known as an activity coefficient
Activity coefficient
An activity coefficient is a factor used in thermodynamics to account for deviations from ideal behaviour in a mixture of chemical substances. In an ideal mixture, the interactions between each pair of chemical species are the same and, as a result, properties of the mixtures can be expressed...
, γ.
- a = γ c
In an ideal electrolyte solution the activity coefficients of all the ions are equal to one. Non-ideality arises principally (but not exclusively) because ions of opposite charge attract each other due to electrostatic forces, while ions of the same charge repel each other. In consequence ions are not randomly distributed throughout the solution, as they would be in an ideal solution.
Activity coefficients of single ions cannot be measured experimentally because an electrolyte solution must contain both positively charged ions and negatively charged ions. Instead, a mean activity coefficient,
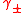is defined. For example, with the electrolyte NaCl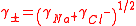In general, the mean activity coefficient of a fully dissociated electrolyte of formula AnBm is given by
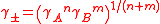
Activity coefficients are themselves functions of concentration as the amount of inter-ionic interaction increases as the concentration of the electrolyte increases. Debye and Hückel developed a theory with which single ion activity coefficients could be calculated. By calculating the mean activity coefficients from them the theory could be tested against experimental data. It was found to give excellent agreement for "dilute" solutions.
The model
A description of Debye–Hückel theory includes a very detailed discussion of the assumptions and their limitations as well as the mathematical development and applications.A snapshot of a 2-dimensional section of an idealized electrolyte solution is shown in the adjacent picture. The ions are shown as spheres with unit electrical charge. The solvent (pale blue) is shown as a uniform medium, without structure. On average, each ion is surrounded more closely by ions of opposite charge than by ions of like charge. These concepts were developed into a quantitative theory involving ions of charge z1e+ and z2e-, where z can be any integer. The principal assumption is that departure from non-ideality is due to electrostatic interactions between ions, mediated by Coulomb's law
Coulomb's law
Coulomb's law or Coulomb's inverse-square law, is a law of physics describing the electrostatic interaction between electrically charged particles. It was first published in 1785 by French physicist Charles Augustin de Coulomb and was essential to the development of the theory of electromagnetism...
: the force of interaction between two ions, separated by a distance, r in a medium of relative permittivity εr is given by
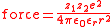
It is also assumed that
- The dissolved electrolyte is completely dissociated; it is a strong electrolyteStrong electrolyteA strong electrolyte is a solute that completely, or almost completely, ionizes or dissociates in a solution. These ions are good conductors of electric current in the solution....
. - Ions are spherical and are not polarizedPolarizabilityPolarizability is the measure of the change in a molecule's electron distribution in response to an applied electric field, which can also be induced by electric interactions with solvents or ionic reagents. It is a property of matter...
by the surrounding electric fieldElectric fieldIn physics, an electric field surrounds electrically charged particles and time-varying magnetic fields. The electric field depicts the force exerted on other electrically charged objects by the electrically charged particle the field is surrounding...
. Solvation of ions is ignored except insofar as it determines the effective sizes of the ions. - The solvent plays no role other than providing a medium of constant relative permittivity (dielectric constantDielectric constantThe relative permittivity of a material under given conditions reflects the extent to which it concentrates electrostatic lines of flux. In technical terms, it is the ratio of the amount of electrical energy stored in a material by an applied voltage, relative to that stored in a vacuum...
). - There is no ElectrostrictionElectrostrictionElectrostriction is a property of all electrical non-conductors, or dielectrics, that causes them to change their shape under the application of an electric field. - Explanation :...
. - Individual ions surrounding a "central" ion can be represented by a statistically averaged cloud of continuous charge density, with a minimum distance of closest approach.
The last assumption means that each cation is surrounded by a spherically symmetric cloud of other ions. The cloud has a net negative charge. Similarly each anion is surrounded by a cloud with net positive charge.
Mathematical development
The deviation from ideality is taken to be a function of the potential energy resulting from the electrostatic interactions between ions and their surrounding clouds. To calculate this energy two steps are needed.The first step is to specify the electrostatic potential for ion j by means of Poisson's equation
Poisson's equation
In mathematics, Poisson's equation is a partial differential equation of elliptic type with broad utility in electrostatics, mechanical engineering and theoretical physics...
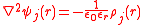
ψ(r) is the total potential at a distance, r, from the central ion and ρ(r) is the averaged charge density of the surrounding cloud at that distance. To apply this formula it is essential that the cloud has spherical symmetry, that is, the charge density is a function only of distance from the central ion as this allows the Poisson equation to be cast in terms of spherical coordinates with no angular dependence.
The second step is to calculate the charge density by means of a Maxwell-Boltzmann distribution.
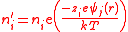
This distribution also depends on the potential ψ(r) and this introduces a serious difficulty in terms of the superposition principle
Superposition principle
In physics and systems theory, the superposition principle , also known as superposition property, states that, for all linear systems, the net response at a given place and time caused by two or more stimuli is the sum of the responses which would have been caused by each stimulus individually...
. Nevertheless, the two equations can be combined to produce the Poisson-Boltzmann equation
Poisson-Boltzmann equation
The Poisson–Boltzmann equation is a differential equation that describes electrostatic interactions between molecules in ionic solutions. It is the mathematical base for the Gouy–Chapman double layer theory; first proposed by Gouy in 1910 and complemented by Chapman in 1913...
.
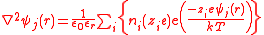
Solution of this equation is far from straightforward. It can be solved by successive approximations using modern electronic computers, but these were not available to Debye and Hückel. Instead they expanded the exponential as a truncated Taylor series
Taylor series
In mathematics, a Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point....
. This results in the truncated Poisson-Boltzmann equation,
which is a type of differential equation
Differential equation
A differential equation is a mathematical equation for an unknown function of one or several variables that relates the values of the function itself and its derivatives of various orders...
which has an analytical solution. This equation applies to 1:1, 2:2 and 3:3 electrolytes; for unsymmetrical electrolytes another term in ψ2 must be included. Incidentally, the truncated expansion may also remove the difficulty with the superposition principle.
The solution for a symmetrical electrolyte, in terms of the mean activity coefficient is given as
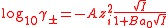
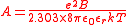
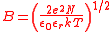
I is the ionic strength
Ionic strength
The ionic strength of a solution is a measure of the concentration of ions in that solution. Ionic compounds, when dissolved in water, dissociate into ions. The total electrolyte concentration in solution will affect important properties such as the dissociation or the solubility of different salts...
and a0 is a parameter which represent the distance of closest approach of ions. For aqueous solutions at 25 °C A = 0.051 mol-1/2dm3/2 and B = 3.29 mol-1/2dm3/2
The most significant aspect of these algebraic manipulations is the prediction that the mean activity coefficient is a function of ionic strength. Of course the ionic strength of a 1:1 electrolyte is equal to its concentration, but that is not true for other types of electrolyte. Generally, activity coefficients are discussed in terms of ionic strength rather than the electrolyte concentration. For very low values of the ionic strength the value of the denominator in the expression above becomes nearly equal to one. In this situation the mean activity coefficient is proportional to the square root of the ionic strength. This is known as the Debye-Hückel limiting law.
Limitations and extensions
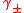gives satisfactory agreement with experimental measurements for low electrolyte concentrations, typically less than 10−3 mol dm−3. Deviations from the theory occur at higher concentrations and with electrolytes that produce ions of higher charges, particularly unsymmetrical electrolytes. Essentially these deviations occur because the model is hopelessly over-simplified, so there is little to be gained making small adjustments to the model. The individual assumptions can be challenged in turn.
- Complete dissociation. Ion association may take place, particularly with ions of higher charge. This was followed up in detail by Niels BjerrumNiels BjerrumNiels Janniksen Bjerrum, born March 11, 1879 in Copenhagen, died September 30, 1958, was a Danish chemist.Niels Bjerrum was the son of opthamologist Jannik Petersen Bjerrum, and started to study at University of Copenhagen in 1897. He received his Master's degree in 1902 and his Doctor's degree in...
. The Bjerrum lengthBjerrum lengthThe Bjerrum length is the separation at which the electrostatic interaction between twoelementary charges is comparable in magnitude to the thermal energy scale,...
is the separation at which the electrostatic interaction between two ions is comparable in magnitude to kT. - Weak electrolytes. A weak electrolyte is one that is not fully dissociated. As such it has a dissociation constant. The dissociation constant can be used to calculate the extent of dissociation and hence, make the necessary correction needed to calculate activity coefficients.
- Ions are spherical and are not polarizedPolarizabilityPolarizability is the measure of the change in a molecule's electron distribution in response to an applied electric field, which can also be induced by electric interactions with solvents or ionic reagents. It is a property of matter...
. Many ions such as the nitrateNitrateThe nitrate ion is a polyatomic ion with the molecular formula NO and a molecular mass of 62.0049 g/mol. It is the conjugate base of nitric acid, consisting of one central nitrogen atom surrounded by three identically-bonded oxygen atoms in a trigonal planar arrangement. The nitrate ion carries a...
ion, NO3-, are manifestly not spherical. Polyatomic ions are also polarizable. - Role of the solvent. The solvent is not a structureless medium but is made up of molecules. The water molecules in aqueous solution are both dipolar and polarizable. Both cations and anions have a strong primary solvation shellSolvation shellA Solvation shell is a shell of any chemical species acting as a solvent, surrounding a solute species. When the solvent is water it is often referred to as a hydration shell or hydration sphere....
and a weaker secondary solvation shell. Ion-solvent interactions are ignored in Debye–Hückel theory.
Moreover, we assume that the ionic radius is negligible, but at higher concentrations, the ionic radius becomes comparable to the radius of the ionic cloud!
Most extensions to Debye–Hückel theory are empirical in nature. They usually allow the Debye–Hückel equation to be followed at low concentration and add further terms in some power of the ionic strength to fit experimental observations. The main extensions are the Davies equation
Davies equation
The Davies equation is an empirical extension of Debye–Hückel theory which can be used to calculate activity coefficients of electrolyte solutions at relatively high concentrations. The equation, originally published in 1938, was refined by fitting to experimental data...
, Pitzer equations and Specific ion interaction theory
Specific ion interaction theory
Specific ion Interaction Theory is a theory used to estimate single-ion activity coefficients in electrolyte solutions at relatively high concentrations. It does so by taking into consideration interaction coefficients between the various ions present in solution...
.
Conductivity
Conductivity (electrolytic)
The conductivity of an electrolyte solution is a measure of its ability to conduct electricity. The SI unit of conductivity is siemens per meter ....
is measured the system is subject to an oscillating external field due to the application of an AC
Alternating current
In alternating current the movement of electric charge periodically reverses direction. In direct current , the flow of electric charge is only in one direction....
voltage to electrodes immersed in the solution. Debye and Hückel modified their theory in 1926 and their theory was further modified by Lars Onsager
Lars Onsager
Lars Onsager was a Norwegian-born American physical chemist and theoretical physicist, winner of the 1968 Nobel Prize in Chemistry.He held the Gibbs Professorship of Theoretical Chemistry at Yale University....
in 1927. All the postulates of the original theory were retained. In addition it was assumed that the electric field causes the charge cloud to be distorted away from spherical symmetry. After taking this into account, together with the specific requirements of moving ions, such as viscosity
Viscosity
Viscosity is a measure of the resistance of a fluid which is being deformed by either shear or tensile stress. In everyday terms , viscosity is "thickness" or "internal friction". Thus, water is "thin", having a lower viscosity, while honey is "thick", having a higher viscosity...
and electrophoretic effects, Onsager was able to derive a theoretical expression to account for the empirical relation known as Kohlrausch's Law, for the molar conductivity, Λm.
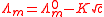
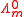is known as the limiting molar conductivity, K is an empirical constant and c is the electrolyte concentration Limiting here means "at the limit of the infinite dilution").Onsager's expression is
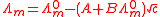
where A and B are constants that depend only on known quantities such as temperature, the charges on the ions and the dielectric constant and viscosity of the solvent. This is known as the Debye-Hückel-Onsager equation. However, this equation only applies to very dilute solutions and has been largely superseded by other equations due to Fuoss and Onsager, 1932 and 1957 and later.