
Containment order
Encyclopedia
In the mathematical
field of order theory
, a containment order is the partial order that arises as the subset
-containment relation on some collection of objects. In a simple way, every poset
P = (≤, X) is (isomorphic
to) a containment order (like every group is isomorphic to a permutation group - Cayley's theorem
). To see this, associate to each element x of X the set
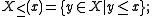
then the transitivity of ≤ ensures that for all a and b in X, we have

More interestingly, several important classes of poset arise as containment orders for some natural collections, like the Boolean lattice Qn, which is the collection of all 2n subsets of an n-element set, the dimension
-n orders, which are the containment orders on collections of n-boxes anchored at the origin, and the interval-containment orders, which are precisely the orders of dimension
2. Other containment orders that are interesting in their own right include the circle orders, which arise from disks in the plane, and the angle orders.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
field of order theory
Order theory
Order theory is a branch of mathematics which investigates our intuitive notion of order using binary relations. It provides a formal framework for describing statements such as "this is less than that" or "this precedes that". This article introduces the field and gives some basic definitions...
, a containment order is the partial order that arises as the subset
Subset
In mathematics, especially in set theory, a set A is a subset of a set B if A is "contained" inside B. A and B may coincide. The relationship of one set being a subset of another is called inclusion or sometimes containment...
-containment relation on some collection of objects. In a simple way, every poset
Partially ordered set
In mathematics, especially order theory, a partially ordered set formalizes and generalizes the intuitive concept of an ordering, sequencing, or arrangement of the elements of a set. A poset consists of a set together with a binary relation that indicates that, for certain pairs of elements in the...
P = (≤, X) is (isomorphic
Isomorphism
In abstract algebra, an isomorphism is a mapping between objects that shows a relationship between two properties or operations. If there exists an isomorphism between two structures, the two structures are said to be isomorphic. In a certain sense, isomorphic structures are...
to) a containment order (like every group is isomorphic to a permutation group - Cayley's theorem
Cayley's theorem
In group theory, Cayley's theorem, named in honor of Arthur Cayley, states that every group G is isomorphic to a subgroup of the symmetric group acting on G...
). To see this, associate to each element x of X the set
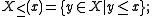
then the transitivity of ≤ ensures that for all a and b in X, we have

More interestingly, several important classes of poset arise as containment orders for some natural collections, like the Boolean lattice Qn, which is the collection of all 2n subsets of an n-element set, the dimension
Order dimension
In mathematics, the dimension of a partially ordered set is the smallest number of total orders the intersection of which gives rise to the partial order....
-n orders, which are the containment orders on collections of n-boxes anchored at the origin, and the interval-containment orders, which are precisely the orders of dimension
Order dimension
In mathematics, the dimension of a partially ordered set is the smallest number of total orders the intersection of which gives rise to the partial order....
2. Other containment orders that are interesting in their own right include the circle orders, which arise from disks in the plane, and the angle orders.

