In mathematics

Mathematics

Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...

, the dimension of a partially ordered set

Partially ordered set

In mathematics, especially order theory, a partially ordered set formalizes and generalizes the intuitive concept of an ordering, sequencing, or arrangement of the elements of a set. A poset consists of a set together with a binary relation that indicates that, for certain pairs of elements in the...

 (poset) is the smallest number of total order

Total order

In set theory, a total order, linear order, simple order, or ordering is a binary relation on some set X. The relation is transitive, antisymmetric, and total...

s the intersection of which gives rise to the partial order.
This concept is also sometimes called the order dimension or the Dushnik–Miller dimension of the partial order.
first studied order dimension; for a more detailed treatment of this subject than provided here, see .

Formal definition

The dimension of a poset P is the least integer t for which there exists a family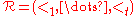


of linear extension

Linear extension

In order theory, a branch of mathematics, a linear extension of a partial order is a linear order that is compatible with the partial order.-Definitions:...

s of P so that, for every x and y in P, x precedes y in P if and only if it precedes y in each of the linear extensions. That is,

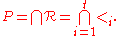
Realizers

A family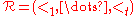 of linear orders on X is called a realizer of a poset P = (X, <_P) if,

which is to say that for any x and y in X,
x <_P y precisely when x <₁ y, x <₂ y, ..., and x <_t y.
Thus, an equivalent definition of the dimension of a poset P is "the least cardinality of a realizer of P."

It can be shown that any nonempty family R of linear extensions is a realizer if and only if, for every critical pair

Critical pair (order theory)

In order theory, a discipline within mathematics, a critical pair is a pair of elements in a partially ordered set that are incomparable but that could be made comparable without changing the order relationships of any other pairs of elements....

 (x,y) of P, y <_i x for some order
<_i in R.

Example

Let n be a positive integer, and let P be the partial order on the elements a_i and b_i (for 1 ≤ i ≤ n) in which a_i ≤ b_j whenever i ≠ j, but no other pairs are comparable. In particular, a_i and b_i are incomparable in P; P can be viewed as an oriented form of a crown graph

Crown graph

In graph theory, a branch of mathematics, a crown graph on 2n vertices is an undirected graph with two sets of vertices ui and vi and with an edge from ui to vj whenever i ≠ j...

. The illustration shows an ordering of this type for n = 4.

Then, for each i, any realizer must contain a linear order that begins with all the a_j except a_i (in some order), then includes b_i, then a_i, and ends with all the remaining b_j. This is so because if there were a realizer that didn't include such an order, then the intersection of that realizer's orders would have a_i preceding b_i, which would contradict the incomparability of a_i and b_i in P. And conversely, any family of linear orders that includes one order of this type for each i has P as its intersection. Thus, P has dimension exactly n. In fact, P is known as the standard example of a poset of dimension n, and is usually denoted by S_n.

Order dimension two

The partial orders with order dimension two may be characterized as the partial orders whose comparability graph

Comparability graph

In graph theory, a comparability graph is an undirected graph that connects pairs of elements that are comparable to each other in a partial order...

 is the complement of the comparability graph of a different partial order . That is, P is a partial order with order dimension two if and only if there exists a partial order Q on the same set of elements, such that every pair x, y of distinct elements is comparable in exactly one of these two partial orders. If P is realized by two linear extensions, then partial order Q complementary to P may be realized by reversing one of the two linear extensions. Therefore, the comparability graphs of the partial orders of dimension two are exactly the permutation graph

Permutation graph

In areas of mathematics influenced by graph theory, a permutation graph is the intersection graph of a family of line segments that connect two parallel lines in the Euclidean plane...

s, graphs that are both themselves comparability graphs and complementary to comparability graphs.

The partial orders of order dimension two include the series-parallel partial order

Series-parallel partial order

In order-theoretic mathematics, a series-parallel partial order is a partially ordered set built up from smaller series-parallel partial orders by two simple composition operations....

s .

Computational complexity

It is possible to determine in polynomial time whether a given finite partially ordered set has order dimension at most two, for instance, by testing whether the comparability graph of the partial order is a permutation graph. However, for any k ≥ 3, it is NP-complete

NP-complete

In computational complexity theory, the complexity class NP-complete is a class of decision problems. A decision problem L is NP-complete if it is in the set of NP problems so that any given solution to the decision problem can be verified in polynomial time, and also in the set of NP-hard...

 to test whether the order dimension is at most k .

Incidence posets of graphs

The incidence poset of any undirected graph G has the vertices and edges of G as its elements; in this poset, x ≤ y if either x = y or x is a vertex, y is an edge, and x is an endpoint of y. Certain kinds of graphs may be characterized by the order dimensions of their incidence posets: a graph is a path graph

Path graph

In the mathematical field of graph theory, a path graph or linear graph is a particularly simple example of a tree, namely a tree with two or more vertices that is not branched at all, that is, contains only vertices of degree 2 and 1...

 if and only if the order dimension of its incidence poset is at most two, and according to Schnyder's theorem

Schnyder's theorem

In graph theory, Schnyder's theorem is a characterization of planar graphs in termsof the order dimension of their incidence posets. It is named after Walter Schnyder, who published its proof in 1989....

 it is a planar graph

Planar graph

In graph theory, a planar graph is a graph that can be embedded in the plane, i.e., it can be drawn on the plane in such a way that its edges intersect only at their endpoints...

if and only if the order dimension of its incidence poset is at most three .