Complex mexican hat wavelet
Encyclopedia
The complex Mexican hat wavelet is a low-oscillation, complex-valued, wavelet for the continuous wavelet transform
. This wavelet is formulated in terms of its Fourier transform
as the Hilbert analytic function of the conventional Mexican hat wavelet:
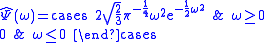
Temporally, this wavelet can be expressed in terms of the error function
,
as:
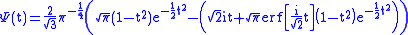
This wavelet has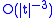
asymptotic temporal decay in 
,
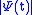dominated by the discontinuity
of the second derivative
of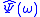
at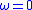
.
This wavelet was proposed in 2002 by Addison et al.
for applications requiring high temporal precision time-frequency analysis
.
Continuous wavelet transform
A continuous wavelet transform is used to divide a continuous-time function into wavelets. Unlike Fourier transform, the continuous wavelet transform possesses the ability to construct a time-frequency representation of a signal that offers very good time and frequency localization...
. This wavelet is formulated in terms of its Fourier transform
Fourier transform
In mathematics, Fourier analysis is a subject area which grew from the study of Fourier series. The subject began with the study of the way general functions may be represented by sums of simpler trigonometric functions...
as the Hilbert analytic function of the conventional Mexican hat wavelet:
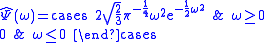
Temporally, this wavelet can be expressed in terms of the error function
Error function
In mathematics, the error function is a special function of sigmoid shape which occurs in probability, statistics and partial differential equations...
,
as:
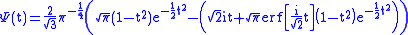
This wavelet has
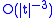asymptotic temporal decay in 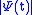,dominated by the discontinuity
Discontinuity
Discontinuity may refer to:*Discontinuity , a harmless irregularity in a casting*Discontinuity in geotechnics is a plane or surface marking a change in physical or chemical properties in a soil or rock mass...
of the second derivative
Derivative
In calculus, a branch of mathematics, the derivative is a measure of how a function changes as its input changes. Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a...
of
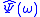
at
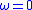.This wavelet was proposed in 2002 by Addison et al.
for applications requiring high temporal precision time-frequency analysis
Time-frequency analysis
In signal processing, time–frequency analysis comprises those techniques that study a signal in both the time and frequency domains simultaneously, using various time–frequency representations...
.