
Bipolar cylindrical coordinates
Encyclopedia

Orthogonal coordinates
In mathematics, orthogonal coordinates are defined as a set of d coordinates q = in which the coordinate surfaces all meet at right angles . A coordinate surface for a particular coordinate qk is the curve, surface, or hypersurface on which qk is a constant...
coordinate system
Coordinate system
In geometry, a coordinate system is a system which uses one or more numbers, or coordinates, to uniquely determine the position of a point or other geometric element. The order of the coordinates is significant and they are sometimes identified by their position in an ordered tuple and sometimes by...
that results from projecting the two-dimensional bipolar coordinate system
Bipolar coordinates
Bipolar coordinates are a two-dimensional orthogonal coordinate system. There are two commonly defined types of bipolar coordinates. The other system is two-center bipolar coordinates. There is also a third coordinate system that is based on two poles . The first is based on the Apollonian circles...
in the
perpendicular
 -direction. The two lines of foci
-direction. The two lines of fociFocus (geometry)
In geometry, the foci are a pair of special points with reference to which any of a variety of curves is constructed. For example, foci can be used in defining conic sections, the four types of which are the circle, ellipse, parabola, and hyperbola...
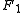 and
and 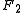 of the projected Apollonian circles
of the projected Apollonian circlesApollonian circles
Apollonian circles are two families of circles such that every circle in the first family intersects every circle in the second family orthogonally, and vice versa. These circles form the basis for bipolar coordinates...
are generally taken to be
defined by
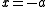 and
and 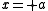 , respectively, (and by
, respectively, (and by  ) in the Cartesian coordinate system
) in the Cartesian coordinate systemCartesian coordinate system
A Cartesian coordinate system specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, measured in the same unit of length...
.
The term "bipolar" is often used to describe other curves having two singular points (foci), such as ellipse
Ellipse
In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...
s, hyperbola
Hyperbola
In mathematics a hyperbola is a curve, specifically a smooth curve that lies in a plane, which can be defined either by its geometric properties or by the kinds of equations for which it is the solution set. A hyperbola has two pieces, called connected components or branches, which are mirror...
s, and Cassini oval
Cassini oval
A Cassini oval is a plane curve defined as the set of points in the plane such that the product of the distances to two fixed points is constant. This is related to an ellipse, for which the...
s. However, the term bipolar coordinates is never used to describe coordinates associated with those curves, e.g., elliptic coordinates
Elliptic coordinates
In geometry, the elliptic coordinate system is a two-dimensional orthogonal coordinate system in whichthe coordinate lines are confocal ellipses and hyperbolae...
.
Basic definition
The most common definition of bipolar cylindrical coordinates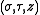 is
is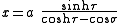
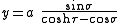

where the
 coordinate of a point
coordinate of a point 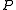
equals the angle
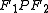 and the
and the coordinate equals the natural logarithm
coordinate equals the natural logarithmNatural logarithm
The natural logarithm is the logarithm to the base e, where e is an irrational and transcendental constant approximately equal to 2.718281828...
of the ratio of the distances
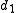 and
and 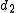 to the focal lines
to the focal lines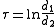
(Recall that the focal lines
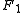 and
and 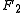 are located at
are located at 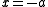 and
and 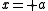 , respectively.)
, respectively.)Surfaces of constant
 correspond to cylinders of different radii
correspond to cylinders of different radii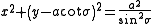
that all pass through the focal lines and are not concentric. The surfaces of constant
 are non-intersecting cylinders of different radii
are non-intersecting cylinders of different radii
that surround the focal lines but again are not concentric. The focal lines and all these cylinders are parallel to the
 -axis (the direction of projection). In the
-axis (the direction of projection). In the 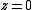 plane, the centers of the constant-
plane, the centers of the constant- and constant-
and constant- cylinders lie on the
cylinders lie on the 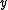 and
and  axes, respectively.
axes, respectively.Scale factors
The scale factors for the bipolar coordinates and
and  are equal
are equal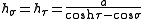
whereas the remaining scale factor
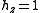 .
.Thus, the infinitesimal volume element equals
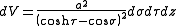
and the Laplacian is given by

Other differential operators such as
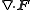
and
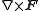 can be expressed in the coordinates
can be expressed in the coordinates 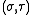 by substituting
by substitutingthe scale factors into the general formulae
found in orthogonal coordinates
Orthogonal coordinates
In mathematics, orthogonal coordinates are defined as a set of d coordinates q = in which the coordinate surfaces all meet at right angles . A coordinate surface for a particular coordinate qk is the curve, surface, or hypersurface on which qk is a constant...
.
Applications
The classic applications of bipolar coordinates are in solving partial differential equations,e.g., Laplace's equation
Laplace's equation
In mathematics, Laplace's equation is a second-order partial differential equation named after Pierre-Simon Laplace who first studied its properties. This is often written as:where ∆ = ∇² is the Laplace operator and \varphi is a scalar function...
or the Helmholtz equation
Helmholtz equation
The Helmholtz equation, named for Hermann von Helmholtz, is the elliptic partial differential equation\nabla^2 A + k^2 A = 0where ∇2 is the Laplacian, k is the wavenumber, and A is the amplitude.-Motivation and uses:...
, for which bipolar coordinates allow a
separation of variables
Separation of variables
In mathematics, separation of variables is any of several methods for solving ordinary and partial differential equations, in which algebra allows one to rewrite an equation so that each of two variables occurs on a different side of the equation....
. A typical example would be the electric field
Electric field
In physics, an electric field surrounds electrically charged particles and time-varying magnetic fields. The electric field depicts the force exerted on other electrically charged objects by the electrically charged particle the field is surrounding...
surrounding two
parallel cylindrical conductors.

