
Beltrami equation
Encyclopedia
In mathematics, the Beltrami equation, named after Eugenio Beltrami
, is the partial differential equation
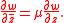
for w a complex distribution of the complex variable z in some open set U, with derivatives that are locally L2, and where μ is a given complex function in L∞(U) of norm less than 1, called the Beltrami coefficient.
Eugenio Beltrami
Eugenio Beltrami was an Italian mathematician notable for his work concerning differential geometry and mathematical physics...
, is the partial differential equation
Partial differential equation
In mathematics, partial differential equations are a type of differential equation, i.e., a relation involving an unknown function of several independent variables and their partial derivatives with respect to those variables...
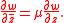
for w a complex distribution of the complex variable z in some open set U, with derivatives that are locally L2, and where μ is a given complex function in L∞(U) of norm less than 1, called the Beltrami coefficient.
See also
- Quasiconformal mappingQuasiconformal mappingIn mathematical complex analysis, a quasiconformal mapping, introduced by and named by , is a homeomorphism between plane domains which to first order takes small circles to small ellipses of bounded eccentricity....
- Measurable Riemann mapping theoremMeasurable Riemann mapping theoremIn the mathematical theory of quasiconformal mappings in two dimensions, the measurable Riemann mapping theorem, proved by , generalizes the Riemann mapping theorem from conformal to quasiconformal homeomorphisms, and is stated as follows...
- Isothermal coordinatesIsothermal coordinatesIn mathematics, specifically in differential geometry, isothermal coordinates on a Riemannian manifoldare local coordinates where the metric isconformal to the Euclidean metric...

