.gif)
Band (algebra)
Encyclopedia
In mathematics
, a band (also called idempotent semigroup) is a semigroup
in which every element is idempotent (in other words equal to its own square). Bands were first studied and named by ; the lattice
of varieties
of bands was described independently in the early 1970s by Biryukov, Fennemore and Gerhard. Semilattices, left-zero bands, right-zero bands, rectangular bands, normal bands, and regular bands, specific subclasses of bands which lie near the bottom of this lattice, are of particular interest and are briefly described below.
if it is closed under formation of subsemigroups, homomorphic images
and direct product
. Each variety of bands can be defined by a single defining identity
.
whence its Cayley table
has constant rows.
Symmetrically, a right zero band is one satisfying
so that the Cayley table has constant columns.
Equivalently,
For example, given arbitrary non-empty sets I and J one can define a semigroup operation on I × J by setting
The resulting semigroup is a rectangular band because
In fact, any rectangular band is isomorphic to one of the above form. Left zero and right zero bands are rectangular bands, and in fact every rectangular band is isomorphic to a direct product of a left zero band and a right zero band. All rectangular bands of prime order are zero bands, either left or right. A rectangular band is said to be purely rectangular if it is not a left or right zero band.
This is the same equation used to define medial magmas
, and so a normal band may also be called a medial band, and normal bands are examples of medial magmas.
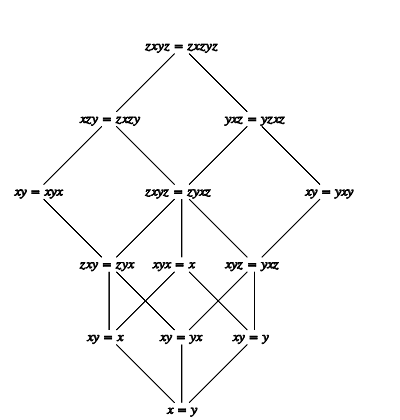When partially ordered
by inclusion, varieties of bands naturally form a lattice
, in which the meet of two varieties is their intersection and the join of two varieties is the smallest variety that contains both of them. The complete structure of this lattice is known; in particular, it is countable, complete
, and distributive
. The sublattice of the 13 varieties of regular bands is shown in the figure. The varieties of left-zero bands, semilattices, and right-zero bands are the three atoms (non-trivial minimal elements) of this lattice.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a band (also called idempotent semigroup) is a semigroup
Semigroup
In mathematics, a semigroup is an algebraic structure consisting of a set together with an associative binary operation. A semigroup generalizes a monoid in that there might not exist an identity element...
in which every element is idempotent (in other words equal to its own square). Bands were first studied and named by ; the lattice
Lattice (order)
In mathematics, a lattice is a partially ordered set in which any two elements have a unique supremum and an infimum . Lattices can also be characterized as algebraic structures satisfying certain axiomatic identities...
of varieties
Variety (universal algebra)
In mathematics, specifically universal algebra, a variety of algebras is the class of all algebraic structures of a given signature satisfying a given set of identities. Equivalently, a variety is a class of algebraic structures of the same signature which is closed under the taking of homomorphic...
of bands was described independently in the early 1970s by Biryukov, Fennemore and Gerhard. Semilattices, left-zero bands, right-zero bands, rectangular bands, normal bands, and regular bands, specific subclasses of bands which lie near the bottom of this lattice, are of particular interest and are briefly described below.
Varieties of bands
A class of bands forms a varietyVariety (universal algebra)
In mathematics, specifically universal algebra, a variety of algebras is the class of all algebraic structures of a given signature satisfying a given set of identities. Equivalently, a variety is a class of algebraic structures of the same signature which is closed under the taking of homomorphic...
if it is closed under formation of subsemigroups, homomorphic images
Homomorphism
In abstract algebra, a homomorphism is a structure-preserving map between two algebraic structures . The word homomorphism comes from the Greek language: ὁμός meaning "same" and μορφή meaning "shape".- Definition :The definition of homomorphism depends on the type of algebraic structure under...
and direct product
Direct product
In mathematics, one can often define a direct product of objectsalready known, giving a new one. This is generally the Cartesian product of the underlying sets, together with a suitably defined structure on the product set....
. Each variety of bands can be defined by a single defining identity
Variety (universal algebra)
In mathematics, specifically universal algebra, a variety of algebras is the class of all algebraic structures of a given signature satisfying a given set of identities. Equivalently, a variety is a class of algebraic structures of the same signature which is closed under the taking of homomorphic...
.
Semilattices
Semilattices are exactly commutative bands; that is, they are the bands satisfying the equation- xy = yx for all x and y.
Zero bands
A left zero band is a band satisfying the equation- xy = x,
whence its Cayley table
Cayley table
A Cayley table, after the 19th century British mathematician Arthur Cayley, describes the structure of a finite group by arranging all the possible products of all the group's elements in a square table reminiscent of an addition or multiplication table...
has constant rows.
Symmetrically, a right zero band is one satisfying
- xy = y,
so that the Cayley table has constant columns.
Rectangular bands
A rectangular band is a band S which satisfies- xyx = x for all x, y ∈ S.
Equivalently,
- xyz = xz.
For example, given arbitrary non-empty sets I and J one can define a semigroup operation on I × J by setting
The resulting semigroup is a rectangular band because
- for any pair (i, j) we have (i, j) · (i, j) = (i, j)
- for any two pairs (ix, jx), (iy, jy) we have
In fact, any rectangular band is isomorphic to one of the above form. Left zero and right zero bands are rectangular bands, and in fact every rectangular band is isomorphic to a direct product of a left zero band and a right zero band. All rectangular bands of prime order are zero bands, either left or right. A rectangular band is said to be purely rectangular if it is not a left or right zero band.
Normal bands
A normal band is a band S satisfying- xyzx = xzyx for all x, y, and z ∈ S.
This is the same equation used to define medial magmas
Medial
- Medial magmas :In abstract algebra, a medial magma is a set with a binary operation which satisfies the identity \cdot = \cdot , or more simply, xy\cdot uv = xu\cdot yv...
, and so a normal band may also be called a medial band, and normal bands are examples of medial magmas.
Regular bands
A regular band is a band S satisfying- xyxzx = xyzx for all x, y, z ∈ S
Lattice of varieties
Partially ordered set
In mathematics, especially order theory, a partially ordered set formalizes and generalizes the intuitive concept of an ordering, sequencing, or arrangement of the elements of a set. A poset consists of a set together with a binary relation that indicates that, for certain pairs of elements in the...
by inclusion, varieties of bands naturally form a lattice
Lattice (order)
In mathematics, a lattice is a partially ordered set in which any two elements have a unique supremum and an infimum . Lattices can also be characterized as algebraic structures satisfying certain axiomatic identities...
, in which the meet of two varieties is their intersection and the join of two varieties is the smallest variety that contains both of them. The complete structure of this lattice is known; in particular, it is countable, complete
Complete lattice
In mathematics, a complete lattice is a partially ordered set in which all subsets have both a supremum and an infimum . Complete lattices appear in many applications in mathematics and computer science...
, and distributive
Distributive lattice
In mathematics, distributive lattices are lattices for which the operations of join and meet distribute over each other. The prototypical examples of such structures are collections of sets for which the lattice operations can be given by set union and intersection...
. The sublattice of the 13 varieties of regular bands is shown in the figure. The varieties of left-zero bands, semilattices, and right-zero bands are the three atoms (non-trivial minimal elements) of this lattice.