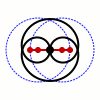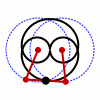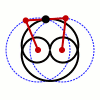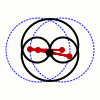
In mathematics, Watt's curve is a tricircular

Circular algebraic curve

In geometry, a circular algebraic curve is a type of plane algebraic curve determined by an equation F = 0, where F is a polynomial with real coefficients and the highest-order terms of F form a polynomial divisible by x2 + y2...

 plane algebraic curve

Algebraic curve

In algebraic geometry, an algebraic curve is an algebraic variety of dimension one. The theory of these curves in general was quite fully developed in the nineteenth century, after many particular examples had been considered, starting with circles and other conic sections.- Plane algebraic curves...

 of degree six. It is generated by two circles of radius b with centers distance 2a apart (taken to be at (±a, 0). A line segment of length 2c attaches to a point on each of the circles, and the midpoint of the line segment traces out the Watt curve as the circles rotate. It arose in connection with James Watt

James Watt

James Watt, FRS, FRSE was a Scottish inventor and mechanical engineer whose improvements to the Newcomen steam engine were fundamental to the changes brought by the Industrial Revolution in both his native Great Britain and the rest of the world.While working as an instrument maker at the...

's pioneering work on the steam engine.

The equation of the curve can be given in polar coordinates as

Polar coordinates

The polar equation for the curve can be derived as follows:
Working in the complex plane

Complex plane

In mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...

, let the centers of the circles be at a and −a, and the connecting segment have endpoints at −a+be^{i λ} and a+be^{i ρ}. Let the angle of inclination of the segment be ψ with its midpoint at re^{i θ}. Then the endpoints are also given by re^{i θ} ± ce^{i ψ}. Setting expressions for the same points equal to each other gives
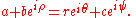
Add these and divide by two to get
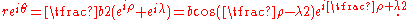
Comparing radii and arguments gives
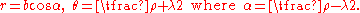
Similarly, subtracting the first two equations and dividing by 2 gives
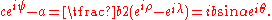
Write
Then

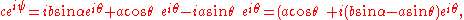
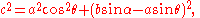
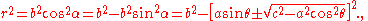
Cartesian coordinates

Expanding the polar equation gives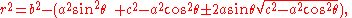
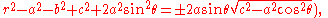
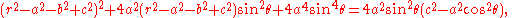
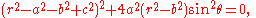
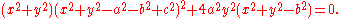
Letting d²=a²+b²-c² simplifies this to

Form of the curve

The construction requires a quadrilateral with sides 2a, b, 2c, b. Any side must be less than the sum of the remaining sides, so the curve is empty (at least in the real plane) unless a<b+c and c<b+a.

The has a crossing point at the origin if there is a triangle with sides a, b and c. Given the previous conditions, this means that the curve crosses the origin if and only if b<a+c. If b=a+c then two branches of the curve meet at the origin with a common vertical tangent, making it a quadruple point.

Given b<a+c, the shape of the curve is determined by the relative magnitude of b and d. If d is imaginary, that is if a²+b²=<c² then the curve has fthe form of a figure eight. If d is 0 then the curve is a figure eight with two branches of the curve having a common horizontal tangent at the origin. If 0<d<b then the curve has two additional double points at ±d and the curve crosses itself at these points. The overall shape of the curve is pretzel-like in this case. If d=b then a=c and the curve decomposes into a circle of radius b and a lemniscate of Booth

Hippopede

In geometry, a hippopede is a plane curve determined by an equation of the form^2=cx^2+dy^2,...

, a figure eight shaped curve. A special case of this is a=c, b=√2c which produces the lemniscate of Bernoulli

Lemniscate of Bernoulli

In geometry, the lemniscate of Bernoulli is a plane curve defined from two given points F1 and F2, known as foci, at distance 2a from each other as the locus of points P so that PF1·PF2 = a2. The curve has a shape similar to the numeral 8 and to the ∞ symbol. Its name is from lemniscus, which is...

. Finally, if d>b then the points ±d are still solutions to the Cartesian equation of the curve, but the curve does not cross these points and they are acnode

Acnode

An acnode is an isolated point not on a curve, but whose coordinates satisfy the equation of the curve. The term "isolated point" or "hermit point" is an equivalent term....

s. The curve again has a figure eight shape though the shape is distorted if d is close to b.

Given b>a+c, the shape of the curve is determined by the relative sizes of a and c. If a<c then the curve has the form of two loops that cross each other at ±d. If a=c then the curve decomposes into a into a circle of radius b and an oval of Booth

Hippopede

In geometry, a hippopede is a plane curve determined by an equation of the form^2=cx^2+dy^2,...

. If a>c then the curve does not cross the x-axis at all and consists of two flattened ovals.

Watt's linkage

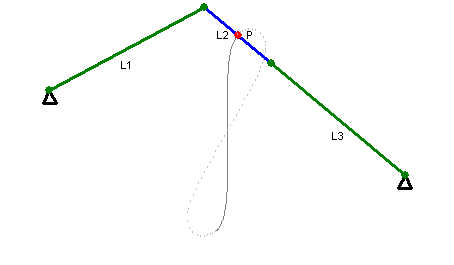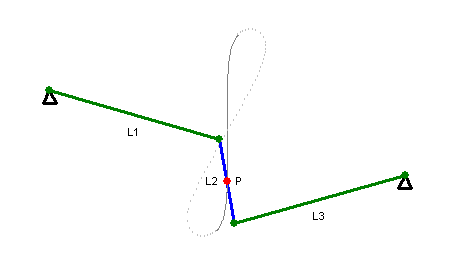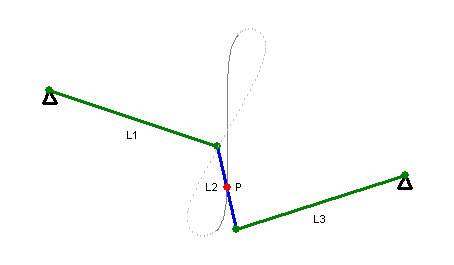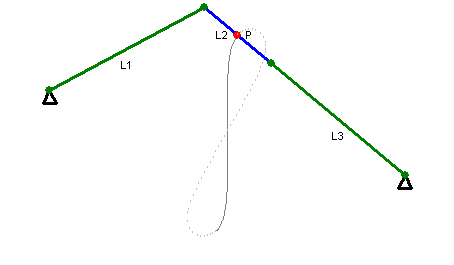
When the curve crosses the origin, the origin is a point of inflection and therefore has contact of order 3 with a tangent. However, if a²=b²+<c² then tangent has contact of order 5 with the tangent, in other words the curve is a close approximation of a straight line. This is the basis for Watt's linkage.