Symplectic cut
Encyclopedia
In mathematics
, specifically in symplectic geometry, the symplectic cut is a geometric modification on symplectic manifold
s. Its effect is to decompose a given manifold into two pieces. There is an inverse operation, the symplectic sum
, that glues two manifolds together into one. The symplectic cut can also be viewed as a generalization of symplectic blow up
. The cut was introduced in 1995 by Eugene Lerman, who used it to study the symplectic quotient
and other operations on manifolds.
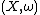be any symplectic manifold and
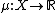
a Hamiltonian
on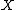
. Let 
be any regular value of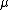 
, so that the level set 
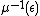is a smooth manifold. Assume furthermore that 
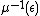is fibered in circles, each of which is an integral curve of the induced Hamiltonian vector field
.
Under these assumptions,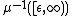
is a manifold with boundary 
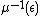, and one can form a manifold
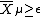
by collapsing each circle fiber to a point. In other words,
is 
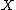with the subset 
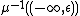removed and the boundary collapsed along each circle fiber. The quotient of the boundary is a submanifold of 
of codimension
two, denoted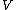
.
Similarly, one may form from
a manifold 
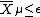, which also contains a copy of 
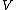. The symplectic cut is the pair of manifolds 
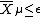and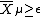 
.
Sometimes it is useful to view the two halves of the symplectic cut as being joined along their shared submanifold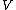
to produce a singular space
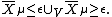
For example, this singular space is the central fiber in the symplectic sum regarded as a deformation.
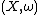be any symplectic manifold. Assume that the circle group 
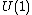acts
on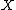
in a Hamiltonian
way with moment map
This moment map can be viewed as a Hamiltonian function that generates the circle action. The product space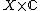
, with coordinate 
on 
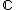, comes with an induced symplectic form
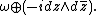
The group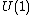
acts on the product in a Hamiltonian way by
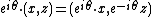
with moment map
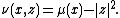
Let
be any real number such that the circle action is free on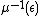 
. Then 
is a regular value of 
, and 
is a manifold.
This manifold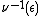
contains as a submanifold the set of points 
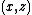with 
and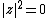 
; this submanifold is naturally identified with 
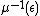. The complement of the submanifold, which consists of points 
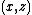with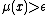 
, is naturally identified with the product of
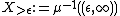
and the circle.
The manifold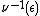
inherits the Hamiltonian circle action, as do its two submanifolds just described. So one may form the symplectic quotient
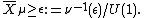
By construction, it contains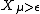
as a dense open submanifold; essentially, it compactifies this open manifold with the symplectic quotient
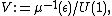
which is a symplectic submanifold of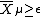
of codimension two.
If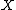
is Kähler
, then so is the cut space
; however, the embedding of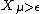 
is not an isometry.
One constructs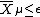
, the other half of the symplectic cut, in a symmetric manner. The normal bundle
s of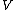
in the two halves of the cut are opposite each other (meaning symplectically anti-isomorphic). The symplectic sum of 
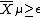and 
along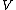 
recovers 
.
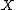The existence of a global Hamiltonian circle action on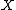
appears to be a restrictive assumption. However, it is not actually necessary; the cut can be performed under more general hypotheses, such as a local Hamiltonian circle action near 
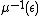(since the cut is a local operation).
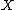is blown up along a submanifold 
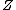, the blow up locus
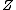is replaced by an exceptional divisor
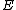and the rest of the manifold is left undisturbed. Topologically, this operation may also be viewed as the removal of an 
-neighborhood of the blow up locus, followed by the collapse of the boundary by the Hopf map.
Blowing up a symplectic manifold is more subtle, since the symplectic form must be adjusted in a neighborhood of the blow up locus in order to continue smoothly across the exceptional divisor in the blow up. The symplectic cut is an elegant means of making the neighborhood-deletion/boundary-collapse process symplectically rigorous.
As before, let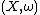
be a symplectic manifold with a Hamiltonian 
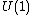-action with moment map 
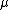. Assume that the moment map is proper and that it achieves its maximum 
exactly along a symplectic submanifold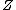 
of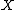 
. Assume furthermore that the weights of the isotropy representation of 
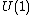on the normal bundle 
are all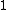 
.
Then for small
the only critical points in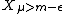 
are those on 
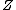. The symplectic cut 
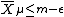, which is formed by deleting a symplectic 
-neighborhood of 
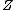and collapsing the boundary, is then the symplectic blow up of 
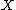along 
.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, specifically in symplectic geometry, the symplectic cut is a geometric modification on symplectic manifold
Symplectic manifold
In mathematics, a symplectic manifold is a smooth manifold, M, equipped with a closed nondegenerate differential 2-form, ω, called the symplectic form. The study of symplectic manifolds is called symplectic geometry or symplectic topology...
s. Its effect is to decompose a given manifold into two pieces. There is an inverse operation, the symplectic sum
Symplectic sum
In mathematics, specifically in symplectic geometry, the symplectic sum is a geometric modification on symplectic manifolds, which glues two given manifolds into a single new one. It is a symplectic version of connected summation along a submanifold, often called a fiber sum.The symplectic sum is...
, that glues two manifolds together into one. The symplectic cut can also be viewed as a generalization of symplectic blow up
Blowing up
In mathematics, blowing up or blowup is a type of geometric transformation which replaces a subspace of a given space with all the directions pointing out of that subspace. For example, the blowup of a point in a plane replaces the point with the projectivized tangent space at that point...
. The cut was introduced in 1995 by Eugene Lerman, who used it to study the symplectic quotient
Moment map
In mathematics, specifically in symplectic geometry, the momentum map is a tool associated with a Hamiltonian action of a Lie group on a symplectic manifold, used to construct conserved quantities for the action. The moment map generalizes the classical notions of linear and angular momentum...
and other operations on manifolds.
Topological description
Let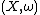 be any symplectic manifold and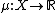a Hamiltonian
Hamiltonian vector field
In mathematics and physics, a Hamiltonian vector field on a symplectic manifold is a vector field, defined for any energy function or Hamiltonian. Named after the physicist and mathematician Sir William Rowan Hamilton, a Hamiltonian vector field is a geometric manifestation of Hamilton's equations...
on
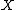. Let be any regular value of 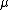, so that the level set 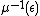is a smooth manifold. Assume furthermore that 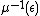is fibered in circles, each of which is an integral curve of the induced Hamiltonian vector fieldHamiltonian vector field
In mathematics and physics, a Hamiltonian vector field on a symplectic manifold is a vector field, defined for any energy function or Hamiltonian. Named after the physicist and mathematician Sir William Rowan Hamilton, a Hamiltonian vector field is a geometric manifestation of Hamilton's equations...
.
Under these assumptions,
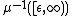is a manifold with boundary 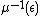, and one can form a manifold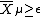by collapsing each circle fiber to a point. In other words,
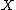is with the subset 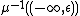removed and the boundary collapsed along each circle fiber. The quotient of the boundary is a submanifold of of codimensionCodimension
In mathematics, codimension is a basic geometric idea that applies to subspaces in vector spaces, and also to submanifolds in manifolds, and suitable subsets of algebraic varieties.The dual concept is relative dimension.-Definition:...
two, denoted
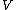.Similarly, one may form from
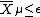a manifold , which also contains a copy of 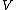. The symplectic cut is the pair of manifolds 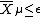and 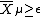.Sometimes it is useful to view the two halves of the symplectic cut as being joined along their shared submanifold
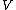to produce a singular space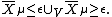For example, this singular space is the central fiber in the symplectic sum regarded as a deformation.
Symplectic description
The preceding description is rather crude; more care is required to keep track of the symplectic structure on the symplectic cut. For this, let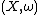 be any symplectic manifold. Assume that the circle group 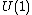actsGroup action
In algebra and geometry, a group action is a way of describing symmetries of objects using groups. The essential elements of the object are described by a set, and the symmetries of the object are described by the symmetry group of this set, which consists of bijective transformations of the set...
on
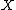in a HamiltonianMoment map
In mathematics, specifically in symplectic geometry, the momentum map is a tool associated with a Hamiltonian action of a Lie group on a symplectic manifold, used to construct conserved quantities for the action. The moment map generalizes the classical notions of linear and angular momentum...
way with moment map
Moment map
In mathematics, specifically in symplectic geometry, the momentum map is a tool associated with a Hamiltonian action of a Lie group on a symplectic manifold, used to construct conserved quantities for the action. The moment map generalizes the classical notions of linear and angular momentum...
This moment map can be viewed as a Hamiltonian function that generates the circle action. The product space
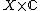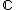, with coordinate on , comes with an induced symplectic form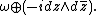The group
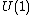acts on the product in a Hamiltonian way by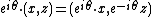with moment map
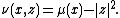
Let
be any real number such that the circle action is free on 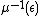. Then is a regular value of , and is a manifold.This manifold
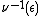contains as a submanifold the set of points 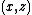with and 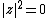; this submanifold is naturally identified with 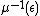. The complement of the submanifold, which consists of points 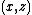with 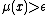, is naturally identified with the product of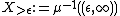and the circle.
The manifold
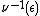inherits the Hamiltonian circle action, as do its two submanifolds just described. So one may form the symplectic quotient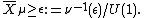By construction, it contains
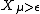as a dense open submanifold; essentially, it compactifies this open manifold with the symplectic quotient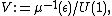which is a symplectic submanifold of
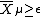of codimension two.If
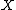is KählerKähler manifold
In mathematics, a Kähler manifold is a manifold with unitary structure satisfying an integrability condition.In particular, it is a Riemannian manifold, a complex manifold, and a symplectic manifold, with these three structures all mutually compatible.This threefold structure corresponds to the...
, then so is the cut space
; however, the embedding of 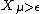is not an isometry.One constructs
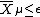, the other half of the symplectic cut, in a symmetric manner. The normal bundleNormal bundle
In differential geometry, a field of mathematics, a normal bundle is a particular kind of vector bundle, complementary to the tangent bundle, and coming from an embedding .-Riemannian manifold:...
s of
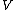in the two halves of the cut are opposite each other (meaning symplectically anti-isomorphic). The symplectic sum of 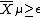and along 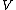recovers 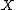.The existence of a global Hamiltonian circle action on
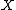appears to be a restrictive assumption. However, it is not actually necessary; the cut can be performed under more general hypotheses, such as a local Hamiltonian circle action near 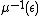(since the cut is a local operation).Blow up as cut
When a complex manifoldComplex manifold
In differential geometry, a complex manifold is a manifold with an atlas of charts to the open unit disk in Cn, such that the transition maps are holomorphic....
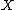is blown up along a submanifold 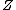, the blow up locusLocus (mathematics)
In geometry, a locus is a collection of points which share a property. For example a circle may be defined as the locus of points in a plane at a fixed distance from a given point....
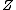is replaced by an exceptional divisorExceptional divisor
In mathematics, specifically algebraic geometry, an exceptional divisor for a regular mapf: X \rightarrow Yof varieties is a kind of 'large' subvariety of X which is 'crushed' by f, in a certain definite sense...
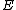and the rest of the manifold is left undisturbed. Topologically, this operation may also be viewed as the removal of an -neighborhood of the blow up locus, followed by the collapse of the boundary by the Hopf map.Blowing up a symplectic manifold is more subtle, since the symplectic form must be adjusted in a neighborhood of the blow up locus in order to continue smoothly across the exceptional divisor in the blow up. The symplectic cut is an elegant means of making the neighborhood-deletion/boundary-collapse process symplectically rigorous.
As before, let
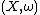be a symplectic manifold with a Hamiltonian 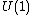-action with moment map 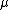. Assume that the moment map is proper and that it achieves its maximum exactly along a symplectic submanifold 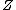of 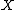. Assume furthermore that the weights of the isotropy representation of 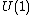on the normal bundle are all 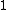.Then for small
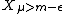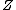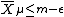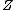the only critical points in are those on . The symplectic cut , which is formed by deleting a symplectic -neighborhood of and collapsing the boundary, is then the symplectic blow up of 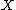along .