
Stewart-Walker lemma
Encyclopedia
The Stewart–Walker lemma provides necessary and sufficient conditions for the linear
perturbation of a tensor
field to be gauge
-invariant.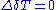 if and only if
if and only if
one of the following holds
1.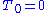
2.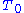 is a constant scalar field
is a constant scalar field
3.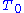 is a linear combination of products of delta functions
is a linear combination of products of delta functions 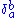
 with
with  has metric
has metric
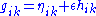 . These manifolds can be put together to form a 5-manifold
. These manifolds can be put together to form a 5-manifold  . A smooth curve
. A smooth curve  can be constructed through
can be constructed through  with tangent 5-vector
with tangent 5-vector  , transverse to
, transverse to  . If
. If 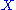 is defined so that if
is defined so that if 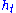 is the family of 1-parameter maps which map
is the family of 1-parameter maps which map  and
and 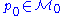 then a point
then a point 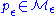 can be written as
can be written as 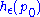 . This also defines a pull back
. This also defines a pull back 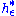 that maps a tensor field
that maps a tensor field 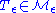 back onto
back onto 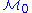 . Given sufficient smoothness a Taylor expansion can be defined
. Given sufficient smoothness a Taylor expansion can be defined
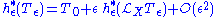
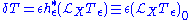 is the linear perturbation of
is the linear perturbation of  . However, since the choice of
. However, since the choice of 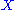 is dependent on the choice of gauge
is dependent on the choice of gauge
another gauge can be taken. Therefore the differences in gauge become . Picking a chart where
. Picking a chart where 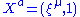 and
and  then
then 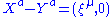 which is a well defined vector in any
which is a well defined vector in any 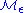 and gives the result
and gives the result

The only three possible ways this can be satisfied are those of the lemma.
Linear
In mathematics, a linear map or function f is a function which satisfies the following two properties:* Additivity : f = f + f...
perturbation of a tensor
Tensor
Tensors are geometric objects that describe linear relations between vectors, scalars, and other tensors. Elementary examples include the dot product, the cross product, and linear maps. Vectors and scalars themselves are also tensors. A tensor can be represented as a multi-dimensional array of...
field to be gauge
Gauge theory
In physics, gauge invariance is the property of a field theory in which different configurations of the underlying fundamental but unobservable fields result in identical observable quantities. A theory with such a property is called a gauge theory...
-invariant.
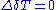 if and only if
if and only ifIf and only if
In logic and related fields such as mathematics and philosophy, if and only if is a biconditional logical connective between statements....
one of the following holds
1.
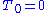
2.
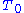 is a constant scalar field
is a constant scalar field3.
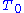 is a linear combination of products of delta functions
is a linear combination of products of delta functions 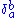
Derivation
A 1-parameter family of manifolds denoted by with
with  has metric
has metricMetric (mathematics)
In mathematics, a metric or distance function is a function which defines a distance between elements of a set. A set with a metric is called a metric space. A metric induces a topology on a set but not all topologies can be generated by a metric...
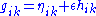 . These manifolds can be put together to form a 5-manifold
. These manifolds can be put together to form a 5-manifold  . A smooth curve
. A smooth curve 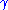 can be constructed through
can be constructed through  with tangent 5-vector
with tangent 5-vector  , transverse to
, transverse to 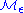 . If
. If 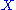 is defined so that if
is defined so that if 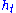 is the family of 1-parameter maps which map
is the family of 1-parameter maps which map  and
and 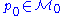 then a point
then a point 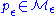 can be written as
can be written as 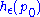 . This also defines a pull back
. This also defines a pull back 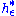 that maps a tensor field
that maps a tensor field 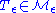 back onto
back onto 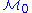 . Given sufficient smoothness a Taylor expansion can be defined
. Given sufficient smoothness a Taylor expansion can be defined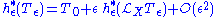
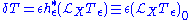 is the linear perturbation of
is the linear perturbation of  . However, since the choice of
. However, since the choice of 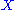 is dependent on the choice of gauge
is dependent on the choice of gaugeGauge theory
In physics, gauge invariance is the property of a field theory in which different configurations of the underlying fundamental but unobservable fields result in identical observable quantities. A theory with such a property is called a gauge theory...
another gauge can be taken. Therefore the differences in gauge become
 . Picking a chart where
. Picking a chart where 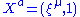 and
and  then
then 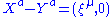 which is a well defined vector in any
which is a well defined vector in any 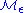 and gives the result
and gives the result
The only three possible ways this can be satisfied are those of the lemma.

