Scalar-tensor-vector gravity
Encyclopedia
Scalar–tensor–vector gravity (STVG) is a modified theory of gravity developed by John Moffat, a researcher at the Perimeter Institute for Theoretical Physics
in Waterloo, Ontario
. The theory is also often referred to by the acronym MOG (MOdified Gravity).
, while elevating the three constants of the theory to scalar fields. In the weak-field approximation, STVG produces a Yukawa-like modification of the gravitational force due to a point source. Intuitively, this result can be described as follows: far from a source gravity is stronger than the Newtonian prediction, but at shorter distances, it is counteracted by a repulsive fifth force
due to the vector field.
STVG has been used successfully to explain galaxy rotation curve
s, the mass profiles of galaxy clusters, gravitational lensing in the Bullet Cluster
, and cosmological observations without the need for dark matter
. On a smaller scale, in the solar system, STVG predicts no observable deviation from general relativity. The theory may also offer an explanation for the origin of inertia
.
signature of
will be used; the speed of light is set to 
, and we are using the following definition for the Ricci tensor:
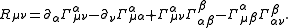
We begin with the Einstein-Hilbert Lagrangian:
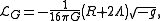
where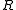
is the trace of the Ricci tensor, 
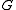is the gravitational constant, 
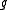is the determinant of the metric tensor 
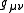, while 
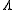is the cosmological constant.
We introduce the Maxwell-Proca
Lagrangian
for the STVG vector field
:
where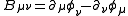
, 
is the mass of the vector field, 
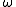characterizes the strength of the coupling between the fifth force and matter, and 
is a self-interaction potential.
The three constants of the theory,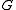
, 
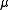and 
, are promoted to scalar fields by introducing associated kinetic and potential terms in the Lagrangian density:
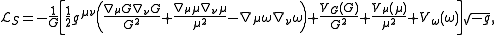
where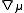
denotes covariant differentiation with respect to the metric 
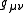, while 
, 
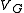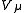, and 
are the self-interaction potentials associated with the scalar fields.
The STVG action integral takes the form
where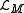
is the ordinary matter Lagrangian density.
. First a test particle Lagrangian is postulated in the form
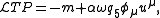
where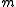
is the test particle mass, 
is a factor representing the nonlinearity of the theory, 
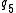is the test particle's fifth-force charge, and 
is its four-velocity. Assuming that the fifth-force charge is proportional to mass, i.e.,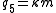 
, the value of 
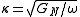is determined and the following equation of motion is obtained in the spherically symmetric, static gravitational field of a point mass of mass 
:
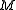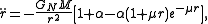
where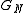
is Newton's constant of gravitation. Further study of the field equations allows a determination of 
and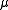 
for a point gravitational source of mass 
in the form
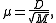
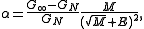
where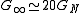
is determined from cosmological observations, while for the constants 
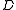and 
galaxy rotation curves yield the following values:
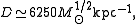
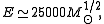
where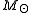
is the mass of the Sun
. These results form the basis of a series of calculations that are used to confront the theory with observation.
On the scale of the solar system, the theory predicts no deviation from the results of Newton and Einstein. This is also true for star clusters containing no more than a maximum of a few million solar masses.
The theory accounts for the rotation curves of spiral galaxies, correctly reproducing the Tully-Fisher law.
STVG is in good agreement with the mass profiles of galaxy clusters.
STVG can also account for key cosmological observations, including:
Perimeter Institute for Theoretical Physics
Perimeter Institute for Theoretical Physics is an independent, resident-based research institute devoted to foundational issues in theoretical physics located in Waterloo, Ontario, Canada. Perimeter Institute was founded in 1999 by Mike Lazaridis...
in Waterloo, Ontario
Waterloo, Ontario
Waterloo is a city in Southern Ontario, Canada. It is the smallest of the three cities in the Regional Municipality of Waterloo, and is adjacent to the city of Kitchener....
. The theory is also often referred to by the acronym MOG (MOdified Gravity).
Overview
Scalar–vector–tensor gravity theory, also known as MOdified Gravity (MOG), is based on an action principle and postulates the existence of a vector fieldVector field
In vector calculus, a vector field is an assignmentof a vector to each point in a subset of Euclidean space. A vector field in the plane for instance can be visualized as an arrow, with a given magnitude and direction, attached to each point in the plane...
, while elevating the three constants of the theory to scalar fields. In the weak-field approximation, STVG produces a Yukawa-like modification of the gravitational force due to a point source. Intuitively, this result can be described as follows: far from a source gravity is stronger than the Newtonian prediction, but at shorter distances, it is counteracted by a repulsive fifth force
Fifth force
Occasionally, physicists have postulated the existence of a fifth force in addition to the four known fundamental forces. The force is generally believed to have roughly the strength of gravity Occasionally, physicists have postulated the existence of a fifth force in addition to the four known...
due to the vector field.
STVG has been used successfully to explain galaxy rotation curve
Galaxy rotation curve
The rotation curve of a galaxy can be represented by a graph that plots the orbital velocity of the stars or gas in the galaxy on the y-axis against the distance from the center of the galaxy on the x-axis....
s, the mass profiles of galaxy clusters, gravitational lensing in the Bullet Cluster
Bullet cluster
The Bullet cluster consists of two colliding clusters of galaxies. Studies of the Bullet cluster, announced in August 2006, provide the best evidence to date for the existence of dark matter...
, and cosmological observations without the need for dark matter
Dark matter
In astronomy and cosmology, dark matter is matter that neither emits nor scatters light or other electromagnetic radiation, and so cannot be directly detected via optical or radio astronomy...
. On a smaller scale, in the solar system, STVG predicts no observable deviation from general relativity. The theory may also offer an explanation for the origin of inertia
Inertia
Inertia is the resistance of any physical object to a change in its state of motion or rest, or the tendency of an object to resist any change in its motion. It is proportional to an object's mass. The principle of inertia is one of the fundamental principles of classical physics which are used to...
.
Mathematical details
STVG is formulated using the action principle. In the following discussion, a metricMetric (mathematics)
In mathematics, a metric or distance function is a function which defines a distance between elements of a set. A set with a metric is called a metric space. A metric induces a topology on a set but not all topologies can be generated by a metric...
signature of
will be used; the speed of light is set to , and we are using the following definition for the Ricci tensor: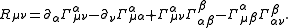We begin with the Einstein-Hilbert Lagrangian:
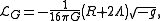
where
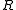is the trace of the Ricci tensor, 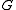is the gravitational constant, 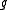is the determinant of the metric tensor 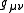, while 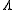is the cosmological constant.We introduce the Maxwell-Proca
Proca action
In physics, in the area of field theory, the Proca action describes a massive spin-1 field of mass m in Minkowski spacetime. The field involved is a real vector field A...
Lagrangian
Lagrangian
The Lagrangian, L, of a dynamical system is a function that summarizes the dynamics of the system. It is named after Joseph Louis Lagrange. The concept of a Lagrangian was originally introduced in a reformulation of classical mechanics by Irish mathematician William Rowan Hamilton known as...
for the STVG vector field
:where
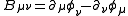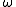, is the mass of the vector field, characterizes the strength of the coupling between the fifth force and matter, and is a self-interaction potential.The three constants of the theory,
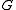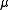, and , are promoted to scalar fields by introducing associated kinetic and potential terms in the Lagrangian density: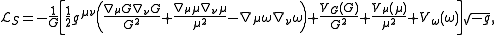where
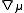denotes covariant differentiation with respect to the metric 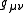, while 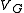, , 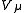and are the self-interaction potentials associated with the scalar fields.The STVG action integral takes the form
where
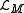is the ordinary matter Lagrangian density.Spherically symmetric, static vacuum solution
The field equations of STVG can be developed from the action integral using the variational principleVariational principle
A variational principle is a scientific principle used within the calculus of variations, which develops general methods for finding functions which minimize or maximize the value of quantities that depend upon those functions...
. First a test particle Lagrangian is postulated in the form
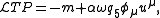
where
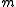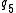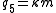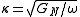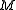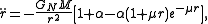
is the test particle mass, is a factor representing the nonlinearity of the theory, is the test particle's fifth-force charge, and is its four-velocity. Assuming that the fifth-force charge is proportional to mass, i.e., , the value of is determined and the following equation of motion is obtained in the spherically symmetric, static gravitational field of a point mass of mass :where
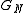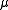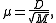
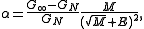
is Newton's constant of gravitation. Further study of the field equations allows a determination of and for a point gravitational source of mass in the formwhere
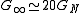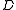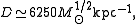
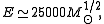
is determined from cosmological observations, while for the constants and galaxy rotation curves yield the following values:where
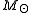is the mass of the SunSun
The Sun is the star at the center of the Solar System. It is almost perfectly spherical and consists of hot plasma interwoven with magnetic fields...
. These results form the basis of a series of calculations that are used to confront the theory with observation.
Observations
STVG/MOG has been applied successfully to a range of astronomical, astrophysical, and cosmological phenomena.On the scale of the solar system, the theory predicts no deviation from the results of Newton and Einstein. This is also true for star clusters containing no more than a maximum of a few million solar masses.
The theory accounts for the rotation curves of spiral galaxies, correctly reproducing the Tully-Fisher law.
STVG is in good agreement with the mass profiles of galaxy clusters.
STVG can also account for key cosmological observations, including:
- The acoustic peaks in the cosmic microwave background radiation;
- The accelerating expansion of the universeAccelerating universeThe accelerating universe is the observation that the universe appears to be expanding at an increasing rate, which in formal terms means that the cosmic scale factor a has a positive second derivative, implying that the velocity at which a given galaxy is receding from us should be continually...
that is apparent from type Ia supernovaType Ia supernovaA Type Ia supernova is a sub-category of supernovae, which in turn are a sub-category of cataclysmic variable stars, that results from the violent explosion of a white dwarf star. A white dwarf is the remnant of a star that has completed its normal life cycle and has ceased nuclear fusion...
observations; - The matter power spectrumMatter power spectrumThe matter power spectrum describes the density contrast of the universe as a function of scale. It is the Fourier transform of the matter correlation function. On large scales, gravity competes with cosmic expansion, and structures grow according to linear theory...
of the universe that is observed in the form of galaxy-galaxy correlations.
See also
- Modified Newtonian DynamicsModified Newtonian dynamicsIn physics, Modified Newtonian dynamics is a hypothesis that proposes a modification of Newton's law of gravity to explain the galaxy rotation problem. When the uniform velocity of rotation of galaxies was first observed, it was unexpected because Newtonian theory of gravity predicts that objects...
- Tensor–vector–scalar gravity
- Nonsymmetric Gravitational TheoryNonsymmetric gravitational theoryIn theoretical physics, the nonsymmetric gravitational theory of John Moffat is a classical theory of gravitation which tries to explain the observation of the flat rotation curves of galaxies....