
Sanov's theorem
Encyclopedia
In information theory
, Sanov's theorem gives a bound on the probability of observing an atypical
sequence of samples from a given probability distribution
.
Let A be a set of probability distributions over an alphabet X, and let q be an arbitrary distribution over X (where q may or may not be in A). Suppose we draw n i.i.d.
samples from q, represented by the vector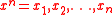 . Then
. Then
 ,
,
where
Furthermore, if A is a closed set
,

Information theory
Information theory is a branch of applied mathematics and electrical engineering involving the quantification of information. Information theory was developed by Claude E. Shannon to find fundamental limits on signal processing operations such as compressing data and on reliably storing and...
, Sanov's theorem gives a bound on the probability of observing an atypical
Typical set
In information theory, the typical set is a set of sequences whose probability is close to two raised to the negative power of the entropy of their source distribution. That this set has total probability close to one is a consequence of the asymptotic equipartition property which is a kind of law...
sequence of samples from a given probability distribution
Probability distribution
In probability theory, a probability mass, probability density, or probability distribution is a function that describes the probability of a random variable taking certain values....
.
Let A be a set of probability distributions over an alphabet X, and let q be an arbitrary distribution over X (where q may or may not be in A). Suppose we draw n i.i.d.
Independent and identically distributed random variables
In probability theory and statistics, a sequence or other collection of random variables is independent and identically distributed if each random variable has the same probability distribution as the others and all are mutually independent....
samples from q, represented by the vector
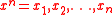 . Then
. Then ,
,where
-
 is shorthand for
is shorthand for 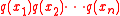 and
and  is shorthand for
is shorthand for  ,
, -
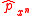 is the empirical distributionEmpirical distribution functionIn statistics, the empirical distribution function, or empirical cdf, is the cumulative distribution function associated with the empirical measure of the sample. This cdf is a step function that jumps up by 1/n at each of the n data points. The empirical distribution function estimates the true...
is the empirical distributionEmpirical distribution functionIn statistics, the empirical distribution function, or empirical cdf, is the cumulative distribution function associated with the empirical measure of the sample. This cdf is a step function that jumps up by 1/n at each of the n data points. The empirical distribution function estimates the true...
of the sample , and
, and -
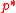 is the information projection of q onto A.
is the information projection of q onto A.
Furthermore, if A is a closed set
Closed set
In geometry, topology, and related branches of mathematics, a closed set is a set whose complement is an open set. In a topological space, a closed set can be defined as a set which contains all its limit points...
,


