
Random compact set
Encyclopedia
In mathematics
, a random compact set is essentially a compact set
-valued random variable
. Random compact sets are useful in the study of attractors for random dynamical system
s.
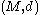 be a complete
be a complete
separable metric space
. Let denote the set of all compact subsets of
denote the set of all compact subsets of 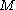 . The Hausdorff metric
. The Hausdorff metric 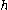 on
on 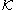 is defined by
is defined by

 is also а complete separable metric space. The corresponding open subsets generate a σ-algebra on
is also а complete separable metric space. The corresponding open subsets generate a σ-algebra on 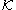 , the Borel sigma algebra
, the Borel sigma algebra 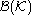 of
of 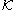 .
.
A random compact set is а measurable function
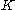 from а probability space
from а probability space
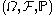 into
into 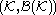 .
.
Put another way, a random compact set is a measurable function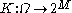 such that
such that  is almost surely
is almost surely
compact and

is a measurable function for every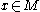 .
.
 for
for 
(The distribution of а random compact convex set is also given by the system of all inclusion probabilities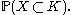 )
)
For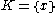 , the probability
, the probability 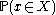 is obtained, which satisfies
is obtained, which satisfies
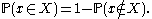
Thus the covering function is given by
is given by
 for
for 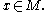
Of course,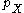 can also be interpreted as the mean of the indicator function
can also be interpreted as the mean of the indicator function 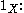

The covering function takes values between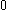 and
and 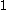 . The set
. The set 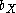 of all
of all 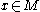 with
with 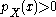 is called the support of
is called the support of  . The set
. The set 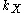 , of all
, of all 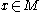 with
with 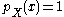 is called the kernel, the set of fixed points, or essential minimum
is called the kernel, the set of fixed points, or essential minimum 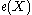 . If
. If 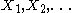 , is а sequence of i.i.d. random compact sets, then almost surely
, is а sequence of i.i.d. random compact sets, then almost surely

and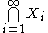 converges almost surely to
converges almost surely to 
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a random compact set is essentially a compact set
Compact space
In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
-valued random variable
Random variable
In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
. Random compact sets are useful in the study of attractors for random dynamical system
Random dynamical system
In mathematics, a random dynamical system is a measure-theoretic formulation of a dynamical system with an element of "randomness", such as the dynamics of solutions to a stochastic differential equation...
s.
Definition
Let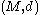 be a complete
be a completeComplete space
In mathematical analysis, a metric space M is called complete if every Cauchy sequence of points in M has a limit that is also in M or, alternatively, if every Cauchy sequence in M converges in M....
separable metric space
Metric space
In mathematics, a metric space is a set where a notion of distance between elements of the set is defined.The metric space which most closely corresponds to our intuitive understanding of space is the 3-dimensional Euclidean space...
. Let
 denote the set of all compact subsets of
denote the set of all compact subsets of 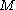 . The Hausdorff metric
. The Hausdorff metric 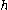 on
on 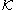 is defined by
is defined by
 is also а complete separable metric space. The corresponding open subsets generate a σ-algebra on
is also а complete separable metric space. The corresponding open subsets generate a σ-algebra on 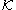 , the Borel sigma algebra
, the Borel sigma algebra 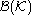 of
of 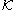 .
.A random compact set is а measurable function
Measurable function
In mathematics, particularly in measure theory, measurable functions are structure-preserving functions between measurable spaces; as such, they form a natural context for the theory of integration...
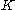 from а probability space
from а probability spaceProbability space
In probability theory, a probability space or a probability triple is a mathematical construct that models a real-world process consisting of states that occur randomly. A probability space is constructed with a specific kind of situation or experiment in mind...
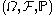 into
into 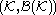 .
.Put another way, a random compact set is a measurable function
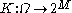 such that
such that  is almost surely
is almost surelyAlmost surely
In probability theory, one says that an event happens almost surely if it happens with probability one. The concept is analogous to the concept of "almost everywhere" in measure theory...
compact and

is a measurable function for every
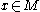 .
.Discussion
Random compact sets in this sense are also random closed sets as in Matheron (1975). Consequently their distribution is given by the probabilities for
for 
(The distribution of а random compact convex set is also given by the system of all inclusion probabilities
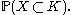 )
)For
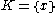 , the probability
, the probability 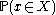 is obtained, which satisfies
is obtained, which satisfies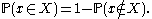
Thus the covering function
 is given by
is given by for
for 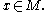
Of course,
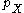 can also be interpreted as the mean of the indicator function
can also be interpreted as the mean of the indicator function 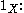

The covering function takes values between
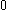 and
and 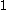 . The set
. The set 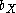 of all
of all 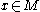 with
with 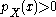 is called the support of
is called the support of  . The set
. The set 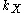 , of all
, of all 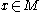 with
with 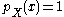 is called the kernel, the set of fixed points, or essential minimum
is called the kernel, the set of fixed points, or essential minimum 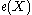 . If
. If 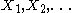 , is а sequence of i.i.d. random compact sets, then almost surely
, is а sequence of i.i.d. random compact sets, then almost surely
and
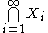 converges almost surely to
converges almost surely to 

