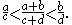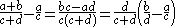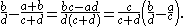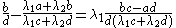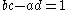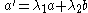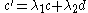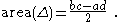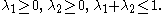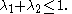Mediant (mathematics)
Encyclopedia
In mathematics
, the mediant (sometimes called freshman sum) of two fractions

is
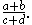
that is to say, the numerator and denominator of the mediant are the sums of the numerators and denominators of the given fractions, respectively.
In general, this is an operation on fractions rather than on rational numbers. That is to say, for two rational numbers q1, q2, the value of the mediant depends on how the rational numbers are expressed using integer pairs.
A way around this, where required, is to specify that both rationals are to be represented as fractions in their lowest terms
(with c > 0, d > 0). With such a restriction, mediant becomes a well-defined binary operation on rationals.
The Stern-Brocot tree
provides an enumeration of all positive rational numbers, in lowest terms, obtained purely by iterative computation of the mediant according to a simple algorithm.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the mediant (sometimes called freshman sum) of two fractions

is
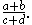
that is to say, the numerator and denominator of the mediant are the sums of the numerators and denominators of the given fractions, respectively.
In general, this is an operation on fractions rather than on rational numbers. That is to say, for two rational numbers q1, q2, the value of the mediant depends on how the rational numbers are expressed using integer pairs.
A way around this, where required, is to specify that both rationals are to be represented as fractions in their lowest terms
(with c > 0, d > 0). With such a restriction, mediant becomes a well-defined binary operation on rationals.
The Stern-Brocot tree
Stern-Brocot tree
In number theory, the Stern–Brocot tree is an infinite complete binary tree in which the vertices correspond precisely to the positive rational numbers, whose values are ordered from left to right as in a search tree....
provides an enumeration of all positive rational numbers, in lowest terms, obtained purely by iterative computation of the mediant according to a simple algorithm.
Properties
- The mediant inequality: An important property (also explaining its name) of the mediant is that it lies strictly between the two fractions of which it is the mediant: If
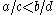 , then
, then
- This property follows from the two relations
-
- and
- Assume that the pair of fractions a/c and b/d satisfies the determinant relation
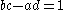 . Then the mediant has the property that it is the simplest fraction in the interval (a/c, b/d), in the sense of being the fraction with the smallest denominator. More precisely, if the fraction
. Then the mediant has the property that it is the simplest fraction in the interval (a/c, b/d), in the sense of being the fraction with the smallest denominator. More precisely, if the fraction 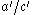 with positive denominator c' lies (strictly) between a/c and b/d, then its numerator resp. denominator can be written as
with positive denominator c' lies (strictly) between a/c and b/d, then its numerator resp. denominator can be written as 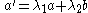 and
and 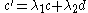 with two positive real (in fact rational) numbers
with two positive real (in fact rational) numbers 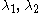 . To see why the
. To see why the 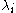 must be positive note that
must be positive note that
-
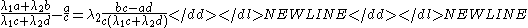
- must be positive. The determinant relation
- then implies that both
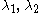 must be integers, solving the system of linear equations
must be integers, solving the system of linear equations
- for
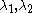 . Therefore
. Therefore 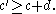
- The converse is also true: assume that the pair of reduced fractions a/c < b/d has the property that the reduced fraction with smallest denominator lying in the interval (a/c, b/d) is equal to the mediant of the two fractions. Then the determinant relation bc − ad = 1 holds. This fact may be deduced e.g. with the help of Pick's theoremPick's theoremGiven a simple polygon constructed on a grid of equal-distanced points such that all the polygon's vertices are grid points, Pick's theorem provides a simple formula for calculating the area A of this polygon in terms of the number i of lattice points in the interior located in the polygon and the...
which expresses the area of a plane triangle whose vertices have integer coordinates in terms of the number vinterior of lattice points (strictly) inside the triangle and the number vboundary of lattice points on the boundary of the triangle. Consider the triangle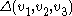 with the three vertices v1 = (0, 0), v2 = (a, c), v3 = (b, d). Its area is equal to
with the three vertices v1 = (0, 0), v2 = (a, c), v3 = (b, d). Its area is equal to
-
- A point
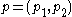 inside the triangle can be parametrized as
inside the triangle can be parametrized as
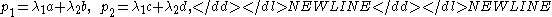
- where
- The Pick formula
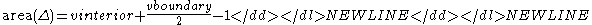
- now implies that there must be a lattice point q = (q1, q2) lying inside the triangle different from the three vertices if bc −&nsbp;ad >1 (then the area of the triangle is
 ). The corresponding fraction q1/q2 lies (strictly) between the given (by assumption reduced) fractions and has denominator
). The corresponding fraction q1/q2 lies (strictly) between the given (by assumption reduced) fractions and has denominator
-
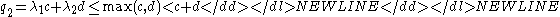
- as
- Relatedly, if p/q and r/s are reduced fractions on the unit interval such that |ps − rq| = 1 (so that they are adjacent elements of a row of the Farey sequenceFarey sequenceIn mathematics, the Farey sequence of order n is the sequence of completely reduced fractions between 0 and 1 which, when in lowest terms, have denominators less than or equal to n, arranged in order of increasing size....
) then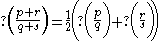
- where ? is Minkowski's question mark functionMinkowski's question mark functionIn mathematics, the Minkowski question mark function, sometimes called the slippery devil's staircase and denoted by ?, is a function possessing various unusual fractal properties, defined by Hermann Minkowski in 1904...
.
- In fact, mediants commonly occur in the study of continued fractionContinued fractionIn mathematics, a continued fraction is an expression obtained through an iterative process of representing a number as the sum of its integer part and the reciprocal of another number, then writing this other number as the sum of its integer part and another reciprocal, and so on...
s and in particular, Farey fractions. The nth Farey sequenceFarey sequenceIn mathematics, the Farey sequence of order n is the sequence of completely reduced fractions between 0 and 1 which, when in lowest terms, have denominators less than or equal to n, arranged in order of increasing size....
Fn is defined as the (ordered with respect to magnitude) sequence of reduced fractions a/b (with coprimeCoprimeIn number theory, a branch of mathematics, two integers a and b are said to be coprime or relatively prime if the only positive integer that evenly divides both of them is 1. This is the same thing as their greatest common divisor being 1...
a, b) such that b ≤ n. If two fractions a/c < b/d are adjacent (neighbouring) fractions in a segment of Fn then the determinant relation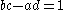 mentioned above is generally valid and therefore the mediant is the simplest fraction in the interval (a/c, b/d), in the sense of being the fraction with the smallest denominator. Thus the mediant will then (first) appear in the (c + d)th Farey sequence and is the "next" fraction which is inserted in any Farey sequence between a/c and b/d. This gives the rule how the Farey sequences Fn are successively built up with increasing n.
mentioned above is generally valid and therefore the mediant is the simplest fraction in the interval (a/c, b/d), in the sense of being the fraction with the smallest denominator. Thus the mediant will then (first) appear in the (c + d)th Farey sequence and is the "next" fraction which is inserted in any Farey sequence between a/c and b/d. This gives the rule how the Farey sequences Fn are successively built up with increasing n.
External links
- Mediant Fractions at cut-the-knotCut-the-knotCut-the-knot is a free, advertisement-funded educational website maintained by Alexander Bogomolny and devoted to popular exposition of many topics in mathematics. The site has won more than 20 awards from scientific and educational publications, including a Scientific American Web Award in 2003,...
- MATHPATH: Cube roots via Mediants
- MATHPAGES, Kevin Brown: Generalized Mediant
- as
- now implies that there must be a lattice point q = (q1, q2) lying inside the triangle different from the three vertices if bc −&nsbp;ad >1 (then the area of the triangle is
- where
-