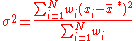Mean square weighted deviation
Encyclopedia
Mean square weighted deviation is used extensively in geochronology
, the science of obtaining information about the time of formation of, for example, rocks, minerals, bones, corals, or charcoal, or the time at which particular processes took place in a rock mass, for example recrystallization and grain growth, or alteration associated with the emplacement of metalliferous ore deposits..
Often the geochronologist will determine a series of age measurements on a single sample, with the measured value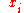
having a weighting 
and an associated error 
for each age determination. As regards weighting, one can either weight all of the measured ages equally, or weight them by the proportion of the sample that they represent. For example, if two thirds of the sample was used for the first measurement and one third for the second and final measurement then one might weight the first measurement twice that of the second.
The arithmetic mean of the age determinations is: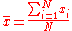
but this value can be misleading unless each determination of the age is of equal significance.
When each measured value can be assumed to have the same weighting, or significance, the biased and unbiased (or "population" and "sample", respectively) estimators of the variance are computed as follows:
The standard deviation is the square root of the variance.
When individual determinations of an age are not of equal significance it is better to use a weighted mean to obtain an 'average' age, as follows: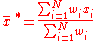
The biased weighted estimator of variance can be shown to be:
which can be computed on the fly as
Geochronology
Geochronology is the science of determining the age of rocks, fossils, and sediments, within a certain degree of uncertainty inherent to the method used. A variety of dating methods are used by geologists to achieve this, and schemes of classification and terminology have been proposed...
, the science of obtaining information about the time of formation of, for example, rocks, minerals, bones, corals, or charcoal, or the time at which particular processes took place in a rock mass, for example recrystallization and grain growth, or alteration associated with the emplacement of metalliferous ore deposits..
Often the geochronologist will determine a series of age measurements on a single sample, with the measured value
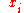having a weighting and an associated error for each age determination. As regards weighting, one can either weight all of the measured ages equally, or weight them by the proportion of the sample that they represent. For example, if two thirds of the sample was used for the first measurement and one third for the second and final measurement then one might weight the first measurement twice that of the second.The arithmetic mean of the age determinations is:
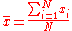
but this value can be misleading unless each determination of the age is of equal significance.
When each measured value can be assumed to have the same weighting, or significance, the biased and unbiased (or "population" and "sample", respectively) estimators of the variance are computed as follows:
The standard deviation is the square root of the variance.
When individual determinations of an age are not of equal significance it is better to use a weighted mean to obtain an 'average' age, as follows:
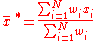
The biased weighted estimator of variance can be shown to be:
which can be computed on the fly as
-
The unbiased weighted estimator of the sample variance can be computed as follows: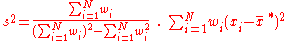
Again the corresponding standard deviation is the square root of the variance.
The unbiased weighted estimator of the sample variance can also be computed on the fly as follows: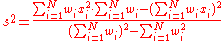
The unweighted mean square of the weighted deviations (unweighted MSWD) can then be computed, as follows:
- MSWD
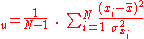
By analogy the weighted mean square of the weighted deviations (weighted MSWD) can be computed, as follows:
- MSWD
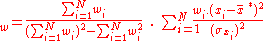
- MSWD